WRITING EXPONENTIAL FUNCTION FOR THE GIVEN TRANSFORMATION

Starting with the graph of f(x) = 4^x, find a formula for the function that result from

Problem 1 :

Shifting f(x) 4 units upwards

Solution :

f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Shifting means translation. We have to move the graph vertically 4 units up. So, the value of k can be fixed as 4.

f(x) = 4^x + 4

Problem 2 :

Shifting f(x) 3 units downwards

Solution :

f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Shifting means translation. We have to move the graph vertically 3 units down. So, the value of k can be fixed as -3.

f(x) = 4^x - 3

Problem 3 :

Shifting f(x) 2 units left

Solution :

f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Shifting means translation. We have to move the graph horizontally 2 units left. So, the value of h can be fixed as -2.

f(x) = 4^x-(-2)

= 4^{x + 2}

So, the required function is f(x) = 4^{x + 2}

Problem 4 :

Shifting f(x) 5 units right

Solution :

Parent function is f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Shifting means translation. We have to move the graph horizontally 5 units right. So, the value of h can be fixed as 5.

f(x) = 4^{x - 5}

= 4^{x - 5}

So, the required function is f(x) = 4^{x - 5}

Problem 5 :

Reflection f(x) about x-axis

Solution :

Parent function is f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Reflection about x-axis, put y = -y

f(x) = -4^x

So, the required function is f(x) = -4^x.

Problem 6 :

Reflection f(x) about y-axis

Solution :

Parent function is f(x) = 4^x

Comparing the given function with y = ab^x-h + k

Reflection about y-axis, put x = -x

f(x) = 4^-x

So, the required function is f(x) = 4^x.

Write the equation for the function that results from each transformation applied to the base function

y = 7^x

Problem 7 :

reflect in the x-axis (vertical reflection)

Solution :

Parent function is y = 7^x

Vertical reflection, then change x as -x.

Applying the changes y = 7^-x

By graphing it,

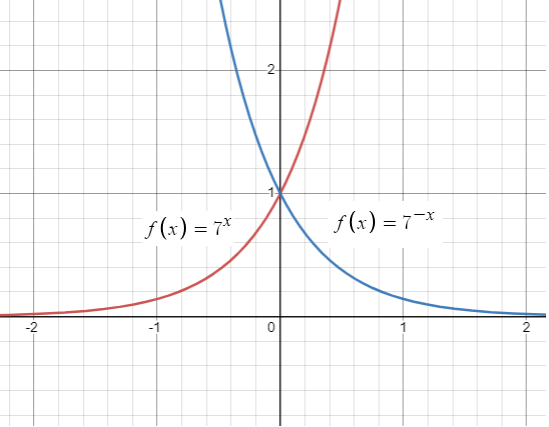
Problem 8 :

stretch vertically by a factor of 3

Solution :

Parent function is y = 7^x

Comparing the given function with y = ab^x-h + k

Vertical stretch, then a = 3

Applying the changes y = 3(7^x)

By graphing it,

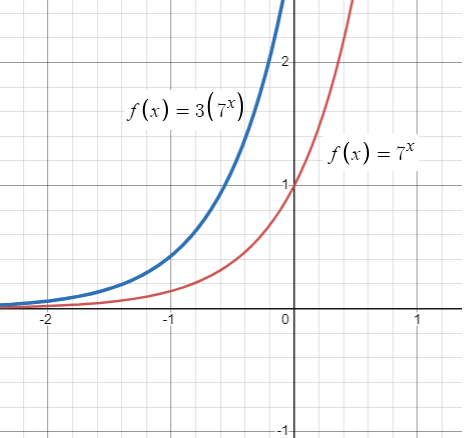
Problem 9 :

Stretch horizontally by a factor of 2.4

Solution :

Parent function is y = 7^x

Comparing the given function with y = ab^x-h + k

Vertical stretch, then a = 2.4

Applying the changes y = 2.4(7^x)

By graphing it,

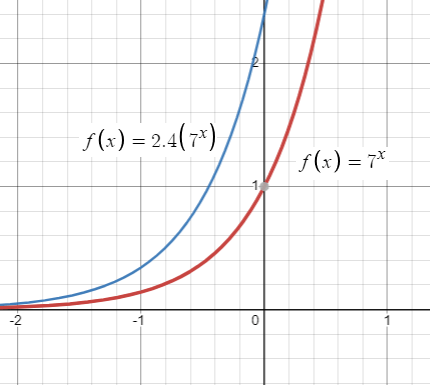
Problem 10 :

reflect in the y-axis and stretch vertically by 7

Solution :

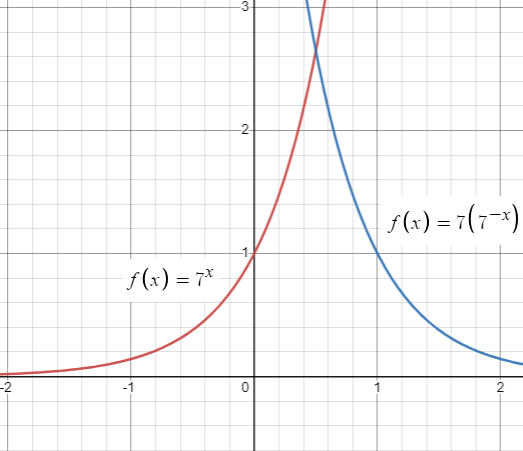
Parent function is y = 7^x

Comparing the given function with y = ab^x-h + k

Reflect about y-axis, then put x = -x

Vertical stretch, then a = 7

Applying the changes y = 7(7^-x)

By graphing it,