TRIANGLES AND SPECIAL RIGHT TRIANGLES VOCABULARY WORDS

Right triangle :

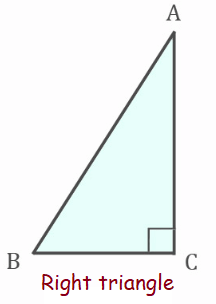
A right angled triangle is a triangle with one of the angles as 90 degrees. A 90-degree angle is called a right angle.

Definition of Leg :

A leg of a triangle is one of its sides. For a right triangle, the term "leg" generally refers to a side other than the one opposite the right angle (which is termed the hypotenuse).

AB is leg.

Hypotenuse :

The hypotenuse of a right triangle is always the side opposite the right angle. It is the longest side in a right triangle

AB is hypotenuse of the right triangle.

Pythagoras theorem :

Pythagoras theorem states that “In a right-angled triangle, the square of the hypotenuse side is equal to the sum of squares of the other two sides“

AB² = AC² + BC²

Pythagorean triples :

Pythagorean triples are

a²+b² = c²

where a, b and c are the three positive integers. These triples are represented as (a,b,c). Here, a is the perpendicular, b is the base and c is the hypotenuse of the right-angled triangle.

For example (9, 12 and 15) are Pythagorean triples.

15² = 9² + 12²

225 = 81 + 144

225 = 225

Special Right Triangles

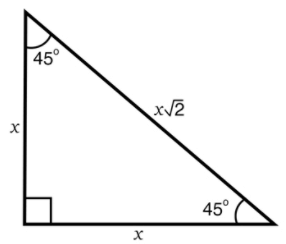
45-45-90 triangle :

The 45-45-90 triangle rule states that the three sides of the triangle are in the ratio 1:1:√2. So, if the measure of the two congruent sides of such a triangle is x each, then the three sides will be x, x and √2x.

This rule can be proved by applying the Pythagorean theorem.

30-60-90 Theorem:

If a triangle has angle measures 30∘, 60^∘ and 90^∘, then the sides are in the ratio x : √3x : 2x .

The shorter leg is always be x

The side opposite to 90 degree is 2x

The side opposite to 60 degree is √3x

Angle of elevation and depression

Trigonometric ratios :

The six trigonometric ratios are sine (sin), cosine (cos), tangent (tan), cotangent (cot), cosecant (cosec), and secant (sec).

Definition of sine :

The value of sin θ can be determined by dividing opposite side by hypotenuse.

sinθ = Opposite side / Hypotenuse

Definition of cosine :

The value of cos θ can be determined by dividing adjacent  side by hypotenuse.

cos θ = Adjacent side / Hypotenuse

Definition of tangent :

The value of tan θ can be determined by dividing opposite side by adjacent  side.

tan θ = Opposite side / Adjacent side

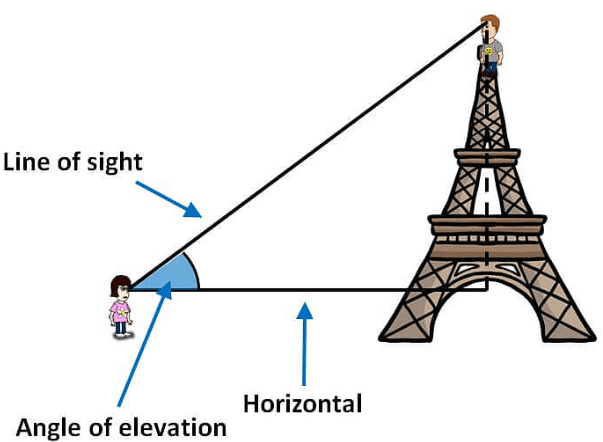
Angle of elevation :

The angle of elevation is formed when it is between the line of sight and the horizontal line. If the line of sight is above to the horizontal line, the angle formed is known as the angle of elevation. But in the angle of depression, the line of sight is downwards to the horizontal line. The figure that represents the angle of elevation is given below:

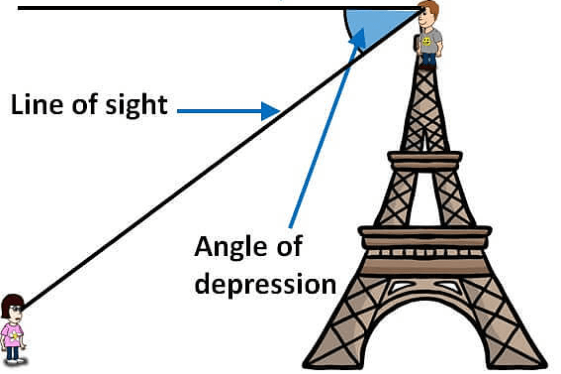
Angle of depression:

The angle of depression is the angle between the horizontal line and the observation of the object from the horizontal line. It is basically used to get the distance of the two objects where the angles and an object’s distance from the ground are known to us. Its an angle that is formed with the horizontal line if the line of sight is downward from the horizontal line.

Law of Sines :

The Law of Sines is the relationship between the sides and angles of non-right (oblique) triangles .  Simply, it states that the ratio of the length of a side of a triangle to the sine of the angle opposite that side is the same for all sides and angles in a given triangle.

Laws of Cosine :

The Cosine Rule can be used in any triangle where you are trying to relate all three sides to one angle.

a² = b² + c² - 2bc cos A

b² = a² + c² - 2ac cos B

c² = a² + b² - 2ab cos C