ANGLE BISECTOR THEOREM PROOF

Theorem

The internal (external) bisector of an angle of a triangle divides the opposite side internally (externally) in the ratio of the corresponding sides containing the angle.

Case (i) (Internally) :

Given : In ΔABC, AD is the internal bisector of ∠BAC which meets BC at D.

To prove : BD/DC = AB/AC.

Construction : Draw CE ∥ DA to meet BA produced at E.

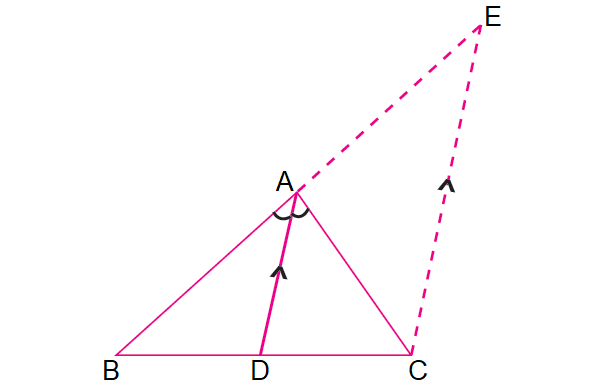
Proof (Internally)

Because CE ∥ DA and AC is the transversal, we have

∠DAC = ∠ACE (alternate angles) -----(1)

and

∠BAD = ∠AEC (corresponding angles) -----(2)

Because AD is the angle bisector of ∠A,

∠BAD = ∠DAC -----(3)

From (1), (2) and (3), we have

∠ACE = ∠AEC

Thus in ΔACE, we have

AE = AC

(Sides opposite to equal angles are equal)

Now, in ΔBCE we have, CE ∥ DA.

By Thales Theorem,

BD/DC = BA/AE

Because AE = AC,

BD/DC = AB/AC

Hence the theorem.

Case (i) (Externally) :

Given : In ΔABC, AD is the external bisector of ∠BAC and intersects BC produced at D.

To prove : BD/DC = AB/AC.

Construction : Draw CE ∥ DA meeting AB at E.

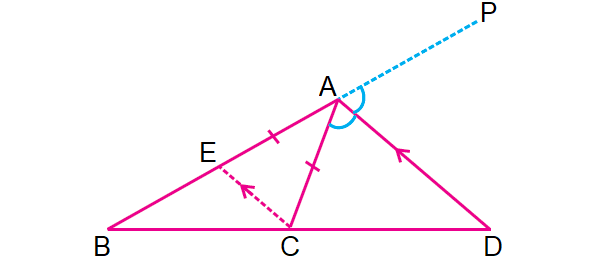
Proof (Externally)

Because CE ∥ DA and AC is a transversal, we have

∠ECA = ∠CAD (alternate angles) ----(1)

Also, CE ∥ DA and BP is a transversal, we have

∠CEA = ∠DAP (corresponding angles) ----(2)

But AD is the bisector of ∠CAP,

∠CAD = ∠DAP ----(3)

From (1), (2) and (3), we have

∠CEA = ∠ECA

(Sides opposite to equal angles are equal)

In ΔBDA, we have EC ∥ AD.

By Thales Theorem,

BD/DC = BA/AE

Because AE = AC,

BD/DC = BA/AC

Hence the theorem.

Solved Problems

Problem 1 :

In the ΔABC shown below, find the length of AD.

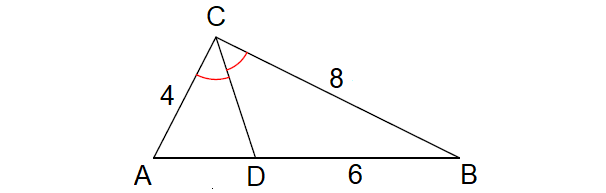
Solution :

Since CD is the angle bisector of ∠C, by Angle Bisector Theorem,

AD/DB = CA/CB

Substitute.

AD/6 = 4/8

AD/6 = 1/2

Multiply both sides by 6.

AD = 3

Problem 2 :

In the ΔABC shown below, find the length of CD.

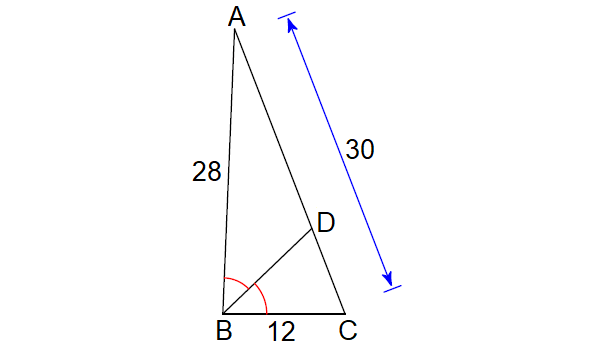
Solution :

Let x be the length of CD.

Then the length of DA = 30 - x.

Since BD is the angle bisector of ∠B, by Angle Bisector Theorem,

CD/DA = BC/BA

Substitute.

x/(30 - x) = 12/28

x/(30 - x) = 3/7

7x = 3(30 - x)

7x = 90 - 3x

Add 3x to each side.

10x = 90

Divide each side by 10.

x = 9

CD = 9

Problem 3 :

Solve for x.

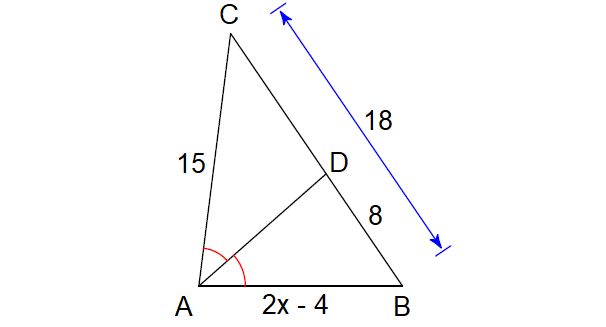
Solution :

Find the length of DC :

DC = BC - DC

= 18 - 8

= 10

Since AD is the angle bisector of ∠A, by Angle Bisector Theorem,

BD/DC = AB/AC

Substitute.

8/10 = (2x - 4)/15

4/5 = (2x - 4)/15

15(4) = 5(2x - 4)

60 = 10x - 20

Add 20 to each side of the equation.

80 = 10x

Divide each side by 10.

8 = x

Problem 4 :

Solve for x.

Solution :

Find the length of DA :

DA = CA - Cd

= (x + 3) - 4

= x + 3 - 4

= x - 1

Since BD is the angle bisector of ∠B, by Angle Bisector Theorem,

CD/DA = BC/BA

Substitute.

4/(x - 1) = 6/9

4/(x - 1) = 2/3

3(4) = 2(x - 1)

12 = 2x - 2

Add 2 to each side.

14 = 2x

Divide each side by 2.

7 = x

Problem 5 :

In the given figure, AD is the bisector of <BAC, if AB = 10 cm, AC = 6 cm and BC = 12 cm. Find BD and DC.

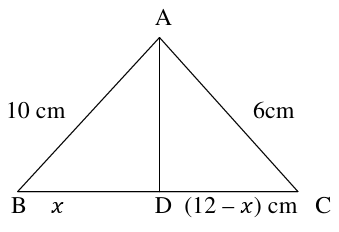
Solution :

BD = x, DC = 12 - x, AB = 10 cm and AC = 6 cm

BD/DC = AB/AC

x/(12 - x) = 10/6

6x = 10(12 - x)

6x = 120 - 10x

6x + 10x = 120

16x = 120

x = 120/16

x = 7.5

DC = 12 - 7.5

= 5.5 cm

Problem 6 :

The figure shows a triangle with one of its angle bisectors.

Find x if m∠2 = 4 x + 5 and m∠1 = 5 x − 2.

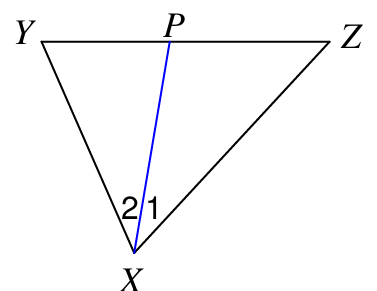
Solution :

m∠1 = m∠2

5 x − 2 = 4 x + 5

5x - 4x = 5 + 2

x = 7

So, the value of x is 7.

Problem 7 :

Find x if m∠2 = 1 + 28 x and m∠XVW = 59 x − 1.

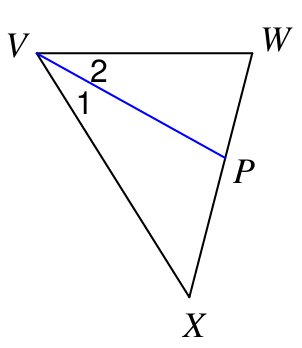
Solution :

m∠1 = m∠2

59x - 1 = 1 + 28x

59x - 28x = 1 + 1

31x = 2

x = 2/31

x = 0.06

Problem 8 :

In triangle ABC, the internal bisector AD of ∠A meets the side BC at D. If BD = 2.5 cm, AB = 5 cm and AC = 4.2 cm, then find DC.

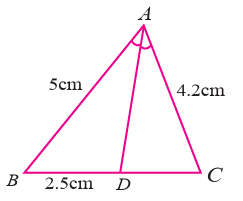
Solution :

Using angle bisector theorem,

BD/DC = AB/AC

Let DC = x

2.5/x = 5/4.2

Doing cross multiplication, we get

2.5(4.2) = 5x

x = 2.5(4.2) / 5

x = 2.1

So, the measure of DC is 2.1 cm.