WRITING INTERVAL NOTATION GIVEN A NUMBER LINE

Interval Notation :

Interval notation is a method to represent an interval on a number line. In other words, it is a way of writing subsets of the real number line.

An interval comprises the numbers lying between two specific given numbers.

Use interval notation to describe :

Problem 1 :

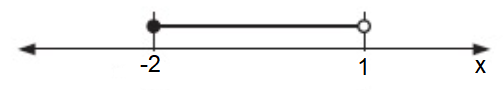
Solution :

By observing the number line, the possible values of x are

-2 ≤ x < 1

By expressing it as interval notation, we get

[-2, 1)

Problem 2 :

Solution :

By observing the number line, the possible values of x are

x ≤ 0 or x > 1

By expressing it as interval notation, we get

(-∞, 0] and (1, ∞)

Problem 3 :

Solution :

By observing the number line, the possible values of x are

2 ≤ x ≤ 4

By expressing it as interval notation, we get

[2, 4]

Problem 4 :

Solution :

By observing the number line, the possible values of x are

-2 < x < 1

By expressing it as interval notation, we get

(-2, 1)

Problem 5 :

Solution :

By observing the number line, the possible values of x are

x < -1 (or) x > 1

By expressing it as interval notation, we get

(-∞, -1) and (1, ∞)

Problem 6 :

Solution :

By observing the number line, the possible values of x are

0 < x ≤ 4

By expressing it as interval notation, we get

(0, 4]

Problem 7 :

Solution :

By observing the number line, the possible values of x are

x < 0 (or) x ≥ 3

By expressing it as interval notation, we get

(-∞, 0) and [3, ∞)

Problem 8 :

Solution :

By observing the number line, the possible values of x are

x ≤ -2 (or) x ≥ 2

By expressing it as interval notation, we get

(-∞, -2] and [2, ∞)

Problem 9 :

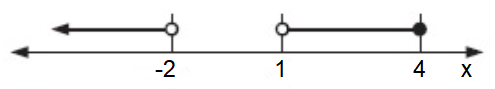
Solution :

By observing the number line, the possible values of x are

x < -2 (or) 1 < x ≤ 4

By expressing it as interval notation, we get

(-∞, -2) and (1, 4]

Problem 10 :

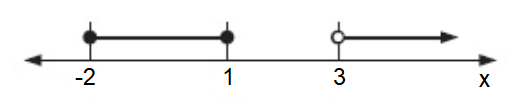
Solution :

By observing the number line, the possible values of x are

-2 ≤ x ≤ 1 (or) x > 3

By expressing it as interval notation, we get

[-2, -1] and (3, ∞)