WRITE THE EQUATION FROM THE GRAPH OF ABSOLUTE VALUE FUNCTION

Write the equation of the graph. Then give its range as an inequality.

Problem 1 :

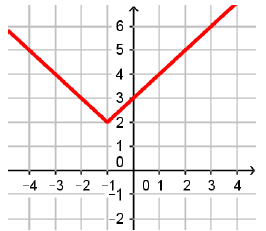
Solution :

By observing the graph, vertex is at (-1, 2).

y = a |x - h| + k

y = a |x - (-1)| + 2

y = a |x +1| + 2 ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (-2, 3). Since the curve opens up, it will have positive slope.

Applying x = -2 and y = 3 in (1)

3 = a |-2 +1| + 2

3 = a |-1| + 2

3 = a + 2

a = 3 - 2

a = 1

So, the required equation is 

y = 1 |x +1| + 2

Range is 2 ≤ y ≤ ∞

Problem 2 :

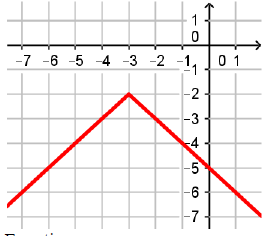
Solution :

By observing the graph, vertex is at (-3, -2).

y = a |x - h| + k

y = a |x - (-3)| - 2

y = a |x + 3| - 2 ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (-4, -3).Since the curve opens down, it will have negative slope.

Applying x = -3 and y = -2 in (1)

-3 = a |-4 +3| - 2

-3 = a |-1| - 2

-3 = a - 2

a = -3 + 2

a = -1

So, the required equation is 

y = -1 |x + 3| - 2

Range is -2 ≤ y ≤ -∞

Problem 3 :

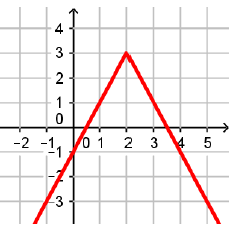
Solution :

By observing the graph, vertex is at (2, 3).

y = a |x - h| + k

y = a |x - 2| + 3

y = a |x - 2| + 3 ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (1, 1).Since the curve opens down, it will have negative slope.

Applying x = 1 and y = 1 in (1)

1 = a |1 - 2| + 3

1 = a |-1| + 3

1 = a + 3

a = 1 - 3

a = -2

So, the required equation is 

y = -2 |x - 2| + 3

Range is 3 ≤ y ≤ -∞

Problem 4 :

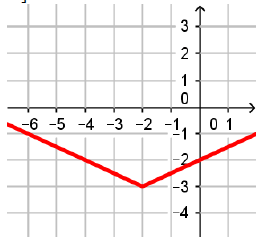
Solution :

By observing the graph, vertex is at (-2, -3).

y = a |x - h| + k

y = a |x - (-2)| - 3

y = a |x + 2| - 3 ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (-4, -2).Since the curve opens down, it will have negative slope.

Applying x = -4 and y = -2 in (1)

-2 = a |-4 + 2| - 3

-2 = a |-2| - 3

-2 = 2a - 3

2a = -2 + 3

2a = 1

a = 1/2

So, the required equation is 

y = (1/2) |x + 2| - 3

Range is -3 ≤ y ≤ ∞

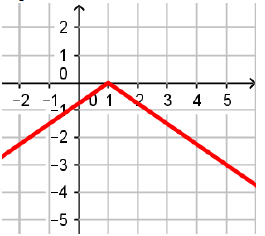
Problem 5 :

Solution :

By observing the graph, vertex is at (1, 0).

y = a |x - h| + k

y = a |x - 1| + 0

y = a |x - 1| ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (5, -3).Since the curve opens down, it will have negative slope.

Applying x = 5 and y = -3 in (1)

-3 = a |5 - 1|

-3 = a |4|

4a = -3

a = -3/4

So, the required equation is 

y = (-3/4) |x - 1|

Range is 1 ≤ y ≤ -∞

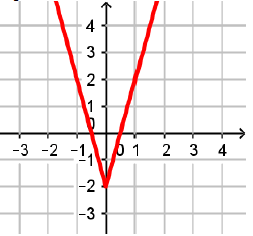
Problem 6 :

Solution :

By observing the graph, vertex is at (-2, 0).

y = a |x - h| + k

y = a |x - (-2)| + 0

y = a |x + 2| ---(1)

Tracing one more point from the graph, the absolute value function passes through the point (1, 2).Since the curve opens down, it will have negative slope.

Applying x = 1 and y = 2 in (1)

2 = a |1 + 2|

2 = a |3|

3a = 2

a = 2/3

So, the required equation is 

y = (2/3) |x + 2|

Range is -2 ≤ y ≤ ∞