WORKSHEET ON ADDING AND SUBTRACTING POLYNOMIALS
Simplify the following.
Problem 1 :
(5x2 – 2x + 7) + (x3 – 5x2 – x + 3)
Problem 2 :
(x3 – x2 + x + 1) + (x3 + x2 - x - 1)
Problem 3 :
(2x4 + x2 - 1) + (x4 – x3 + x + 1)
Problem 4 :
(x4 - 2x3 + 3x2 – 4x + 5) + (5x3 – x2 + 7x - 3)
Problem 5 :
(2x3 + 3x2 – 5x + 1) + (6 – 2x + 3x2 – x3)
Problem 6 :
(4x2 – x + 3) – (3x2 + x + 1)
Problem 7 :
(2x3 + x2 + 5x - 7) – (x3 – 2x2 + 5x + 4)
Problem 8 :
(9x4 + x3 + 2x - 3) – (5x4 + 7x2 – 2x + 3)
Problem 9 :
(5x2 + 7x + 1) + (2x2 + x - 3) + (x2 – 10x + 7)
Problem 10 :
(x3 – x + 3) + (x2 – 3x + 4) + (2x3 – x2 + 5)
Answer Key
1) x3 – 3x + 10
2) 2x3
3) 3x4 – x3 + x2 + x
4) x4 + 3x3 + 2x2 + 3x + 2
5) x3 + 6x2 – 7x + 7
6) x2 – 2x + 2
7) x3 + 3x2 – 11
8) 4x4 + x3 – 7x2 + 4x – 6
9) 8x2 - 2x + 8
10) 3x3 – 4x + 11
Express the area of the figure as a polynomial in descending powers of the variable x.
Problem 1 :
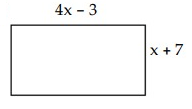
Problem 2 :
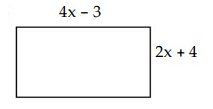
Problem 3 :
The area of a rectangle is 20m2 – 13m – 15. Find the length if the width is 4m – 5.
Problem 4 :
A rectangular patio has an area of 2m3 + 12m2 + 6m – 40. Find the length if the width is 2m + 8.
Find simplified expressions for the perimeter and area of the given figure.
Problem 5 :
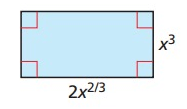
Problem 6 :
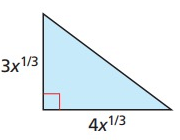
Problem 7 :
The area of a rectangular window is (2x2 – 7x – 15). Both the length and the width are polynomials with integer coefficients. Which of the following could represent the length of the window?
Problem 8 :
A triangle is inscribed in a square, as shown. Write and simplify a function r in terms of x that represents the area of the shaded region.
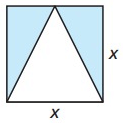
Answer Key
1) 4x2 + 25x - 21
2) 8x2 + 10x - 12
3) 5m + 3 = l
4) m2 + 2m - 5.
5) 2x(11/3)
6) 12x1/3
7) the length of the window is 2x + 3.
8) 1/2 x2
More Practice Questions
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling