WORD PROBLEMS ON CONES AND PYRAMIDS

Find each part. All bases are regular.

Problem 1 :

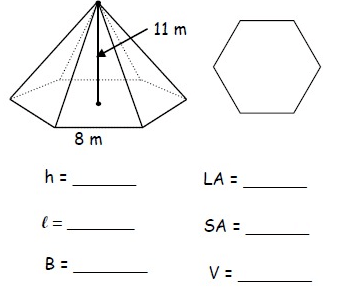
Solution :

Height (h):

Height h = 11 m

Base edge b = 8 m

Slant height (l):

Slant height l = √11² + (4√3)²

= √121 + 48

= √169

Slant height l = 13 m

Base area (B):

Perimeter = sides × base

= 6 × 8

P = 48

Base area = 1/2 × aP

= 1/2 × 4√3 × 48

Base area = 166.27 m²

Lateral area (LA):

Lateral area = 1/2 × Pl

LA = 1/2 × 48 × 13

= 24 × 13

LA = 312 m²

Surface area (SA):

Surface area = Base area + LA

SA = 166.27 + 312

SA = 478.27 m²

Volume (V):

Volume V = 1/3 × B × h

= 1/3 × 166.27 × 11

V = 609.65 m³

Problem 2 :

Solution :

Height (h) :

Height h = 15 cm

Base edge b = 16 cm

Slant height (l) :

Slant height = √h² + (s/2)²

= √15² + 8²

= √225 + 64

= √289

Slant height (l) = 17 cm

Base area (B) :

Base area = a²

= 16 × 16

Base area = 256 cm²

Lateral area (LA) :

Lateral area = 1/2 × Pl

LA = 1/2 × 64 × 17

LA = 544 cm²

Surface area (SA) :

Surface area = Base area + LA

= 256 + 544

 SA = 800 cm²

Volume (V) :

Volume V = 1/3 × B × h

= 1/3 × 256 × 15

V = 1280 cm³

Problem 3 :

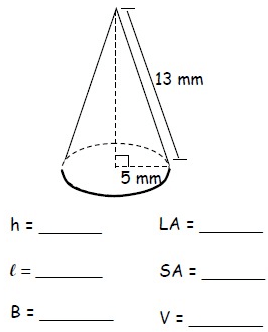
Solution :

Height (h):

Height = √l² - r²

= √13² - 5²

= √169 - 25

= √144

Height = 12 mm

Slant height (l):

Slant height = 13 mm

Base area (B):

Base area = πr²

= 3.14 × 5 × 5

B = 78.54 mm²

Lateral area (LA):

Lateral area = πrl

= 3.14 × 5 × 13

LA = 204.1 mm²

Surface area (SA):

Surface area = Base area + LA

= 78.54 + 204.1

SA = 282.64 mm²

Volume (V):

Volume V = 1/3 πr²h

= 1/3 × 3.14 × 5 × 5 × 12

V = 314 mm³

Problem 4 :

Jill made a popcorn container that is shaped like a cone. The diameter of the opening is 8 inches and the height is 9 inches. What is the volume of the popcorn container?

Solution :

Given, diameter = 8 inches

Radius = 8/2 = 4 inches

Height = 9 inches

Volume of popcorn container = 1/3 πr²h

= 1/3 × 22/7 × (4)² × 9

= 150.85 inches³

So, the volume of popcorn container is 150.85 inches³.

Problem 5 :

The lateral area of a cone is 48π in². The radius of the base is 12 in. Find the slant height.

Solution :

Given, lateral area of cone = 48π in²

Radius of the base = 12 in

Lateral area of cone = π × r × l

48π = π × r × l

48 = 12 × l

l = 48/12

l = 4 in

So, slant height of the cone is 4 in.

Problem 6 :

The volume of a square pyramid is 600 in³. The height of the pyramid is 8 in. what is the length of each base edge?

Solution :

Given, volume of square pyramid = 600 in³

Height of the pyramid = 8 in

Volume of square pyramid = 1/3 × a²h

600 = 1/3 × a² × 8

a² = 600 × 3/8

a² = 225

a = 15 in

So, length of each base edge is 15 in.

Problem 7 :

A square pyramid has a slant height of 25 m and a lateral area of 350 m². which is the closest to the volume?

a)  392 m³   b) 1176 m³   c) 404 m³   d) 1225 m³

Solution :

Given, lateral area = 350 m²

Slant height = 25 m

4 ∙ (a/2) ∙ s = 350

2a ∙ 25 = 350

50a = 350

a = 350/50

a = 7 m

The side length of the square base is 7 m.

The volume of a square pyramid = 1/3 ∙ a²h

h = √25² - (7/2)²

h = √2451/4

h = 24.75 m

V = (1/3) × 7² × 24.75

V = 404.25 m³

So, option (c) is correct.