VOLUME OF TRIANGULAR PRISM

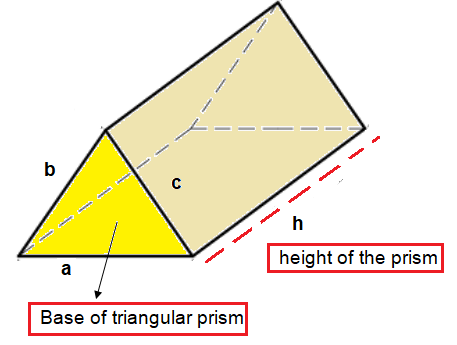
Volume of triangular prism = Base area x height

Problem 1 :

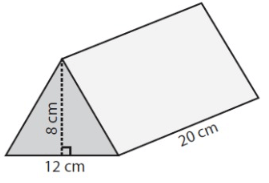
Find the volume of the triangular prism given below.

Solution :

Base area = (1/2) x base x height

= (1/2) x 12 x 8

= 48

Volume = baes area x height of the prism

= 48 x 20

V = 960 cm³

Problem 2 :

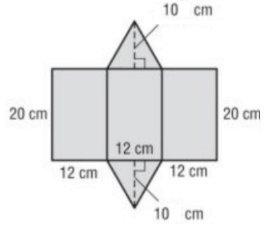
Find the 

(i) Total surface area

(ii) Volume

of the triangular prism given below.

Solution :

(ii) Total surface area :

Area of triangle = 2(1/2) × b × h

= 12 × 10

Area of triangle = 120 cm² --- > (1)

Area of rectangle = b × h

= 20 × 12

= 240 cm²  

Area of three rectangle = 3 × b × h

= 3 × 240

= 720 cm² --- > (2)

Add (1) & (2)

Total surface area = 120 + 720

= 840 cm²

So, surface area of triangular prism = 840 cm²

(ii)  Volume = Base area x height

= 120 x 20

= 2400 cm³

Problem 3 :

The height of a triangular prism is 6 cm and its base is an equilateral triangle of side 5 cm. Find the volume of the prism.

Solution :

Volume of triangular prism (V) = Base area x height

Base is in the shape of equilateral triangle,

Area of equilateral triangle = √3/4 × a²

So, volume of triangular prism = 64.95 cm³

Problem 4 :

A right prism stands on a base which is a right triangle with legs 3 cm and 4 cm. find the volume of the prism if its height is 9 cm.

Solution :

Given, side a = 3 cm and b = 4 cm

Using Pythagoras theorem,

Area of triangle = √s(s - a)(s - b)(s - c)

= √6 (6 - 3)(6 - 4)(6 - 5)

= √6(3)(2)(1)

= √36

= 6 cm²

Volume of right prism = Base area × height

= 6 × 9

= 54 cm³

So, volume of triangular prism = 54 cm³