VOLUME OF SQUARE PYRAMID

To calculate volume

• Find the base area
• Multiply it by height
• Multiply this by 1/3

For the answer use cubic units.

Find the volume of the square pyramids given below.

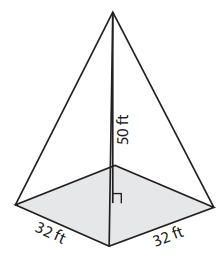
Problem 1 :

Solution :

Base is in the shape of square 

Base area = area of square

Area of square = (Side)²

= (32)²

Height = 50 ft

Volume of pyramid = (1/3) x (32)² x 50

= 17066.6 ft³

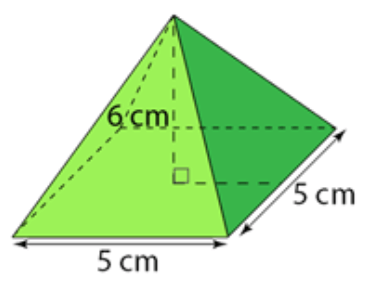
Problem 2 :

Solution :

Base area = area of square

height = 6 cm

Area of square = 5²

Volume = (1/3) x 5² x 6

= 50 cm³

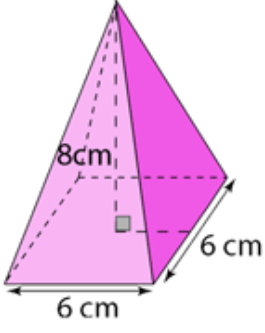
Problem 3 :

Solution :

Base area = area of square

height = 8 cm

Area of square = 6²

Volume = (1/3) x 6² x 8

= 96 cm³

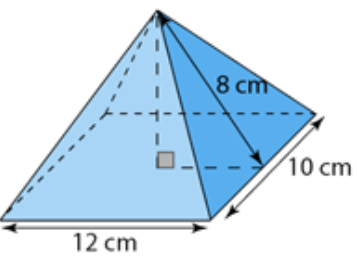
Problem 4 :

Solution :

Base area = area of square

Let height = h cm

Slant height = 8 cm

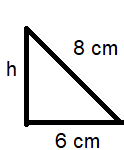Using Pythagorean theorem h2 + 62 = 82 h2 + 36 = 64 h2 = 64 - 36 h2 = 28 h = 5.29

Area of square = 12²

= 144

Volume = (1/3) x 144 x 5.29

= 253.92 cm³

Problem 4 :

Find the volume of the square pyramid with a height of 15 in and a slant height of 17 in. The square base measures 16 by 16 inches. 

Solution :

Base length of square = 16 inches

height = 15 in

slant height = 17 in

Volume of square pyramid = (1/3) x 16² x 15

= 1280 cubic inches

Problem 5 :

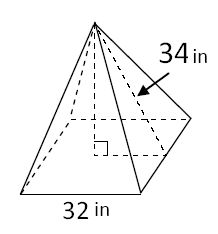
Solution :

Base length = 32 in

Slant height = 34 in

height = h 

h² + 16² = 34²

h² + 256 = 1156

h² = 1156 - 256

h² = 900

h = 30

Volume = (1/3) x 32² x 30

 = 1024 x 10

= 10240 cubic inches

Problem 6 :

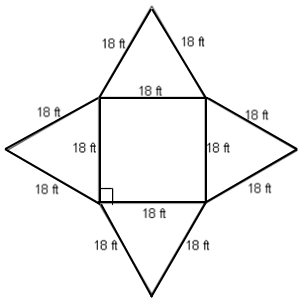
Solution :

When we fold this shape, we will get square base pyramid.

Volume of the square pyramid = (1/3) x base area x height

Base area = 18²

= 324 square feet

Considering the equilateral triangle.

h² + 9² = 18²

h² + 81 = 324

h² = 324 - 81

h² = 243

h = 15.58

Volume = (1/3) x 324 x 15.58

= 108 x 15.58

= 1682.64 cubic ft

Problem 7 :

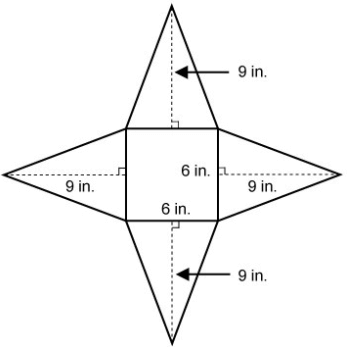
Solution :

Area of the base = 6² ==> 36

height = 9 in

Volume = (1/3) x 36 x 9

= 108 cubic inches