UNDERSTAND BOX AND WHISKER PLOT

A box and whisker plot (or simply box plot) is a visual display of the descriptive statistics of a data set. It shows 

• Minimum value 
• The lower quartile (Q₁)
• the median (Q₂)
• the upper quartile (Q₃)
• the maximum value

These five numbers form what is known as the five number summary of a data set

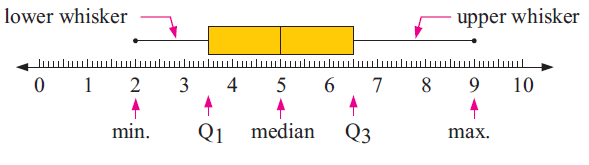
• The rectangular box represents the "middle" half of the data set.
• The lower whisker represents the 25% of the data with smallest values.
• The upper whisker represents the 25% of the data with greatest values.
  

Problem 1 :

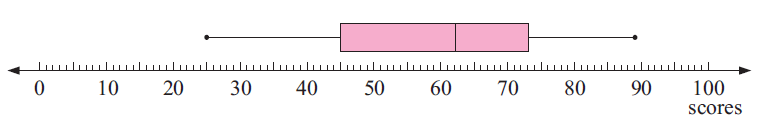
The box plot shown summarises the points scored by a basket ball team. Complete the statements about the results.

a)  The highest score was ________ points.

b)  The lowest score was ________ points.

c)  Half of the scores were greater than or equal to  _____ points.

d)  The top 25% of the scores was ______ points.

e)  The middle half of the scores were between _____ and ____ points.

f)  Find the range of the data set.

g) Find the interquartile range of the data set.

Solution :

a) The highest score is 89.

b)  The lowest score is 45.

c)  Half of the scores were greater than or equal to 62 points.

d)  To figure out top 25% of the score, we have to look the upper whisker.

The top 25% of the scores was at least 73 points.

e) To find the middle half of the scores, we have to look at the box, 

So, the middle half of the scores were between 45 and 73 points.

f) Range :

= Greatest value - smallest value

= 89 - 25

= 64

g) IQR = Q3 - Q1

Q3 = 73, Q1 = 45

IQR = 73 - 45

= 28

Problem 2 :

The box & whisker graph below which shows the test results of a math class.

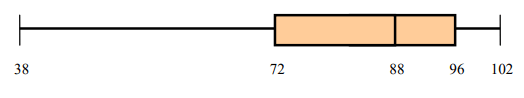
a)  What was the high score on the test?

b)  What percent of the class scored above a 72?

c)  What was the median score on the test?

d)  What percent of the class scored between 88 & 96?

e)  Do you think that this test was too hard for the students? Explain.

Solution :

a) Highest score is where the whisker ends, that is 102. 

b) Here Q1 = 72, before 72 the lower whisker shows 25% of the data. The box shows 50% of the data and the upper whisker shows 25% of the data

So, (50 + 25), that is 75% of class scores more than 72

c)  Median score is 88.

d) In between 88 to 96, 25% of the data is shown.

e) No, it is too easy. Because 75% of the students scored more than 72 and 50% of students has scored more than 88.

Problem 3 :

The box & whisker graph below that shows how much time was spent per night on homework for sophomore class at a certain high school during September.

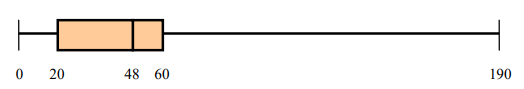
a)  What percent of the sophomores spend more than 60 minutes on homework per night?

b)  What is the range of times that the middle 50% of the sophomores spend on homework per night?

c)  How many sophomores do not do homework? 

d)  What percent of the sophomores spend less than 20 minutes per night on homework?

Solution :

a) 25% of sophomores spend more than 60 minutes on homework per night.

b) Range of middle 50% of the data :

= 60 - 20

= 40

So, the middle 50% of sophomores will spend 40 minutes on homework per night.

c)  How many people are spending 0 minutes to do their homework = number of students who do not do homework

So, the answer is at least 1.

d)  25% of the sophomores spend less than 20 minutes per night on homework.

Problem 3 :

The box & whisker graphs below that compare homework time per night with TV time per night for the same group of sophomores

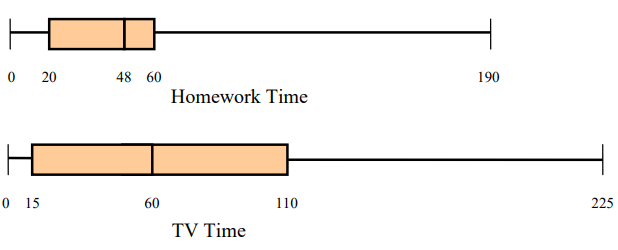
a)  What percent of the sophomores watch TV for at least 15 minutes per night?

b) What is the 3rd quartile for the TV time data?

c) Is it more common for a sophomore at this high school to spend more than 1 hour on homework or more than 1 hour watching TV? Explain.

Solution :

a)  15 minutes lies between 0 to 20 minutes (lower whisker). So, 75% of the sophomores watch TV for at least 15 minutes per night

b) Third quartile is 60.

c)  1 hour = 60 minutes.

From the first box and whisker plot, 25% of the sophomore spend more than 1 hour on homework.

From the second whisker plot 50% of the sophomore spend more than 1 hour on watching TV.

So, it is more common for a sophomore at this high school watch TV.

Problem 4 :

The accompanying box and whisker plot represents the scores earned on a math test.

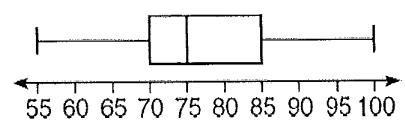
1)  What is the median score ?

a)   75    b)  70     c)  85    d) 77

2)  What score represents the first quartile ?

a)   55    b)  70     c)  100    d) 75

3)  What statement is not true about the box and whisker plot shown ?

a)  75 represents the mean score 

b)  85 represents the 3rd quartile

c)  100 represents the maximum score

d)  55 represents the minimum score

4)  A score of an 85 on the box and whisker plot shown refers to :

a)  the third quartile    b)  the maximum score   c)  the median     d) the mean

Solution :

1)  Median score is 75

2)  First quartile (Q1) = 70

3) In option a, 75 represents the median score. But the given statement says it is mean. So, it is incorrect.

4) 85 is the third quartile.