TRIGNOMETRY AND INVERSE TRIGONOMETRIC FUNCTIONS WORKSHEET

Find the exact value of the expression.

Problem 1 :

Solution

Problem 2 :

Solution

Problem 3 :

Solution

Problem 4 :

Find the exact value of the expression.

Solution

Problem 5 :

Use a right triangle to write the expression as an algebraic expression. Assume that x is positive and in the domain of the given inverse trigonometric function.

sin (tan^-1 x)

Solution

Problem 6 :

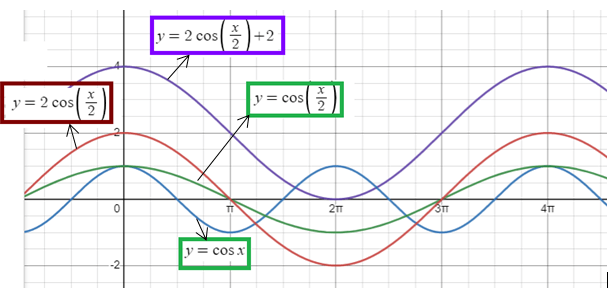
Use a transformations to graph the function.

y = 2 cos (1/2) x + 2

Solution

Problem 7 :

Use the given information to find the exact value of the expression.

sin θ  = 15/17, θ lies in quadrant I

Find cos 2θ.

Solution

Problem 8 :

Find all solutions of the equation.

2 cos x - 1 = 0

Solution

Problem 9 :

Solve the equation on the interval [0, 2π]

cos x + 2 cos x sin x = 0

Solution

Problem 10 :

Solve the equation on the interval [0, 2π]

2 cos²x + sin x - 2 = 0

Solution

Answer Key

1) 60°

2)  45°

3)  45°

4)  3/5

5)  

6)

7)  -161/289

8)  x = π/3 + 2nπ (n is a integer) and (2π is a radians)

9) x = 7π/6 

10)  

sin x = 0 when x = 0, π, 2π

sin x = 1/2 when x = π/6, 5π/6.