TRANSLATION OF GEOMETRIC FIGURES

The object A has been translated to give an image B in each diagram. Give the translation in each case.

Problem 1 :

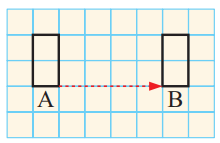
Solution :

Observing the vertex of A to vertex of rectangle B, only horizontal movement is done.

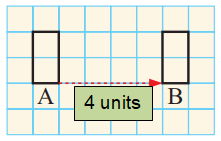
Moved 4 units right, so the required translation vector is (4, 0).

Problem 2 :

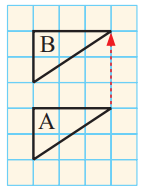
Solution :

Observing the vertex of A to vertex of triangle B, only vertical  movement is done.

Moved 3 units up, so the required translation vector is (0, 3).

Problem 3 :

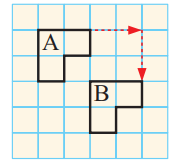
Solution :

Observing the vertex of A to vertex of B, both horizontal and vertical movements are done.

Number of horizontal movements = 2 (right, so +)

Number of vertical movements = -2 (down, so -)

So, the required translation vector is (2, -2).

Problem 4 :

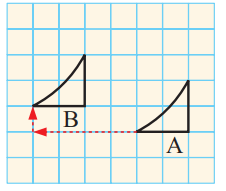
Solution :

Observing the vertex of A to vertex of B, both horizontal and vertical movements are done.

Number of horizontal movements = -4 (left, so -)

Number of vertical movements = 1 (up, so +)

So, the required translation vector is (-4, 1).

Problem 5 :

Solution :

Observing the vertex of A to vertex of B, both horizontal and vertical movements are done.

Number of horizontal movements = 3 (right, so +)

Number of vertical movements = -2 (down, so -)

So, the required translation vector is (3, -2).

Problem 5 :

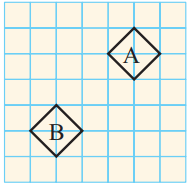
Solution :

Observing the vertex of A to vertex of B, both horizontal and vertical movements are done.

Number of horizontal movements = -3 (left, so -)

Number of vertical movements = -3 (down, so -)

So, the required translation vector is (-3, -3).

Problem 6 :

a)  Write down the coordinates of A, B, C and D.

b)  Each point is translated 5 units to the right and 2 units up. What are the coordinates of the image A', B', C' and D'

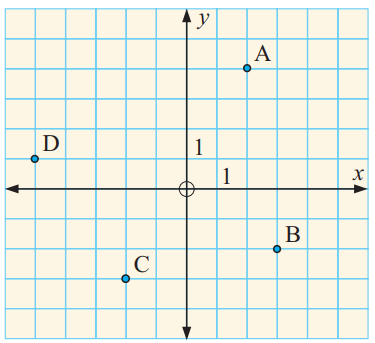
Solution :

A (2, 4), B(3, -2), C(-2, -3) and D(-5, 1).

5 units to the right, so +5

2 units up, so +2