TRANSFORMATIONS OF QUADRATIC FUNCTIONS

Translation

f(x) = a(x − h)² + k

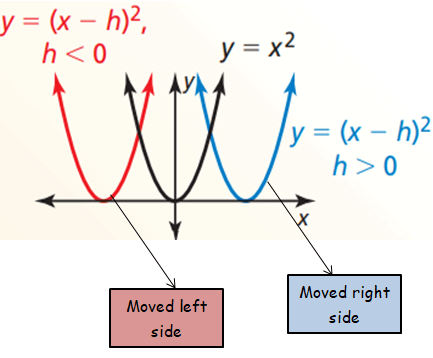
Horizontal translation :

Moving the graph towards left or right.

h > 0, then move the graph right.

h < 0, then move the graph left.

Vertical translation :

Moving the graph towards up or down.

k > 0, then move the graph up.

k < 0, then move the graph down.

Reflection

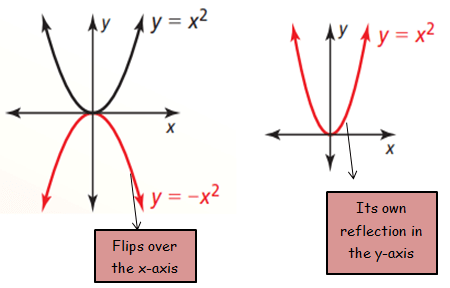
Reflection across x-axis :

f(x) = x²

-f(x) = -x²

Reflection across y-axis :

f(x) = x²

f(-x) = (-x)²

Shrinks and Stretches

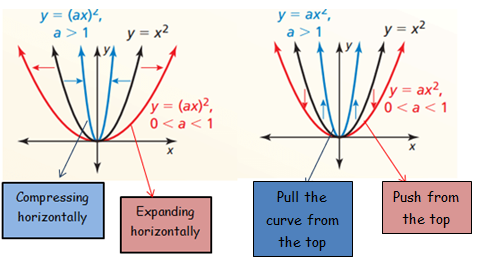
Horizontal stretches and shrinks :

f(x) = x²

f(ax) = (ax)²

If a > 1 (horizontal shrink)

If 0 < a < 1 (horizontal stretch)

Vertical stretches and shrinks :

f(x) = x²

a f(x) = ax²

If a > 1 (vertical stretch)

If 0 < a < 1 (vertical shrink)

Problem 1 :

Which function includes a translation of 3 units to the left?

(a)  f(x) = (x + 3)² + 1             (b)  f(x) = (x - 3)² + 1

(c)  f(x) = 3x² + 1                    (d)  f(x) = (x + 1)² - 3

Solution :

Considering the quadratic function in vertex form

y = a(x - h)² + k

Here the value of h decides the horizontal movement. Since we move the curve left 3 units, the value of h will be -3

Option a :

f(x) = (x + 3)² + 1

f(x) = (x - (-3))² + 1

h = -3

So, option a is correct.

Problem 2 :

Which equation shows a translation of 3 left and vertical compression by a factor of 2 to the graph of y = x²?

(a)  f(x) = 2(x - 3)²                (b)  f(x) = (1/2)(x - 3)²

(c)  f(x) = 2(x + 3)²                 (d)  f(x) = (1/2)(x + 3)²

Solution :

Translation of 3 units to the left. So, h = -3

Parent function is y = x²

Vertical compression means the curve will be observed towards y-axis.

Then, the value of a will be 0 < a < 1. Considering option d,

f(x) = (1/2)(x -(-3))²

h = -3 and a = 1/2 (0 < a < 1)

Problem 3 :

Which equation describes a parabola that opens downward, is congruent to y = x², and has its vertex at (0, 3)?

(a)  f(x) = (x + 3)² - 1                (b)  f(x) = -x² + 3

(c)  f(x) = -(x - 3)²                 (d)  f(x) = x² + 3

Solution :

Parent function :

y = x²

Vertex is at (0, 3). So, h = 0 and k = 3

Opens down, then y = -x²

f(x) = -(x - 0)² + 3

f(x) = -x² + 3

Problem 4 :

List the sequence of steps required to graph the function

y = -(x + 4)² - 6

(a) horizontal translation 4 units to the right, vertical compression by a factor of 1, vertical translation 6 units down

(b) horizontal translation 4 units to the right, reflection in x-axis, vertical translation 6 units down

(c) horizontal translation 4 units to the left, vertical translation 6 units up, reflection in x-axis

(d) horizontal translation 4 units to the left, reflection in x-axis, vertical translation 6 units down

Solution :

Considering the function y = -(x + 4)² - 6 with y = a(x - h)² + k

Since we have negative at the beginning, it must be reflection.

a = -1 (Reflection)

h = -4 (horizontal translation to the left)

k = -6 (vertical translation down)

So, option d is correct.

Problem 5 :

Which function matches the graph?

(a)  f(x) = -2(x - 3)² + 1                (b)  f(x) =  (x + 3)² + 2 

(c)  f(x) = -2(x + 3)² - 1             (d)  f(x) = (1/2) (x - 3)² - 1

Solution :

By observing the curve, the vertex is at (-3, -1).

So, (h, k) ==> (-3, -1)

In option c only, we have the given vertex. So, option c is correct.

Problem 6 :

Consider a parabola P that is congruent to 

y = x²

opens upward, and has vertex (-1, 3). Now find the equation of a new parabola that results if P is reflected in the x-axis and translated 3 units down

(a)  f(x) = -(x + 4)² + 3                (b)  f(x) =  (x - 1)² + 6

(c)  f(x) = -(x + 1)²                (d)  f(x) = -(x - 2)² + 3

Solution :

Parent function :

y = x²

Since (h, k) is (-1, 3)

y = (x - (-1))² + 3

Equation of quadratic function with vertex (-1, 3) :

y = (x + 1)² + 3

Reflected in the x-axis, so y = -(x + 1)² + 3

Translated 3 units down, so 

y = -(x + 1)² + 3 - 3

y = -(x + 1)²

So, the required function is y = -(x + 1)², option c is correct.

Problem 7 :

The graphs of y = x² and another parabola are shown below. What is a possible equation for the second parabola?

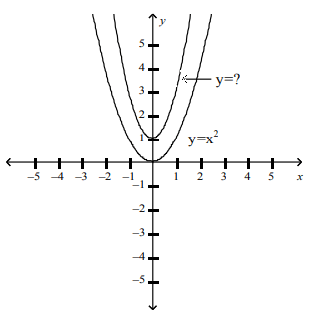
(a)  f(x) = 2x² + 1                (b)  f(x) =  (1/2)x² + 1

(c)  f(x) = 2(x + 1)²                (d)  f(x) = -2x² - 1

Solution :

Comparing with the parent function, y = x²

the translated graph moved 1 unit up and there is no horizontal translation. It looks like compression towards y-axis

The scale factor should lie in between 0 to 1. Accordingly options given, option b works for the given condition.

Problem 8 :

Describe the following transformations on the function y = x². 

y = -(x – 2)²

Solution :

Since h = 2 and a = -1

the graph should be reflected across y-axis and move the parent function 2 units right.

Problem 9 :

Describe the following transformations on the function y = x². 

y = (1/3)(x + 2)² + 3

Solution :

Since h = -2, k = 3 and a = 1/3 (horizontal stretch)

the graph should be moved 2 units left, 3 units down and