STANDARD DEVIATION FROM FREQUENCY TABLE WITH INTERVALS
To find the standard deviation of n values, we use the formula given below.
Problem 1 :
The weekly wages (in dollars) of 90 workers in a steel yard are given alongside :
Find estimates of the mean wage and the standard deviation of the wages.
|
Wage ($) 360 - 369.99 370 - 379.99 380 - 389.99 390 - 399.99 400 - 409.99 410 - 419.99 420 - 429.99 430 - 439.99 |
Number of Workers 5 16 27 16 12 8 4 2 |
Solution :
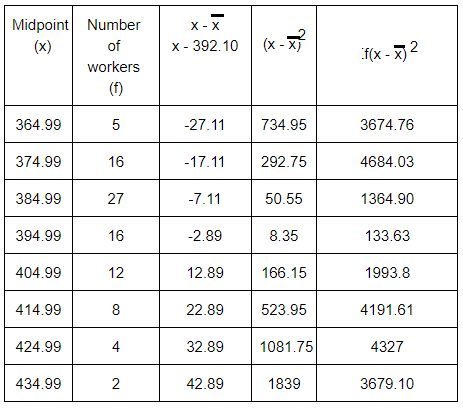
Mean wages = 392.10
Standard deviation = 16.34
Problem 2 :
A random sample of the weekly supermarket bills for a number of families was observed and recorded in the table given.
a. Find the mean bill and the standard deviation of the bills.
b. variance of the population
|
Bill ($) 70 - 79.99 80 - 89.99 90 - 99.99 100 - 109.99 110 - 119.99 120 - 129.99 130 - 139.99 140 - 149.99 |
No. of families 27 32 48 25 37 21 18 7 |
Solution :
a)
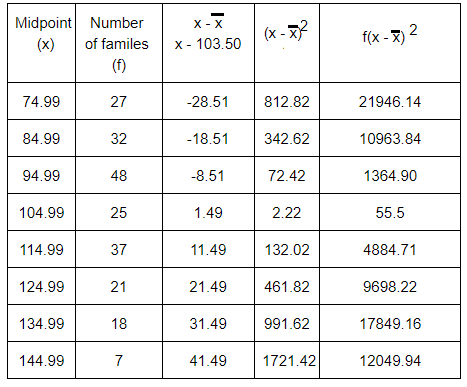
Mean = 103.50
Standard deviation = 19.14
b) Variance = 366.33
Problem 3 :
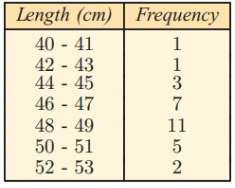
The length of 30 randomly selected 12 day old babies were measured to the nearest cm and the following data obtained.
i) Find the mean length and standard deviation of the lengths.
ii) Find the variance.
Solution :
Since the given interval is not continuous, let us make it as continuous interval.
|
Length(x) 39.5 - 41.5 41.5 - 43.5 43.5 - 45.5 45.5 - 47.5 47.5 - 49.5 49.5 - 51.5 51.5 - 53.5 |
Midpoint 40.5 42.5 44.5 46.5 48.5 50.5 52.5 |
Frequency 1 1 3 7 11 5 2 |
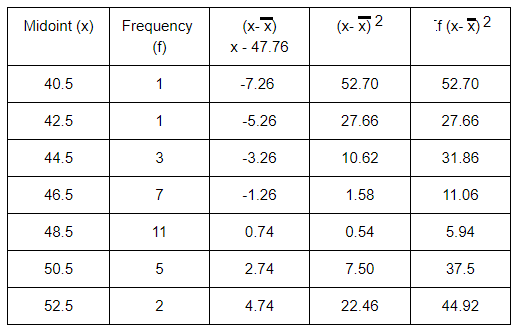
i) Mean = 47.76, standard deviation = 2.65
ii) Variance = 7.05
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling