SPECIAL RIGHT TRIANGLES

There are two types of special right triangles

• 30 - 60 - 90 right triangle
• 45 - 45 - 90 right triangle

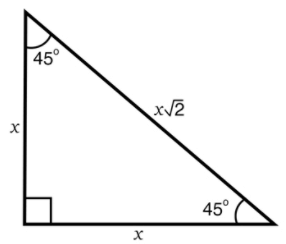
In a 45-45-90 triangle

the hypotenuse is 2 times as long as a leg

An isosceles right triangle is also called a 45-45-90 triangle.

In a 30 - 60 - 90 triangle,

the hypotenuse = twice as long as the shorter leg

and

the longer leg = √3 times as long as the shorter leg.

Find the value of x in each triangle

Problem 1 :

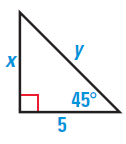
Solution :

It comes under 45-45-90 right triangle. 

Hypotenuse (y) = 2(Smallest sides)

y = 2(5)

y = 10

x = 5

Problem 2 :

Solution :

It comes under 30-60-90 right triangle. 

Hypotenuse = b

Smallest leg = 12

Longer leg (a) = √3(shorter leg)

= 12√3

Hypotenuse = 2(shorter leg)

= 2(12)

= 24

Problem 3 :

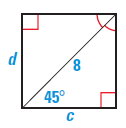
Solution :

Hypotenuse = 8

Shorter leg = c

c = d

Hypotenuse = 2(Shorter leg)

8 = 2c

c = 4 and d = 4

Problem 4 :

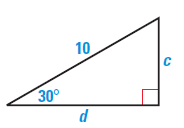
Solution :

Shorter leg = c, hypotenuse = 10, longer leg = d

Problem 5 :

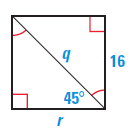
Solution :

Shorter leg = r = 16, hypotenuse = q

Hypotenuse = 2(Shorter leg)

q = 2(16)

q = 32

Problem 6 :

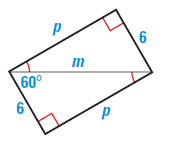
Solution :

Hypotenuse  = m

Shorter leg = 6 and 

longer leg = p

Hypotenuse = 2(Shorter leg)

m = 2(6)

m = 12

Longer leg = √3 (shorter leg)

P = 6√3

Problem 7 :

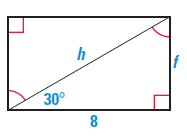
Solution :

Hypotenuse  = h

Shorter leg = f and 

longer leg = 8

Longer leg = √3 (shorter leg)

8 = √3(f)

f = 8/√3

f = (8/√3) ⋅ (√3/√3)

f = 8√3/3

Hypotenuse (h) = 2(shorter leg)

= 2(8√3/3)

= 16√3/3

Problem 8 :

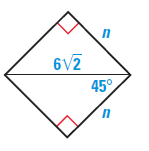
Solution :

Hypotenuse = 6√2

Shorter leg = n

Hypotenuse = 2(Shorter leg)

6√2 = 2n

n = 3√2

Problem 9 :

The side length of an equilateral triangle is 5 cm. Find the length of an altitude of the triangle.

Solution :

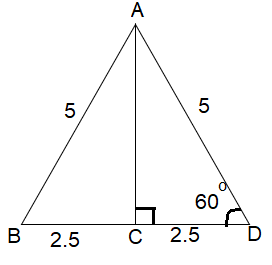
Shorter leg = CD = 2.5

Longer leg = AC

Hypotenuse = AD = 5

Longer leg = √3(Shorter leg)

= √3(2.5)

= 2.5√3

Problem 10 :

The perimeter of the square is 36 inches. Find the length of the diagonal.

Solution :

Let x be the side length of square.

Perimeter of the square = 36

4x = 36

x = 9

Using Pythagorean theorem :

x² + x² = (Length of diagonal)²

9² + 9² = (Length of diagonal)²

81 + 81 = (Length of diagonal)²

Length of diagonal = √162

= 9√2