SOLVING SYSTEM OF LINEAR EQUATIONS WITH SPECIAL CASES

A set of linear equations with the same two variables is called a system of linear equations.

The system of linear equations can have the following solutions.

(i) Unique solutions

(ii)  No solution

(iii) Infinitely many solution

Unique solution :

Intersecting lines will have one solution (or) unique solution.

The point of intersection is known as solution.

No solution :

The parallel lines will never meet. So, there is no point of intersection that is no solution.

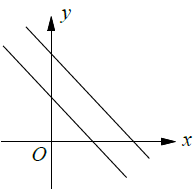
Parallel lines will have the same slope but different y-intercepts.

Infinitely many solution :

Two lines that lie exactly on top of each other or we can say that one line is exactly lying on top of another line are called coincident lines.

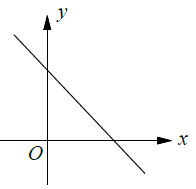
Coincident lines will have the same slope and same y-intercepts.

Problem 1 :

For what value of c will the system of equations below have no solution ?

cx - 2y = 6

3x + 4y = 4

Solution :

cx - 2y = 6  -----(1)

3x + 4y = 4 -----(2)

Since the system has no solution, they must be parallel and they will have same slope.

m₁ = m₂

c/2 = -3/4

Multiply by 2 on both sides.

c = (-3/4) ⋅ 2

c = -3/2

So, the value of c is -3/2.

Problem 2 :

For what value of b will the system of equations below have infinitely many solution ?

-2x + y = 4

5x - by = -10

Solution :

-2x + y = 4 -------(1)

5x - by = -10 -------(2)

Since the system has infinitely many solutions, they must be coincident lines and they will have same slope and same y-intercepts. 

Equating the y-intercepts, we get

4 = 10/b

Multiplying by b on both sides.

4b = 10

Divide by 4 on both sides.

b = 10/4

b = 5/2

Problem 3 :

ax - y = 0

x - by = 1

In the system of equations above, a and b are constants and x and y are variables. If the system of equations above has no solution. What is the value of a ⋅ b ?

Solution :

Since the system has no solution, they must be parallel lines and they will have equal slopes.

By equating slopes, we get

a = 1/b

Multiply by b on both sides, we get

a ⋅ b = 1

Problem 4 :

2x - ky = 14

5x - 2y = 5

In the system of equations above, k is constant and x and y are variables. For what values of k will the system of equations have no solution ?.

Solution :

Since the system has no solution, slopes will be equal.

Slopes will be equal.

2/k = 5/2

Doing cross multiplication, we get

4 = 5k

Dividing by 5 on both sides.

k = 4/5

Without graphing, determine whether the system of linear equations has one solution, infinitely many solutions, or no solution. Explain your reasoning.

Problem 5 :

y = 5x - 9

y = 5x + 9

Solution :

Comparing the given equations with slope intercept form y = mx + b

y = 5x - 9

y = 5x + 9

Slopes are equal but y-intercepts are not. Then the lines are parallel. So, it has no solution.

Problem 6 :

y = 6x + 2 

y = 3x + 1

Solution :

Comparing the given equations with slope intercept form y = mx + b

y = 6x + 2 

y = 3x + 1

Slopes are not equal, then they must be intersecting lines.

Problem 7 :

y = 8x - 2

y - 8x = -2

Solution :

Comparing the given equations with slope intercept form y = mx + b

y = 8x - 2

y = 8x - 2

Since slope and y-intercept both are equal, the lines must be co-incident lines. It must have infinitely many solutions.