SOLVING SPECIAL RIGHT TRIANGLES WITH MULTI VARIABLES

There are two types of special right triangles

• 30 - 60 - 90 right triangle
• 45 - 45 - 90 right triangle

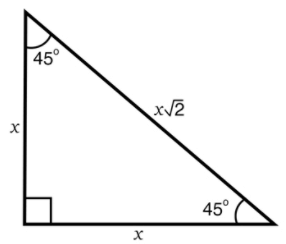
In a 45-45-90 triangle

the hypotenuse is 2 times as long as a leg

An isosceles right triangle is also called a 45-45-90 triangle

In a 30 - 60 - 90 triangle,

the hypotenuse = twice as long as the shorter leg

and

the longer leg = √3 times as long as the shorter leg.

Find the value of each variable. Leave your answer in simplest radical form.

Problem 1 :

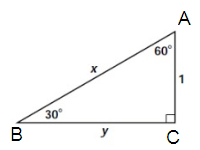
Triangle ABC is a 30^º - 60^º - 90^º triangle.

Finding the value of x :

By 30^º - 60^º - 90^º triangle theorem,

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = x, and shorter length = 1.

x = 2 ⋅ 1

x = 2

So, the value of x is 2.

Finding the value of y :

By 30^º - 60^º - 90^º triangle theorem,

longer length = √3 ⋅ shorter length

Here, longer length = y, and shorter length = 1.

y = √3 ⋅ 1

y = √3

So, the value of y is √3.

Problem 2 :

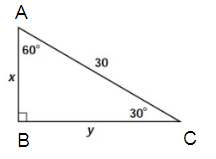
∠ABC is a 30^º - 60^º - 90^º triangle.

Finding the value of x :

By 30^º - 60^º - 90^º triangle theorem,

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = 30, and shorter length = x.

30 = 2 ⋅ x

Dividing 2 on both sides.

2x/2 = 30/2

x = 15

So, the value of x is 15.

Finding the value of y :

By 30^º - 60^º - 90^º triangle theorem,

longer length = √3 ⋅ shorter length

Here, longer length = y, and shorter length (x) = 15.

y = √3 ⋅ 15

y = 15√3

So, the value of y is 15√3.

Problem 3 :

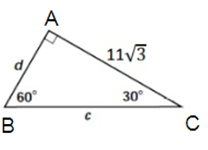
Triangle  ABC is a 30^º - 60^º - 90^º triangle.

Finding the value of d :

By 30^º - 60^º - 90^º triangle theorem,

longer length = √3 ⋅ shorter length

Here, longer length = 11√3, and shorter length = d.

11√3 = √3 ⋅ d

Dividing √3 on both sides.

11√3/√3 = √3/√3d

d = 11

So, the value of d is √3.

Finding the value of c :

By 30^º - 60^º - 90^º triangle theorem,

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = c, and shorter length (d) = 11.

c = 2 ⋅ 11

c = 22

So, the value of c is 22.

Problem 4 :

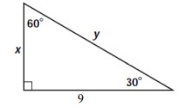
∠ABC is a 30^º - 60^º - 90^º triangle.

Finding the value of y :

By 30^º - 60^º - 90^º triangle theorem,

longer length = √3 ⋅ shorter length

Here, longer length = 9, and shorter length = x.

9 = √3 ⋅ x

x = 9/√3

Rationalizing the denominator, we get

x = 9√3/3

x = 3√3

Finding the value of y :

By 30^º - 60^º - 90^º triangle theorem,

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = y, and shorter length (x) = 3√3

y = 2 ⋅ 3√3

y = 6√3

So, the value of y is 6√3

Problem 5 :

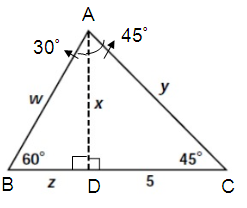
Solution :

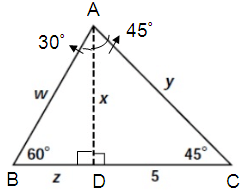
Finding the value of x :

ABD is a 30^º - 60^º - 90^º triangle.

AD = CD

x = 5

Finding the value of y :

ADC is a 45^º - 45^º - 90^º triangle.

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = y, and shorter length (x) = 5.

y = 2 ⋅ 5

y = 10

So, the value of y is 10.

Finding the value of z :

ABD is a 30^º - 60^º - 90^º triangle.

longer length = √3 ⋅ shorter length

Here, longer length (x) = 5, and shorter length = z.

5 = √3 ⋅ z

Divide each side by √3.

5/√3 = √3/√3 ⋅ z

5/√3 = z

Multiply numerator and denominator by √3.

5/√3 ⋅ √3/√3 = z

5√3/3 = z

So, the value of z is 5√3/3.

Finding the value of w :

ABD is a 30^º - 60^º - 90^º triangle.

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = w, and shorter length (z) = 5√3/3.

w = 2 ⋅ 5√3/3

w = 10√3/3

So, the value of w is 10√3/3.

Problem 6 :

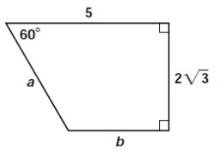
Solution :

Drawing perpendicular from one vertex, we may get special right triangle.

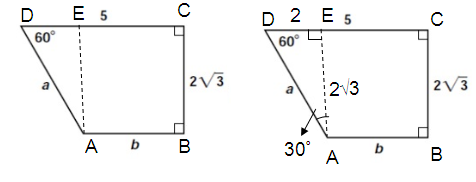
AD² = DE² + AE²

a² = 2² + (2√3)²

a² = 4 + (4 × 3)

= 4 + 12

= 16

a = √16

a = 4

AB = DC – DE

b = 5 – 2

b = 3 So, the value of a and b are 4 and 3 respectively.

Problem 7 :

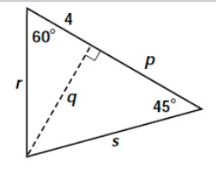
Solution :

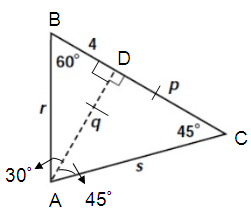
Triangle ABD is a 30^º - 60^º - 90^º triangle.

Finding the value of q :

longer length = √3 ⋅ shorter length

Here, longer length = q, and shorter length = 4.

q = 4√3

Finding the value of r :

Hypotenuse = 2 ⋅ shorter length

Here, hypotenuse = r, and shorter length = 4

r = 2 ⋅ 4

r = 8

Finding the value of p :

∠ADC is a 45^º - 45^º - 90^º triangle.

AD = CD

q = p

4√3 = p

Finding the value of s :

∠ADC is a 45^º - 45^º - 90^º triangle.

Using Pythagorean theorem

AC² = DC² + AD²

s² = p² + q²

= (4√3)² + (4√3)²

= 16 × 3 + 16 × 3

= 48 + 48

s² = 96

s = √(16 × 6)

= √(4 × 4 × 6)

s = 4√6

So, the value of p, q, r and s are 4√3, 4√3, 8 and 4√6 respectively.