SOLVING SIMPLE CUBIC EQUATIONS WORKSHEET

To solve cubic equation, we should know the inverse operation of cube.

To remove cube or raised to the power of 3, we have to take cube root on both sides.

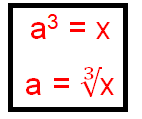
Step 1 :

Using inverse operations isolate the terms which has the highest exponent 3 in one side of the equal sign.

Step 2 :

After isolating the terms which has the highest exponent 3, to remove cube we will take cube root on both side of the equal sign.

Step 3 :

Decomposing the numerical value that we have inside the cube root as product of prime factors, for every three same values inside the cube root, take one value out of the radical sign.

Solve for x :

Problem 1 :

x³ = 64

Solution :

x³ = 64

x = ∛64

x = 4

Problem 2 :

x³ - 27 = 0

Solution :

x³ - 27 = 0

x³ = 27

x = ∛27

x = 3

Problem 3 :

x³ + 216 = 0

Solution :

x³ + 216 = 0

x³ = -216

x = ∛-216

x = -6

Problem 4 :

x³ = 0

Solution :

x³ = 0

x = 0

Problem 5 :

x³ = -125

Solution :

x³ = -125

x = ∛25

x = -5

Problem 6 :

x³ + 8 = 0

Solution :

x³ + 8 = 0

x³ = -8

x = ∛-8

x = -2

Problem 7 :

x³ - 1 = 0

Solution :

x³ - 1 = 0

x³ = 1

x = ∛1

x = 1

Problem 8 :

x³ = -1000000

Solution :

x³ = -1000000

x = ∛-1000000

x = -100

Problem 9 :

x³ = 512

Solution :

x³ = 512

x = ∛512

x = 8

Problem 10:

x³ + 1 = 0

Solution :

x³ + 1 = 0

x³ = -1

x = ∛-1

x = -1