SOLVING REAL LIFE PROBLEMS INVOLVING SLOPE WORKSHEET

Problem 1 :

The percent y (in decimal form) of battery power remaining x hours after you turn on a laptop computer is 

y = -0.2x + 1

a)  Graph the equation

b)  Interpret the x and y-intercepts.

c) After how many hours is the battery power at 75%.

Solution

Problem 2 :

The amount y (in gallons) of gasoline remaining in a gas tank after driving x hours is

y = −2x + 12

(a) Graph the equation.

(b) Interpret the x- and y-intercepts.

(c) After how many hours are there 5 gallons left?

Solution

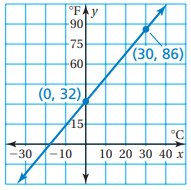
Problem 3 :

The graph relates temperatures y (in degrees Fahrenheit) to temperatures x (in degree Celsius)  

a) Find the slope and y-intercept

b) Write an equation of the line

c) What is the mean temperature of Earth in degrees Fahrenheit)

Solution

Problem 4 :

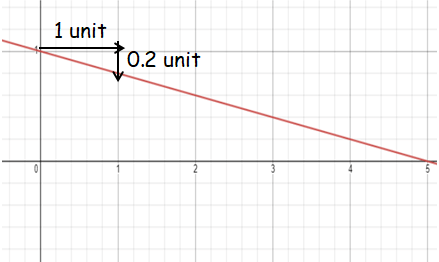
You are downloading a song. The percent y (in decimal form) of megabytes remaining to download after x seconds is

y = −0.1x + 1.

a) Graph the equation.

b) Interpret the x- and y-intercepts.

c) After how many seconds is the download 50% complete?

Solution

Answer Key

1)  a) 

b) The x-intercept is 5. So, the battery lasts 5 hours. The y-intercept is 1. So, the battery power is at 100% when you turn on the laptop

c)  After 1.25 hours, the battery will have power of 75%.

2) 

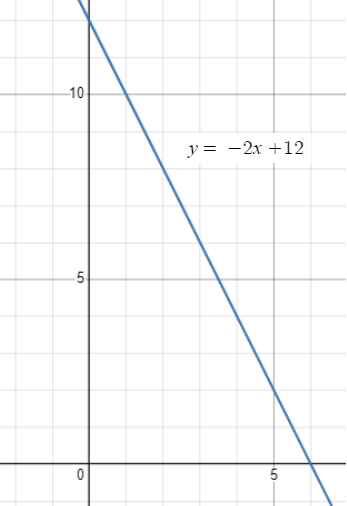
b) After 6 hours only, the quantity of gasoline will become 0.

Initially capacity of the tank is 12 gallons.

c)  After 3.5 hours  gallons left.

3)  a)  Slope = 9/5 and y-intercept is at 32.

b) y = (9x/5) + 32

c)  y = 59

4)  a) Slope = -0.1 and y-intercept is 1

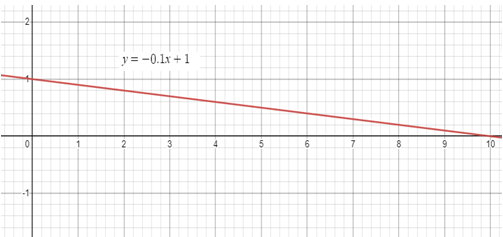
b)  x-intercept is 10 and y-intercept is 1.

c) x = 5

Problem 1 :

Felicia Johnson paid $125 to join a tennis club. She pays an additional $5 every time she uses one of the club's tennis courts. Use this information to answer the following questions.

a. Write an equation that describes Felicia's total cost for playing tennis as a function of the number of times she plays. Let C = the total cost and n = the number of times she plays.

b. Describe the domain and range of the function.

c. Felicia does not want to spend more than $275 to play tennis during the summer.

What is the maximum number of times that she can play tennis on the club's courts for this amount?

Solution

Problem 2 :

Members of the soccer team are walking to raise money for a local shelter. 92 sponsors pledged a dollar per kilometer. Some sponsors gave additional donations that did not depend on the distance students walked.

a. Write a verbal model that relates the total amount A of money raised by the soccer team to the number n of kilometers walked and the amount d given in additional donations.

b. The team walked 8 kilometers and raised a total amount of $842. Is there enough information to find how much money came from additional donations that did not depend on walking distance? If so, find this amount.

Solution

Problem 3 :

A grocer knows that if he sells his canned hams for $8 each, he can sell 950 per month, and if he sells the same hams for $10, he will sell 900 per month. Assuming the relationship between price and sales is linear, write an equation you could use to predict sales for other prices.

Solution

Problem 4 :

If a large factory sells its new gadgets for $5 each, it can sell 1050 per month, and if it sells the same gadgets for $9, it will sell 900 per month. Assuming the relationship between price and sales is linear, predict the monthly sales of gadgets to the nearest whole number if the price is $12.

Solution

Problem 5 :

A balloon is released from the top of a building. The graph shows the height of the balloon over time

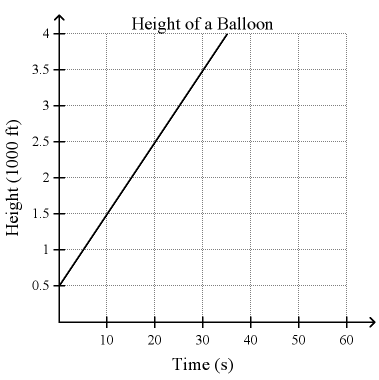
a. What does the slope and y-intercept reveal about the situation?

b. For a similar situation, the slope 35 is and the y-intercept is 550. What can you conclude?

Solution