SOLVING LINEAR AND QUADRATIC EQUATIONS GRAPHICALLY

Problem 1 :

y = x² - 2x - 3

y = 2x - 3

Solution:

y = x² - 2x - 3

y + 3 = x² - 2x

y + 3 + 1 = x² - 2x + 1

y + 4 = x² - 2x + 1

y + 4 = (x - 1)²

Vertex Form:

y = (x - 1)² - 4

y = a(x - h)² + k

Vertex (h, k) = (1, -4)

x-intercept: y = 0

y = x² - 2x - 3

x² - 2x - 3 = 0

(x + 1) (x - 3) = 0

x = -1 and x = 3

x-intercept are (-1, 0) and (3, 0).

y-intercept: x = 0

y = (0)² - 2(0) - 3

y = -3

y-intercept = (0, -3)

Number of solutions : 2

So, the solutions are (0, -3) and (4, 5).

Problem 2 :

y = -(x + 2)² + 5

y = 5

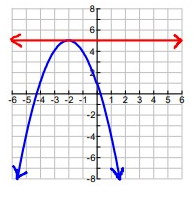
Solution:

From the given graph, the intersect point is (-2, 5).

Number of solution : 1

So, the solution is (-2, 5).

Problem 3 :

y = x² - 2x + 4

y = x - 1

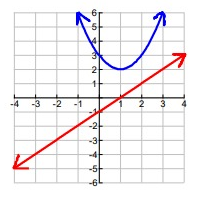
Solution:

From the given graph, there is no intersect point.

Number of solution zero.

Problem 4 :

y = (x + 2)² - 6

y = 4x - 2

Solution:

y = (x + 2)² - 6

Vertex Form:

y = a(x - h)² + k

Vertex (h, k) = (-2, -6)

y = (x + 2)² - 6

y = x² + 4x + 4 - 6

y = x² + 4x - 2

x-intercept: y = 0

x² + 4x - 2 = 0

x-intercept = (0.449, 0) and (-4.449, 0)

y-intercept: x = 0

y = x² + 4x - 2

y = -2

y-intercept = (0, -2)

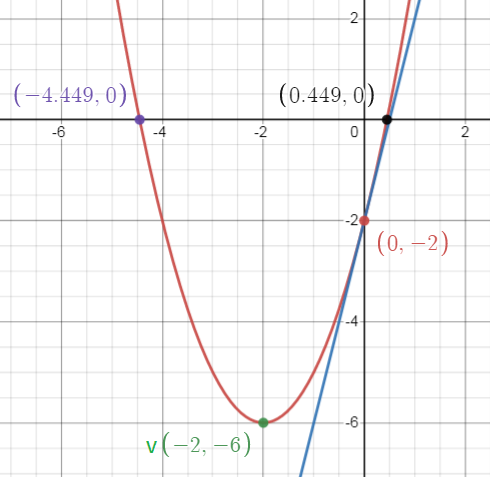
Number of solution 1.

So, the intersecting point is (0, -2).

Problem 5 :

y = x² - 2x - 3

y = -5

Solution:

y = x² - 2x - 3

y + 3 = x² - 2x

y + 3 + 1 = x² - 2x + 1

y + 4 = x² - 2x + 1

y + 4 = (x - 1)²

Vertex Form:

y = (x - 1)² - 4

y = a(x - h)² + k

Vertex (h, k) = (1, -4)

x-intercept: y = 0

y = x² - 2x - 3

x² - 2x - 3 = 0

(x + 1) (x - 3) = 0

x = -1 and x = 3

x-intercept are (-1, 0) and (3, 0)

y-intercept: x = 0

y = (0)² - 2(0) - 3

y = -3

y-intercept = (0, -3)

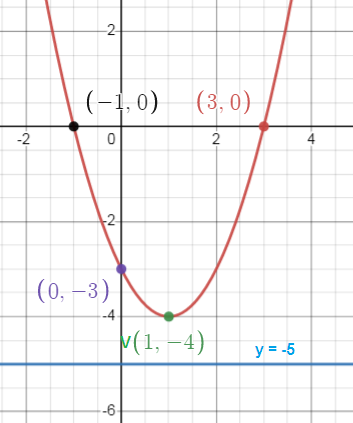
Number of solution zero.

So, there is no solution since the curves are not intersecting each other.

Problem 6 :

y = -x² + 2x + 7

y = -2x + 2

Solution:

y = -x² + 2x + 7

y = -(x² - 2x) + 7

y - 7 - 1 = -(x² - 2x + 1) 

y = -(x - 1)² + 8

Vertex Form:

y = a(x - h)² + k

Vertex (h, k) = (1, 8)

x-intercept: y = 0

x-intercept are (3.828, 0) and (-1.828).

y-intercept: x = 0

y = -x² + 2x + 7

y = 7

y-intercept = (0, 7)

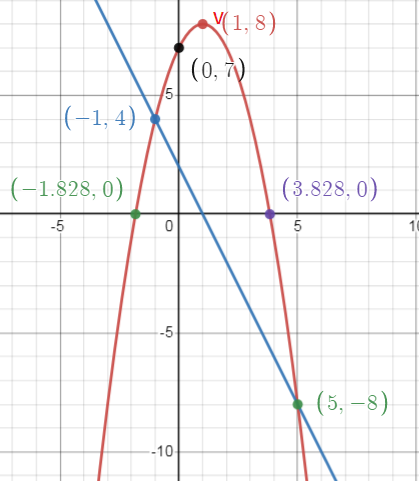
Number of solution = 2

So, the intersecting points are (-1, 4) and (5, -8).