SOLVING INEQUALITIES BY CLEARING THE FRACTIONS

Problem 2 :

(7/13)x  - 1 > 1/2

Solution :

Add 1 to both sides

(7/13)x  - 1 + 1 > (1/2) + 1

(7/13)x > 3/2

Multiply both sides by 13/7

(7/13)x (13/7) > (3/2) (13/7)

x > 39/14

Converting improper fraction into mixed fraction, we get

x > 2 11/14

Problem 3 :

(4/5) ≥ (2/3) - (2/7x)

Solution :

(4/5) ≥ (-2/7x) + (2/3)

Subtract 2/3 from both sides.

(4/5) - 2/3 ≥ (-2/7x) + 2/3 - 2/3

2/15 ≥ (-2/7)x

Since we multiply both sides by (-7/2), change inequality ≥ into ≤

(2/15) (-7/2) ≥ (-2/7)x (-7/2)

-7/15 ≤ x

Problem 4 :

(8/15x) – (17/30) < 7/10

Solution :

Add 17/30 to both sides

(8/15x) – (17/30) + 17/30 < (7/10) + 17/30

8/15x < 38/30

Multiply both sides by 15/8

(8/15)x (15/8) < (38/30) (15/8)

x < 19/8

Problem 5 :

(-4/11)z - 1 > (-8/11)

Solution :

Add 1 to both sides

(-4/11)z – 1 + 1 > (-8/11) + 1

(-4/11)z > 3/11

Since we multiply both sides by (-11/4), change inequality > into <

(-4/11)z (-11/4) > (3/11) (-11/4)

z < -3/4

Problem 6 :

(1/5k) + 14 ≤ 2/9

Solution :

Subtract 14 from both sides

(1/5k) + 14 – 14 ≤ 2/9 – 14

1/5k ≤ -124/9

Multiply both sides by 5

(1/5)k (5) ≤ (-124/9) (5)

k ≤ -620/9

Converting improper fraction into mixed fraction, we get

k ≤ -68  8/9

Problem 7 :

-31/4 < -13 + (7/8f)

Solution :

-31/4 < -13 + (7/8f)

Add 13 to both sides

-31/4 +13 < -13 + 13 + (7/8)f 

21/4 < 7/8f 

Multiply both sides by 8/7

(21/4) (8/7) < (7/8f) (8/7) 

6 < f

Problem 8 :

(1/7r) + (53/56) > 6/7

Solution :

Subtract 53/56 from both sides

(1/7)r + (53/56) – (53/56) > (6/7) – (53/56)

1/7r > -5/56

Multiply both sides by 7

(1/7)r (7) > (-5/56) (7)

r > -5/8

Problem 9 :

(5/6n) – (1/5) < -8/15

Solution :

Add 1/5 to both sides

(5/6)n – (1/5) + (1/5) < (-8/15) + (1/5)

5/6n < -5/15

Multiply both sides by 6/5

(5/6n) (6/5) < (-5/15) (6/5)

n < -2/5

Problem 10 :

(1/3) + (1/13d) ≥ 17/39

Solution :

Subtract 1/3 from both sides

(1/3) - (1/3) + (1/13)d ≥ (17/39) - (1/3)

1/13d ≥ 14/39

Multiply both sides by 13

(1/13d) (13) ≥ (14/39) (13)

d ≥ 14/13

d ≥ 1 1/13

Problem 11 :

Each visit to a water park costs $19.95. An annual pass to the park costs $89.95. Write an inequality to represent the number of times you would need to visit the park for the pass to be a better deal.

Solution :

Let x be the number of times.

Cost for each visit = $19.95

Annual pass to the park = $89.95

To get the better deal,

19.95x > 89.95

Dividing by 19.95 on both sides,

x > 89.95/19.95

x > 4.5

So, maximum at 5 times.

Problem 12 :

A thrill ride at an amusement park holds a maximum of 12 people per ride.

a. Write and solve an inequality to find the least number of rides needed for 15,000 people.

b. Do you think it is possible for 15,000 people to ride the thrill ride in 1 day? Explain.

Solution :

Maximum number of people to be seated = 12

a)

Let x be the required number of rides needed for 15000 people.

12x > 15000

x > 15000/12

x > 1250

The least number of rides needed is 1250, and it is likely not possible for 15000 people to ride in one day due to time constraints.

b)  To answer this, we must know the time taken for each ride. 

Problem 13 :

Describe and correct the error in solving the inequality

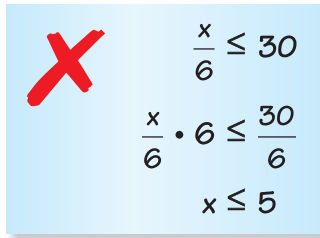
Solution :

By observing the steps above, to remove 6 which is at the denominator. We have to multiply by 6 on both sides.

x/6 ≤ 30

(x/6) ⋅ 6 ≤ 30 ⋅ 6

x ≤ 180

The error instead of multiplying on both sides, its been multiplied.

Problem 14 :

A one-way bus ride costs $1.75. A 30-day bus pass costs $42.

a. Write and solve an inequality to find the least number of one-way rides you must take for the 30-day pass to be a better deal.

b. You ride the bus an average of 20 times each month. Is the pass a better deal? Explain.

Solution :

a) The price of a one way ride x the number of one way rides is more than $42.

Let x be the number of one-way rides

1.75x > 42

x > 42/1.75

x > 24

So, you need to take more than 24 one-way rides for the pass to be a better deal.

b. No. The cost of 20 one-way rides is less than $42. So, the pass is not a better deal.