SOLVING COMPOUND INEQUALITITES
There are two operators in inequality. Those are
i) And
ii) or
How we use the operator and ?
The region that satisfies both conditions can be fixed as solution. n - and
How we use the operator Or ?
The region that satisfies any one of the conditions can be fixed as solution. u - or
Problem 1 :
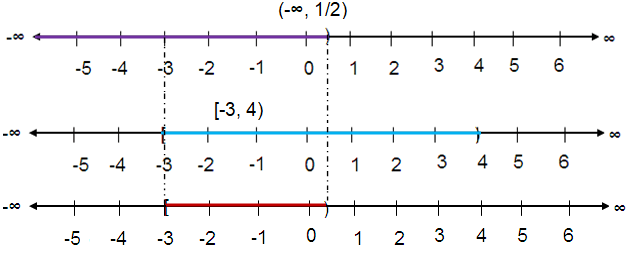
(-∞, 1/2) ∩ [-3, 4)
To find the intersection, graph each interval separately. Then find the real numbers common to both intervals.
Problem 2 :
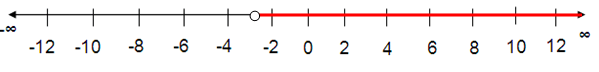
(-∞, -2) ∪ [-4, 3)

Solve the compound inequalities . Write the solutions in interval notation.
Problem 1 :
4m > -11 and 4m – 3 ≤ 13
Solution :
4m > -11
Divide by 4 on both sides.
4/4m > -11/4
m > -11/4
By graphing the inequality m > -11/4, we get the graph given below.
4m – 3 ≤ 13
Add 3 on both sides.
4m – 3 + 3 ≤ 13 + 3
4m ≤ 16
Divide by 4 on both sides.
4m/4 ≤ 16/4
m ≤ 4

By combining the above two graphs, we get the common region between -11/4 and 4.
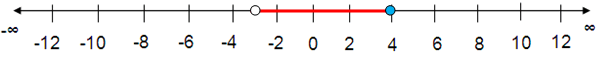
Problem 2 :
4n – 7 < 1 and 7 + 3n ≥ -8
Solution :
4n – 7 < 1
Add 7 on both sides.
4n – 7 + 7 < 1 + 7
4n < 8
Divide by 4 on both sides.
4n/4 < 8/4
n < 2
7 + 3n ≥ -8
Subtract by 7 on both sides.
7 + 3n - 7 ≥ -8 – 7
3n ≥ -15
Divide by 3 on both sides.
3/3n ≥ -15/3
n ≥ -5
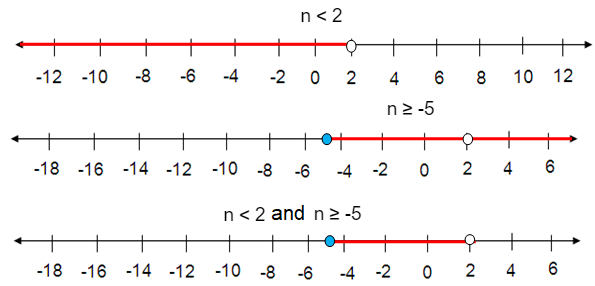
Problem 3 :
-3y + 1 ≥ 10 and -2y – 5 ≤ -15
Solution :
Solving -3y + 1 ≥ 10 :
-3y + 1 ≥ 10
Subtract by 1 on both sides.
-3y + 1 - 1 ≥ 10 – 1
-3y ≥ 9
Divide by -3 on both sides.
y ≤ -3
Solving -2y – 5 ≤ -15 :
-2y – 5 ≤ -15
Add 5 on both sides.
-2y – 5 + 5 ≤ -15 + 5
-2y ≤ -10
Divide by -2 on both sides.
y ≥ 5
It should satisfy the conditions y ≤ -3 and y ≥ 5. No such values in the number line. So, there is no solution.
Problem 4 :
(1/2) – (h/12) ≤ -7/12 and (1/2) - (h/10) > -1/5
Solution :
Solving 1/2 - h/10 > -1/5 :
1/2 - h/10 > -1/5
Subtract by 1/2 on both sides.
-h/10 > -1/5 – 1/2
-h/10 > -1/5 × (2/2) – 1/2 × (5/5)
-h/10 > -2/10 – 5/10
-h/10 > -7/10
Multiply 10 on each sides.
(-h/10) × 10 > (-7/10) × 10
-h > -7
h < 7
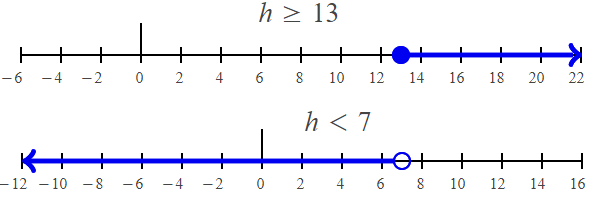
So, no solution.
Problem 5 :
(2/3)t – 3 ≤ 1 or (3/4)t – 2 > 7
Solution :
Solving (2/3)t – 3 ≤ 1 :
2t – 9 ≤ 3
Add 9 on both sides.
2t – 9 + 9 ≤ 3 + 9
2t ≤ 12
Divide by 2 on both sides.
2/2t ≤ 12/2
t ≤ 6
Solving for (3/4)t – 2 > 7 :
(3/4)t – 2 > 7
3t – 8 > 28
Add 8 on both sides.
3t – 8 + 8 > 28 + 8
3t < 36
Divide by 3 on both sides.
3/3t < 36/3
t < 12
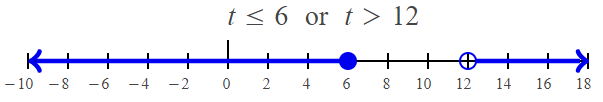
Problem 6 :
2(3x + 1) < -10 or 3(2x – 4) ≥ 0
Solution :
Solving 2(3x + 1) < -10 :
2(3x + 1) < -10
6x + 2 < -10
Subtract by 2 on both sides.
6x + 2 - 2 < -10 – 2
6x < -12
Divide by 6 on both sides.
6x/6 < -12/6
x < -2
Solving 3(2x – 4) ≥ 0 :
3(2x – 4) ≥ 0
6x – 12 ≥ 0
Add 12 on both sides.
6x ≥ 12
Divide by 6 on both sides.
6x/6 ≥ 12/6
x ≥ 2
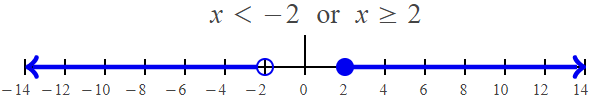
Problem 7 :
-7 < -7(2w + 3) or -2 < -4(3w – 1)
Solution :
Solving -7 < -7(2w + 3) :
-7 < -7(2w + 3)
Multiplying by negative -1, we get
1 > (2w + 3)
Subtracting 3
1 - 3 > 2w
-2 > 2w
-1 > w
Solving -2 < -4(3w – 1) :
-2 < -4(3w – 1)
Dividing by -2, we get
1 > 2(3w - 1)
1/2 > 3w - 1
Add 1, we get
1/2 + 1 > 3w
3/2 > 3w
1/2 > w
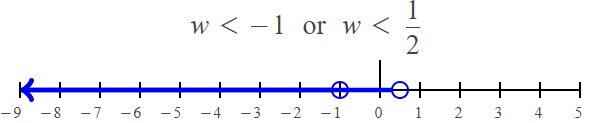
Problem 8 :
5(p + 3) + 4 > p -1 or 4(p – 1) + 2 > p + 8
Solution :
Solving 5(p + 3) + 4 > p -1 :
5(p + 3) + 4 > p -1
5p + 15 + 4 > p -1
5p + 19 > p – 1
Subtract by 19 on both sides.
5p > p – 1 – 19
5p > p – 20
Subtract by p on both sides.
4p > -20
Divide by 4 on both sides.
4p/4 > -20/4
p > -5
Solving 4(p – 1) + 2 > p + 8 :
4(p – 1) + 2 > p + 8
4p – 4 + 2 > p + 8
4p - 2 > p + 8
Subtract by p on both sides.
4p – p – 2 > 8
3p – 2 > 8
Add 2 on both sides.
3p > 8 + 2
3p > 10
Divide by 3 on both sides.
3p/p > 10/3
p > 10/3
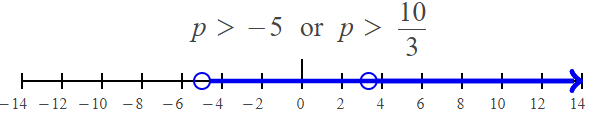
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling