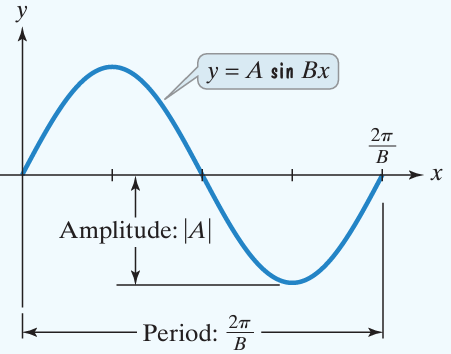
SKETCHING THE GRAPH OF Y EQUAL A SIN BX

To learn how to sketch the graph of y = A sin Bx, we have to understand the meaning of the terms involving this topic.

• The amplitude is the vertical distance between a maximum or minimum point of the principal axis. From the given equation, A is amplitude.
• The horizontal length of one cycle of the graph is known as period. To calculate period, we will use the formula 2𝜋/|B|
• For the function in the form y = A sin Bx, there is no horizontal or vertical shift. 

In general for the function y = sin x.

• Amplitude is 1 and period is 2𝜋. 
• the graph will start with (0, 0).
• To get the next inputs, we will add the preceding inputs by the value of period / 4

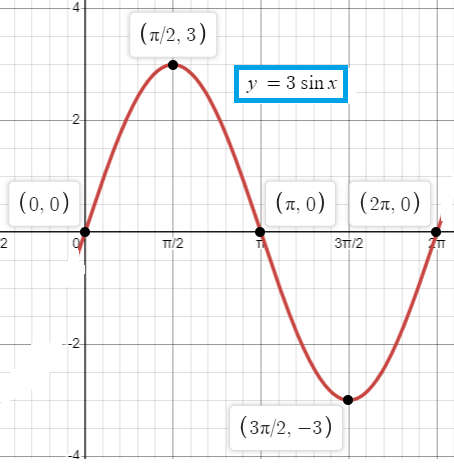
Determine the amplitude, period, phase shift of each function. Then graph of one period of the function.

Problem 1 :

y = 3 sin x

Solution :

Comparing the given function with y = A sin Bx

y = 3 sin x

A = 3, B = 1

Finding amplitude :

Amplitude = 1

Finding period :

Period = 2𝜋/|B|

= 2𝜋/1

= 2𝜋

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= 2𝜋/4

= 𝜋/2

Calculating Inputs and Outputs :

So, the points are (0, 0), (𝜋/2, 3) (𝜋, 0) (3𝜋/2, -3) and (2𝜋, 0)

Problem 2 :

y = (1/3) sin x

Solution :

Comparing the given function with y = A sin Bx

y = (1/3) sin x

A = 1/3, B = 1

Finding amplitude :

Amplitude = 1/3

Finding period :

Period = 2𝜋/|B|

= 2𝜋/1

= 2𝜋

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= 2𝜋/4

= 𝜋/2

Calculating Inputs and Outputs :

So, the points are (0, 0), (𝜋/2, 1/3) (𝜋, 0) (3𝜋/2, -1/3) and (2𝜋, 0)

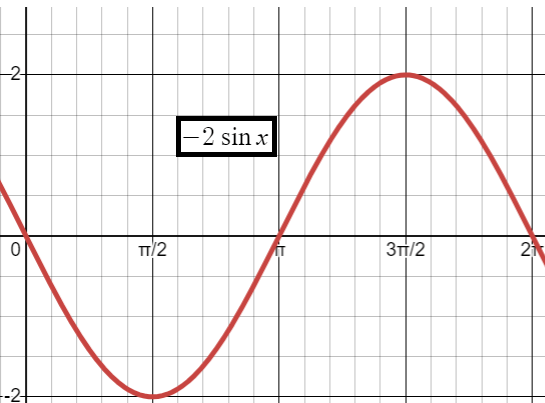
Problem 3 :

y = -2 sin x

Solution :

Comparing the given function with y = A sin Bx

y = -2 sin x

A = 2, B = 1

Finding amplitude :

Amplitude = 2

Since we have negative there should be reflection across y-axis.

Finding period :

Period = 2𝜋/|B|

= 2𝜋/1

= 2𝜋

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= 2𝜋/4

= 𝜋/2

Calculating Inputs and Outputs :

So, the points are (0, 0), (𝜋/2, 2) (𝜋, 0) (3𝜋/2, -2) and (2𝜋, 0)

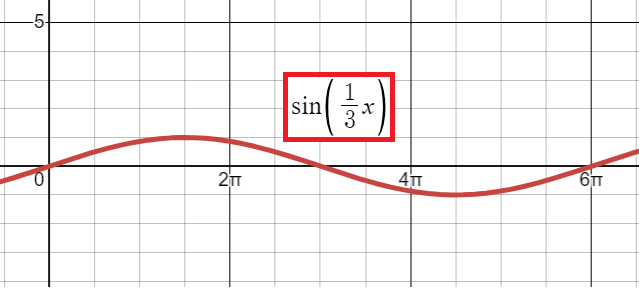
Problem 4 :

y = sin (1/3)x

Solution :

Comparing the given function with y = A sin Bx

y = sin (1/3)x

A = 1, B = 1/3

Finding amplitude :

Amplitude = 1

Finding period :

Period = 2𝜋/|B|

= 2𝜋/(1/3)

= 6𝜋

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= 6𝜋/4

= 3𝜋/2

Calculating Inputs and Outputs :

So, the points are (0, 0), (3𝜋/2, 1) (3𝜋, 0) (9𝜋/2, -1) and (6𝜋, 0)

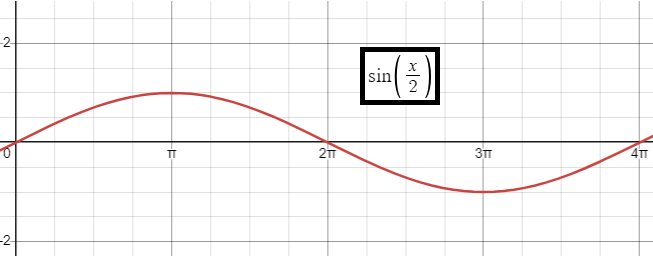
Problem 5 :

y = sin (x/2)

Solution :

Comparing the given function with y = A sin Bx 

y = sin (x/2)

A = 1, B = 1/2

Finding amplitude :

Amplitude = 1

Finding period :

Period = 2𝜋/|B|

= 2𝜋/(1/2)

= 4𝜋

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= 4𝜋/4

= 𝜋

Calculating Inputs and Outputs :

So, the points are (0, 0), (𝜋, 1) (2𝜋, 0) (3𝜋, -1) and (4𝜋, 0)

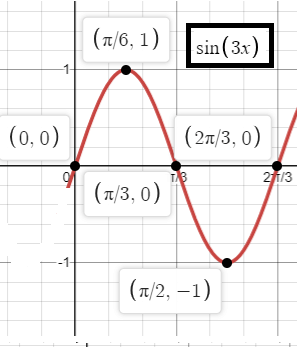
Problem 6 :

y = sin 3x

Solution :

Comparing the given function with y = A sin Bx 

y = sin (3x)

A = 1, B = 3

Finding amplitude :

Amplitude = 1

Finding period :

Period = 2𝜋/|B|

= 2𝜋/3

Finding x-coordinates of key points :

Each input should add upto = Period / 4

= (2𝜋/3)/4

= 𝜋/6

Calculating Inputs and Outputs :

So, the points are (0, 0), (𝜋/6, 1) (𝜋/3, 0) (𝜋/2, -1) and (2𝜋/3, 0)