RADIAN AND MEASURE OF ANGLE SAT PRACTICE QUESTIONS

Problem 1 :

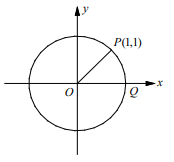
In the xy- plane above, O is the center of the circle, and the measure of ∠POQ is kπ radians. What is the value of k ?

a)  1/6    b)  1/4     c)  1/3     d)  1/2

Solution :

∠POQ = kπ

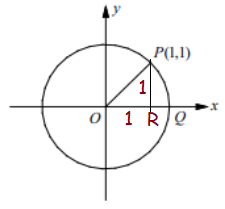tan ∠POQ = PR/OR = 1/1 = tan 45 45 = π/4 kπ = π/4 k = 1/4

So, the value of k is 1/4.

Problem 2 :

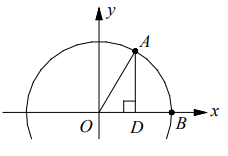
In the xy- plane above, O is the center of the circle and the measure of ∠AOD is π/3 . If the radius of circle O is 6 what is the length of AD ?

a)  3      b)  3√2      c)  4.5    d) 3√3

Solution :

∠AOD = 3 π

Radius of the circle (OA) = 6

sin ∠AOD = Opposite side / Hypotenuse

sin ∠AOD = AD/OA

sin (π/3) = AD/6

√3/2 = AD/6

AD = 6(√3/2)

= 3√3

So, option d is correct.

Problem 3 :

Which of the following is equal to cos (π/8)

A)  cos 3π/8     b)  cos 7π/8     c)  sin (3π/8)

d)  sin (7π/8)

Solution :

sin θ = cos (90 - θ)

cos θ = sin (90 - θ)

cos (π/8) = sin ((π/2) - π/8)

= sin (4π - π)/8)

= sin (3π/8)

So, option c is correct.

Problem 4 :

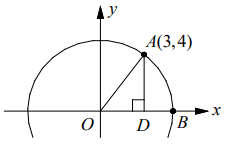
In the figure above, what is the value of cos∠AOD ?

a) 3/5    b) 3/4    c) 4/5     D) 4/3

Solution :

cos∠AOD = Adjacent side / Hypotenuse

cos∠AOD = OD/OA

From the given triangle,

OD = 3, AD = 4

Using Pythagorean theorem :

OA² = OD² + DA²

OA² = 3² + 4²

 = 9 + 16

OA² = 25

OA = 5

cos ∠AOD = 3/5

Problem 5 :

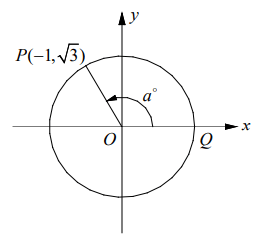
In the xy- plane above, O is the center of the circle, and the measure of ∠POQ is a°.

What is the cosine of a° ?

a)  -1/2    b)  √3      c)  -1/√3     d)  √3/2

Solution :

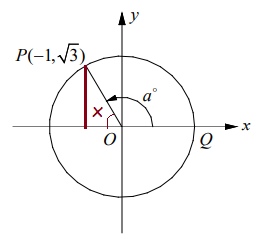
tan θ = Opposite side / Adjacent side

Opposite side = 1,  Adjacent side = √3

tan θ = 1/√3

θ = π/3

a = π - (π/3)

a = (3π - π)/3

a = 2π/3

Evaluating the value of cosine of a° :

cos (2π/3)

= cos (π/2 + π/3)

= sin (π/3)

= √3/2

So, option d is correct.

Problem 6 :

In the xy- plane above, O is the center of the circle, and the measure of the angle shown is kπ radians.

Solution :

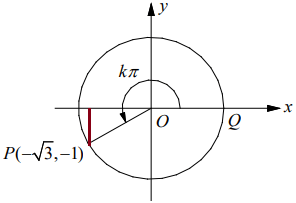
In the triangle above, 

tanθ = Opposite side ./ adjacent side

tanθ = √3/1

tanθ = √3

θ = π/3

k π = π/3

k π = (1/3) π

k = 1/3

So, the value of k is 1/3.

Problem 7 :

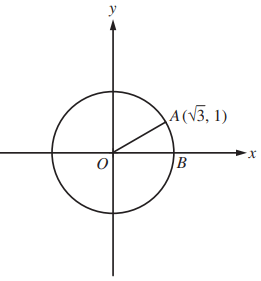
In the xy-plane above, O is the center of the circle, and the measure of ∠AOB is π/a radians. What is the value of a ?

Solution :

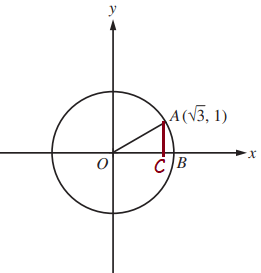
tanθ = Opposite side / adjacent side

Opposite side = √3, adjacent side = 1

tan ∠AOB = √3 / 1

tan ∠AOB = √3

For what angle measure of tangent, we get √3

For tan π/3, we get √3

π/a = π/3

Comparing the corresponding terms, we get a = 3.

Problem 8 :

In the circle above, point A is the center and the length of arc BC is 2/5 of the circumference of the circle. What is the value of x ?

Solution :

Length of arc BC = (2/5) x circumference of circle

= (2/5) x 360

x = 144

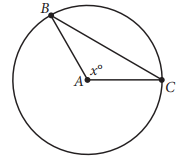
Problem 9 :

In the figure above, triangle ABC has an area of 19. What is the value of tan θ?

Solution :

Area of triangle ABC = 19

Area of triangle ABD + Area of triangle BDC = 19 ----(1)

In triangle ABD, using Pythagorean theorem :

AB² = AD² + DB²

5² = AD² + 4²

25 - 16 = AD²

 AD² = 9

AD = 3

(1/2) x AD x BD + (1/2) x DC x BD = 19

1/2 x BD [AD + DC] = 19

1/2 x 4 x [3 + DC] = 19

[3 + DC] = 19/2

DC = (19/2) - 3

DC = (19 - 6)/2

DC = 13/2

tan θ = Opposite side / Adjacent side

tan θ = BD / DC

tan θ = 4 / (13/2)

tan θ = 8 / 13

Problem 10 :

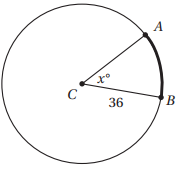
In the circle above, arc AB has a measure of 7π. What is the value of x?

Solution :

Length of arc AB = (θ/360) x 2πr

= (x/360) x 2π(36)

= (x/5) x π

Given that, Length of arc AB = 7π

(x/5) x π = 7π

x = 35