QUIZ ON PROPERTIES OF QUADRILATERALS

Part A

Answer the following with the response of True or False:

1) A trapezoid has one pair of parallel sides. ____________________

True

2) In an isosceles trapezoid, the non-parallel sides are congruent. ____________________

True

3) In a rhombus, all sides are congruent. ____________________

True

4) In a rectangle, diagonals are perpendicular. ____________________

False

In square and in rhombus, the diagonals are perpendicular. For Parallelogram, rectangle, and trapeziums, the diagonals are not perpendicular.

5) In an isosceles trapezoid, opposite angles are congruent. ____________________

False

The opposite angles are supplementary.

6) All quadrilaterals are rectangles. ____________________

False

Rectangle can be considered as quadrilateral but all quadrilateral not not rectangles.

Part B

Problem 1 :

Which of the following is true about every parallelogram ?

a) All four sides are congruent

b) The diagonals are perpendicular to each other.

c) Two pairs of opposite angles are congruent

d) The consecutive angles are congruent.

Solution :

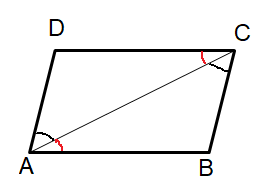
In parallelogram, opposite sides are parallel and equal.

In triangle ADC and in ABC,

∠DCA = ∠BAC (DC || AB, alternate interior angles)

∠DAC = ∠BCA  (AD || BC, alternate interior angles)

AC = AC (Common)

So, triangles ADC and ABC are congruent. Using CPCT 

∠D = ∠B

Opposite angles are equal.

Problem 2 :

Which reason can be used to prove that a parallelogram is a rhombus ?

a) The diagonals are congruent

b) The opposite angles are congruent

c) The diagonals are perpendicular

d) The opposite sides are parallel.

Solution :

In rhombus, the diagonal will be perpendicular. But in parallelogram the diagonals will not be perpendicular.

Problem 3 :

For which quadrilateral are the diagonals congruent but do not bisect each other ?

a) Parallelogram

b) Isosceles trapezoid

c) Rectangle 

d) Rhombus

Solution :

In isosceles trapezoid, the diagonals are congruent but they will not bisect each other.

Problem 4 :

For which quadrilaterals are the diagonals congruent ?

a) Rhombus   b)  Parallelogram  c) Isosceles trapezoid

d) Rectangle      e) Square

Solution :

In  Isosceles trapezoid, Rectangle and Square the diagonals are congruent.

Problem 5 :

If the diagonals of a quadrilateral do not bisect each other, then the quadrilateral could be a

a) Trapezoid   b) Square   c) Rectangle   d) Rhombus

Solution :

In trapezoid, the diagonals do not bisect each other.