PROPERTIES OF A RECTANGLE

Rectangle

Definition of rectangle :

A rectangle is a parallelogram with four equal angles of 90 degree.

Properties of rectangle :

• Opposite sides are equal and parallel.
• Diagonals bisect each other.
• Diagonals are equal in length.

Problem 1 :

In the given rectangle, find ∠1 and ∠2.

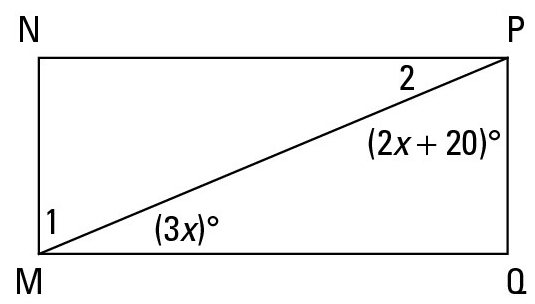
Solution :

∠PMQ + ∠MQP + ∠QPM = 180

3x + 90 + 2x + 20 = 180

5x + 110 = 180

Subtracting 110 on both sides.

5x = 180 - 110

5x = 70

Dividing by 5 on both sides.

x = 70/5

x = 14

Problem 2 :

Find x.

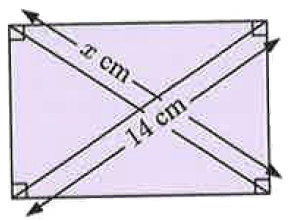
Solution :

Since the diagonals are equal, the value of x is 14 cm.

Problem 3 :

In the rectangle given below, find the value of x.

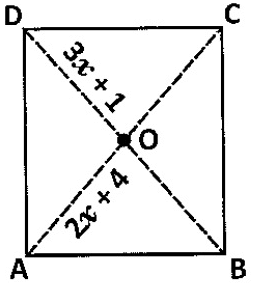
Solution :

Since the diagonals are equal and bisect each other,

OA = OD

OA is half of diagonal AC and OD is half of the diagonal of BD.

2x + 4 = 3x + 1

Subtracting 3x and 4 on both sides.

2x - 3x = 1 - 4

-x = -3

x = 3

Problem 4 :

In the rectangle given below, the length of the diagonal is 36. Find values of x and y.

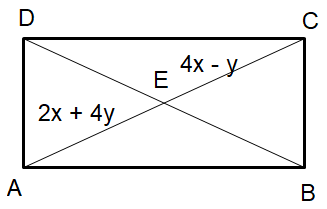
Solution :

Length of diagonal = 36

2x + 4y + 4x - y = 36

6x + 3y = 36

Dividing by 2, we get

2x + y = 12 ----(1)

Length of diagonal will be equal.

2x + 4y = 4x - y

2x - 4x + 4y + y = 0

-2x + 5y = 0 ----(2)

(1) + (2)

2x - 2x + y + 5y = 12 + 0

6y = 12

y = 12/6

y = 2

By applying the value of y in (1), we get

2x + 2 = 12

2x = 10

x = 10/2

x = 5

Problem 5 :

In rectangle ABCD, AC = x + 8, AB = x + 7 and AD = x. Find the value of x, also find AC, AB and AD.

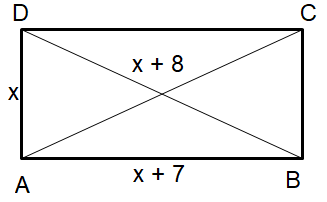
Solution :

In triangle ABC, angle B is 90 degree.

AC² = AB² + BC²

(x + 8)² = (x + 7)² + x²

x² + 16x + 64 = x² + 14x + 49 + x²

2x² - x² + 14x - 16x + 49 - 64 = 0

x² - 2x - 15 = 0

(x - 5) (x + 3) = 0

x = 5 and x = -3

So, AB = 12, AC = 13 and AD = 5.