PROBLEMS ON ZEROS OF POLYNOMIAL

Problem 1 :

The value of k for which -4 is a zero of the polynomial

x² - x - (2k + 2) is

a)   3     b) 9     c) 6     d) -1

Solution :

x² - x - (2k + 2)

As (-4) is zero of above polynomial. So,

(-4)² - (-4) - (2k + 2) = 0

16 + 4 - 2k -2 = 0

20 - 2k - 2 = 0

-2k = -18

k = 18/2

k = 9

So, option (b) is correct.

Problem 2 :

If the zeros of the quadratic polynomial

ax² + bx + c, c ≠ 0

are equal, then

a. c and a have opposite sign

b. c and b have opposite sign

c. c and a have same sign

d. c and b have same sign

Solution :

Given that, the zeros of the quadratic polynomial

ax² + bx + c, c ≠ 0 are equal (D) = 0

Discriminant

b² - 4ac = 0

b² = 4ac

Which is only possible when a and c have the same sign.

So, option (c) is correct.

Problem 3 :

The number of zeros of the polynomial from the graph is

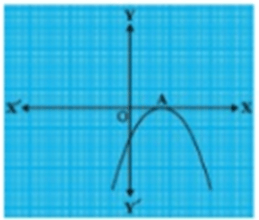
a)   0     b) 1     c) 2     d) 3

Solution :

The curve does not cross x-axis, it touches the x-axis. So, it has two zeroes but both are same.

So, option (c) is correct.

Problem 4 :

If one of the zero of the quadratic polynomial

x² + 3x + k is 2

then the value of k is

a)   10     b) -10     c) 2     d) 3

Solution :

p(x) = x² + 3x + k

2 is a zero of p(x), then

p(2) = 0

2² + 3(2) + k = 0

4 + 6 + k = 0

10 + k = 0

k = -10

So, option (b) is correct.

Problem 5 :

A quadratic polynomial whose zeros are -3 and 4 is

a)   x²  - x + 12     b) x² + x + 12

c) 2x² + 2x - 24     d) none of the above

Solution :

Quadratic equation with α and β as roots can be written as

x² - (α + β) + αβ = 0

Here, α = -3 and β = 4

α + β = -3 + 4 = 1

α × β = -3 × 4 = -12

The quadratic equation is

x² - (α + β) + αβ = 0

x² - x - 12 = 0

p(x) = x² - x - 12

So, option (d) is correct.

Problem 6 :

The relationship between the zeros and coefficients of the quadratic polynomial

ax² + bx + c is

a)   α + β = c/a     b) α + β = -b/a

c) α + β = -c/a     d) α + β = b/a

Solution:

The relationship between the zeros and the sum of the zeros is given by

Sum of zeros (α + β) = -(Coefficient of x) / Coefficient of x²

α + β = -b/a

So, option (b) is correct.

Problem 7 :

The zeros of the polynomial

x² + 7x + 10 are

a)   2 and 5     b) -2 and 5     c) -2 and -5     d) 2 and -5

Solution :

x² + 7x + 10 = 0

x² + 5x + 2x + 10 = 0

x(x + 5) + 2(x + 5) = 0

(x + 2) (x + 5) = 0

x = -2 or x = -5

So, option (c) is correct.

Problem 8 :

The relationship between the zeros and coefficients of the quadratic polynomial ax² + bx + c is

a)   α · β = c/a     b) α · β = -b/a

c) α · β = -c/a     d) α · β = b/a

Solution :

The relationship between the zeros and the product of the zeros is given by

Product of zeros (α · β) = constant term / Coefficient of x²

α · β = c/a

So, option (a) is correct.

Problem 9 :

The zeros of the polynomial x² - 3 are

a)   2 and 5     b) -2 and 5

c) -2 and -5     d) none of the above

Solution :

x² - 3 = 0

x² = 3

x = ± √3

Therefore, the zeros of the given polynomial are √3 and -√3.

So, option (d) is correct.

Problem 10:

The number of zeros of the polynomial from the graph is

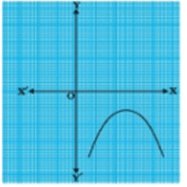
a)   0     b) 1     c) 2     d) 3

Solution :

The zeros of the polynomial are the points where the graph intersects the x axis. In the given graph, the curve intersects the x axis at 0 point, therefore, the number of zeros of the polynomial is 0.

So, option (a) is correct.

Problem 11 :

A quadratic polynomial whose sum and product of zeros are -3 and 2 is

a)   x²  - 3x + 2     b) x² + 3x + 2

c) x² + 2x - 3     d) x² + 2x + 3

Solution :

Let the zeros be α and β.

α + β = -3 and αβ = 2

The quadratic polynomial whose sum and product of the zeros are given is

x² - (α + β)x + αβ

Then the quadratic polynomial will be

= x² - (-3)x + 2

= x² + 3x + 2

So, option (b) is correct.

Problem 12 :

The zeros of the quadratic polynomial x² + kx + k, k ≠ 0,

a. Cannot both be positive

b. Cannot both be negative

c. Are always unequal

d. Are always equal

Solution :

We know that, the zeros of a polynomial ax² + bx + c

Sum of zeros = -b/a

Product of zeros = c/a

Here a = 1, b = k, c = k

Sum of the roots = -b/a

= -k/1

= -k

Product of the roots = c/a

= k/1

= k

If k is negative :

Sum is positive, product is negative.

One zero will be positive, one will be negative.

If k is positive :

Sum is negative, product is positive.

So, in both cases, both zeros cannot be positive.

So, both zeros are negative.

So, option (a) is correct.