PROBLEMS ON TRIGONOMETRY FOR SAT

Problem 1 :

sin(x) = cos(K − x)

In the equation above, the angle measures are in radians and K is a constant. Which of the following could be the value of K?

A) 0      B) π/4      C) π/2       D) π

Solution :

Trigonometry angles of complementary angles :

sin(x) = cos(K − x)

So, the value of K is π/2.

Problem 2 :

In a right triangle, one angle measures x°, where

sin x° = 4/5

What is cos(90° − x°) ?

Solution :

sin x° = 4/5

Using the formula of complementary angles involving trigonometry, it is clear 

sin x = cos (90 - x)

cos (90 - x) = 4/5

Problem 3 :

In a circle with center O, central angle AOB has a measure of 5π/4 radians. The area of the sector formed by central angle AOB is what fraction of the area of the circle?

Solution :

Area of circle = πr²

Converting the radian into degree,

= 5π/4 x (180/π)

= 225°

Area of sector = (θ/360) πr²

= (225/360) πr²

= (5/8)πr²

So, area of the sector is 5/8 of area of circle.

Problem 4 :

In the -xy plane below, O is the center of the circle, and the measure of ∠POQ is kπ radians. What is the value of k?

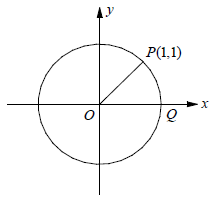
Solution :

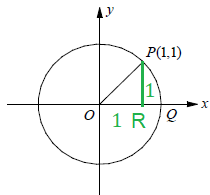
tan θ = opposite side / adjacent side

tan kπ = 1/1

tan kπ = 1

tan kπ = tan (π/4)

k = 1/4

Problem 5 :

In the -xy plane below, O is the center of the circle and the measure of ∠AOD is π/3. If the radius of circle O is 6 what is the length of AD?

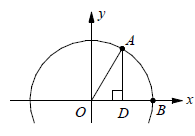
Solution :

∠AOD = π/3 = 60°

In triangle AOD,

OA = Hypotenuse = radius = 6 cm

OD = Smaller side and AD = Opposite to 60°

sin θ = opposite side / hypotenuse

sin θ = AD / OA

sin 60° = AD / 6

√3/2 = AD / 6

AD = (6√3/2)

AD = 3√3

Problem 6 :

Which of the following is equal to cos(π/8) ?

A)  cos 3π/8       B)  cos 7π/8

C)  sin 3π/8        D)  sin 7π/8

Solution :

Using complementary angles in trigonometry,

cos θ = sin (90 - θ)

cos(π/8) = sin ((π/2) - (π/8))

= sin (4π-π)/8

= sin 3π/8

Problem 7 :

In the figure below, what is the value of cos ∠AOD?

A) 3/5        B) 3/4     C) 4/5     D) 4/3

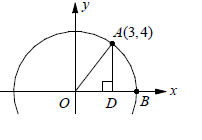
Solution :

Let ∠AOD = θ

cos θ = Adjacent side / hypotenuse = OD/OA ----(1) 

OD = Adjacent side = 3

AD = Opposite side = 4

OA = √OD² + AD²

OA = √3² + 4²

OA = √(9+16)

OA = √25

OA = 5

cos θ = 3/5 

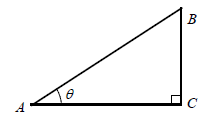
Problem 8 :

In the right triangle shown below, if tan θ = 3/4, what is sin θ?

A)  1/3     B)  1/2       C)  4/5     D) 3/5

Solution :

tan θ = 3/4 = Opposite side / adjacent side

Hypotenuse(AB) = √3² + 4²

AB = 5

sin θ = Opposite side / hypotenuse

sin θ = 3/5

Problem 9 :

In the xy-plane below, O is the center of the circle and the measure of ∠POQ is k π radians.

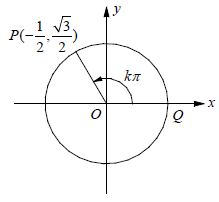
What is the value of k ?

A)  1/3    B) 1/2     C)  2/3     D)  3/4

Solution :

∠POR = θ

tan θ = opposite side / adjacent side 

tan θ = (1/2) / (√3/2)

tan θ = 1/√3

θ = π/3

π/3 + k π = π

k π = π - (π/3)

k π = 2π/3

k = 2/3

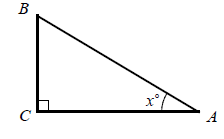
Problem 10 :

In the right triangle ABC below, the cosine of x° is 3/5. If BC = 12, what is the length of AC?

Solution :

cos x = 3/5 = Adjacent side / Hypotenuse = AC/AB

Adjacent side(AC) = 3a and hypotenuse (AB) = 5a

BC = 12

Opposite side (BC) = √(5a)² - (3a)²

= √25a² - 9a²

= √16a²

Opposite side (BC) = 4a

12 = 4a

a = 3

Adjacent side = 3a ==> 3(3) ==> 9