PROBLEMS ON COMPLEMENTARY AND SUPPLEMENTARY ANGLES

Find the values of unknowns :

Problem 1 :

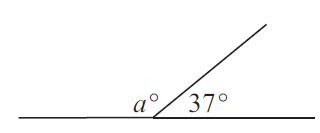
Solution :

In the picture the above angles are linear pairs.

a + 37 = 180

a = 180 - 37

a = 143

Problem 2 :

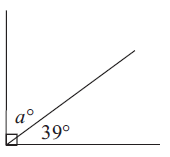
Solution :

Since the angles a and 39 are complementary, sum of those angles will be 90°.

a + 39 = 90

a = 90 - 39

a = 51°

Problem 3 :

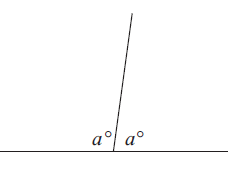
Solution :

In the picture the above angles are linear pairs.

a + a = 180

2a = 180

a = 180/2

a = 90

Problem 4 :

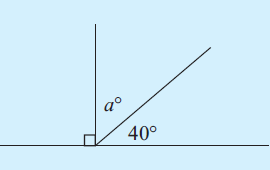
Solution :

From the picture, it is clear

90 + a + 40 = 180

130 + a = 180

a = 180 - 130

a = 50

Problem 5 :

Solution :

h and 2h are supplementary.

h + 2h = 180

3h = 180

Divide by 3, we get

h = 180/3

h = 60

Problem 6 :

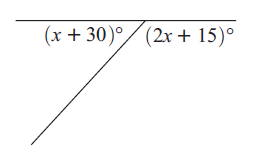
Solution :

x + 30 and 2x + 15 are supplementary.

x + 30 + 2x + 15 = 180

3x + 45 = 180

Subtracting 45

3x = 180 - 45

3x = 135

Divide by 3

x = 135/3

x = 45

Problem 7 :

Solution :

2x + 5 and 15x are complementary.

2x + 5 + 15x = 90

17x + 5 = 90

Subtracting 5 on both sides.

17x = 90 - 5

17x = 85

Dividing by 17 on both sides.

x = 85/17

x = 5

Problem 8 :

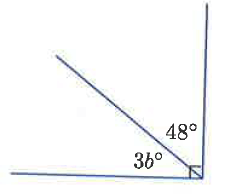
Solution :

3b and 48 are complementary.

3b + 48 = 90

Subtracting 48 on both sides.

3b = 90 - 48

3b = 42

Dividing by 3, we get

b = 42/3

b = 14

Problem 9 :

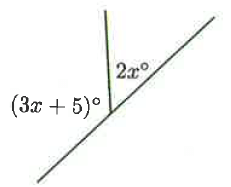
Solution :

3x + 5 and 2x are supplementary.

3x + 5 + 2x = 180

5x + 5 = 180

Subtracting 5 on both sides.

5x = 180 - 5

5x = 175

Divide by 5 on both sides.

x = 175/5

x = 35

Problem 10 :

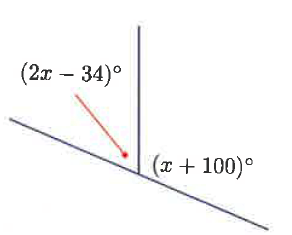
Solution :

2x - 34 and x + 100 are supplementary.

2x - 34 + x + 100 = 180

3x + 66 = 180

Subtracting 66 on both sides.

3x = 180 - 66

3x = 114

Dividing by 3 on both sides.

x = 114/3

x = 38