PROBLEMS ON BEARING AND TRIGONOMETRY

Problem 1 :

The angle of depression from a kookaburra’s feet to a worm on a ground is 40°.The worm is 15 meters from a point on the ground directly below the kookaburra's feet.

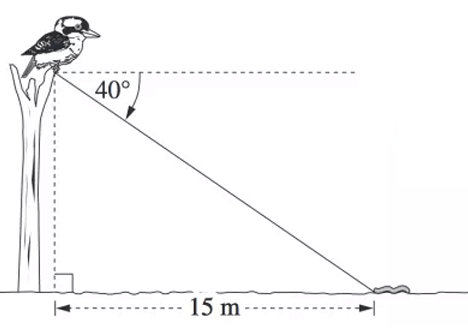
How high above the ground are the kookaburra's feet, correct to the nearest meter?

Solution :

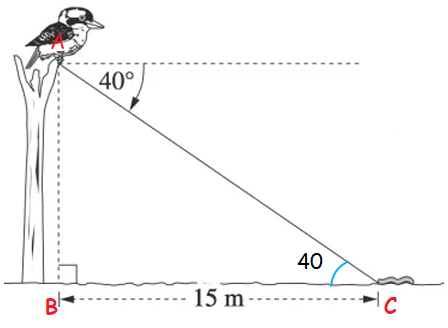
In triangle ABC,

∠BCA = 40

AB = Opposite side

AC = Hypotenuse

BC = Adjacent side = 15 m

tan θ = Opposite side / adjacent side

tan 40 = AB/BC

tan 40 = AB/15

AB = 15 tan 40

AB = 15 (0.839)

= 12.58 m

So, the kookaburra is approximately of 13 m height.

Problem 2 :

Danni is flying a kite that is attached to a string of length 80 meters. The string an angle of 55° with the horizontal. How high, to the nearest meter, is the kite above Danni's hand ?

Solution :

sin θ = Opposite side / hypotenuse

sin 55 = h / 80

0.819 = h/80

h = 0.819(80)

h = 65.52

So, the required height is approximately 66 m.

Problem 3 :

The plane flies on a bearing of 150° from A to B.

What is the bearing of A from B ?

Solution :

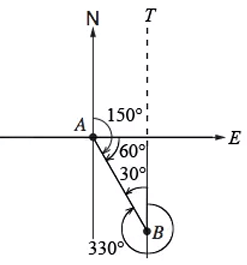
∠TBA = 30

Bearing of A from B = 330

Problem 4 :

Two trees on a ground level, 12 m apart are joined by a cable. It is attached 2 meters above the ground on one tree and 11 meters above the ground to the other.

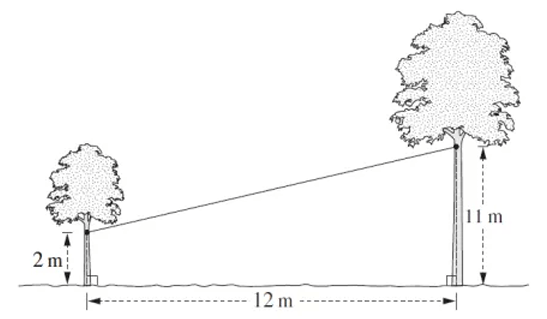
What is the length of the cable between the two trees ?

Solution :

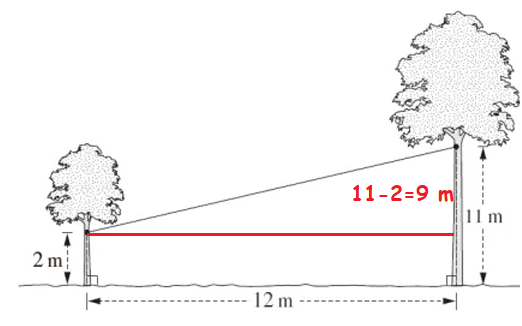
In the triangle shown above, using Pythagorean theorem

Let x be the unknown side.

x² = 12² + 9²

x² = 144 + 81

x² = 225

x = 15 m

So, the length of the cable is 15 m.

Problem 5 :

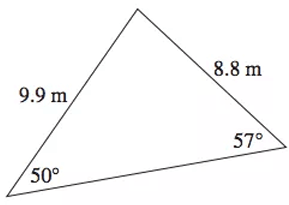
What is the area of the triangle ?

Solution :

To find area of the triangle shown above, we will use the formula

= 1/2 ab sin C

∠C = 180 - (50 + 57)

= 180 - 107

∠C = 73

a = 9.9 m and b = 8.8 m

Area of the triangle ABC = 1/2 x 9.9 x 8.8 sin 73

= 0.5 x 9.9 x 8.8 x 0.956

= 41.64 m²

Problem 6 :

Three towns P, Q and R are marked to the diagram. The distance from R to P is 76 km. ∠RQP = 26° and ∠RPQ = 46°

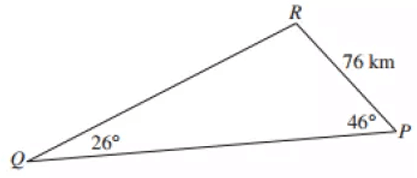
What is the distance between P to Q to the nearest km ?

Solution :

Using sin rule,

a/sin A = b/sin B = c/sin C

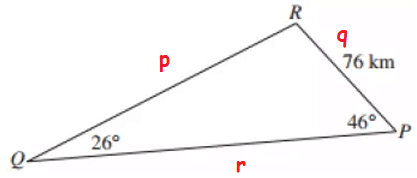
∠R = 180 - (∠Q + ∠P)

∠R = 180 - (26 + 46)

= 180 - 72

= 108

p/sin p = q/sin Q = r/sin R

p/sin 46 = 76/sin 26 = r/sin 108

76/sin 26 = r/sin 108

76/0.438 = r/0.951

r = (76/0.438) (0.951)

r = 165.01 km

So, the required distance between P and Q is 165.01 km.

Problem 7 :

Town B is 80 km due north of Town A and 59 km from Town C. Town A is 31 km from Town C.

What is the bearing of Town C from Town B ?

Solution :

We need to figure out angle measure B.

cos B = (a² + c² - b²)/2ac

a = 59 km, b = 31 km and c = 80 km

cos B = (59² + 80² - 31²)/2(59)(80)

= (3481 + 6400 - 961)/9558

= 8920 / 9558

B = cos^-1(0.933).

B = 21.05

Bearing of C from B = 180 - 21

= 159

So, the required bearing is 159.

Problem 8 :

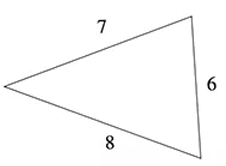
What is the size of the smallest angle in the triangle ?

Solution :

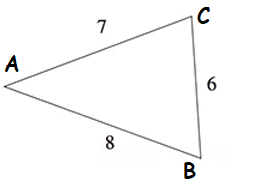
In geometry, the side which is opposite to the smallest angle measure is the smallest side.

∠A is the smallest angle.

cos A = (b² + c² - a²) / 2bc

a = 6, b = 7 and c = 8

cos A = (7² + 6² - 8²) / 2(6)(7)

= (49 + 36 - 64) / 84

= 21/84

A = cos ^-1(0.25)

A = 75.52

So, the required angle measure is 75.52.