PROBLEMS ON AREA OF SECTOR AND SEGMENT OF CIRCLE

Find the area of the shaded region. Leave your answer in terms of π and in simplest radical form.

Problem 1 :

Solution:

Problem 2 :

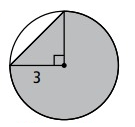
Solution:

Problem 3 :

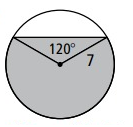
Solution:

Base of the triangle is  7√3 and height is 3.5

Area of triangle which is having central angle as 120 degree is

= (1/2) x  (7√3) x 3.5

= 12.25√3 ---(2)

(1) + (2)

= 98π/3 + 12.25√3

Find the area of each shaded segment. Round your answer to the nearest tenth.

Problem 4 :

Solution:

The diameter will divide the circle into two semicircles, from the picture it is clear, we have three sectors with same measure of central angle.

Area of triangle = (1/2) x 4 x2√3

= 6.928 --(2)

Required area = 8.37 - 6.928

= 1.442

= 1.4 mm²

Problem 5 :

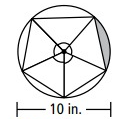
Solution:

Diameter = 10 in

radius = 10/2 = 5 in

Problem 6 :

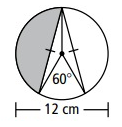
Solution:

60+150+150 ==> 360

The central angle of two equal arcs will be 150 degree.

Diameter = 12 cm

radius = 12/2 = 6 cm