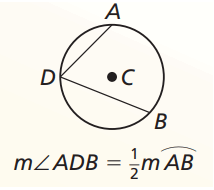
PROBLEMS ON ARC AND INSCRIBED ANGLE OF CIRCLES

What is inscribed angle ?

Angle whose vertex is on the circle ang whose sides are chords of a circle.

Intercepted arc ?

The arc that lies between two chords on the inscribed angle.

Measure of an inscribed angle is half of its intercepted arc.

Find the measure of angle indicated.

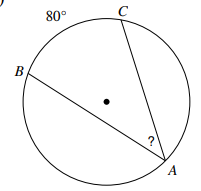
Problem 1 :

Find ∠BAC

Solution :

∠BAC is the angle created by the chords AC and AB.

∠BAC = (1/2) Measure of arc BC

∠BAC = (1/2) 80

∠BAC = 40

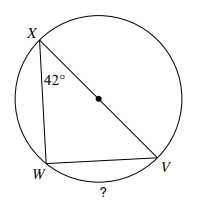
Problem 2 :

Find the measure of WV.

Solution :

∠WXV is the angle created by two chords XW and VX.

∠WXV = (1/2) Measure of arc WV

42 = (1/2) Measure of arc WV

Measure of arc WV = 42(2) ==> 84

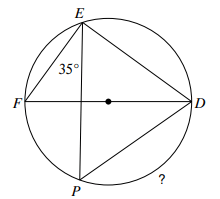
Problem 3 :

Find the measure of arc PD.

Solution :

∠FEP is the angle created between the chords EF and EP.

∠FEP = (1/2) Measure of arc FP

35 = (1/2)  Measure of arc FP

Measure of arc FP = 2(35)

Measure of arc FP = 70

Measure of arc FP + Measure of arc PD = 180

70 + Measure of arc PD = 180

Measure of arc PD = 180 - 70

Measure of arc PD = 110

Problem 4 :

Find x.

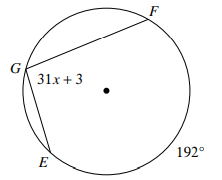
Solution :

∠EGF = (1/2) Measure of arc EF

31x + 3 = (1/2) (192)

31x + 3 = 96

31x = 96 - 3

31x = 93

x = 93/31

x = 3

Problem 5 :

Find the value of x.

Solution :

Measure of arc NM + Measure of arc LM = 180

7x - 10 + 13x - 10 = 180

20x - 20 = 180

20x = 180 + 20

20x = 200

x = 200/20

x = 10

Problem 6 :

In the diagram, AC = 12, CD = 3 and EC = 9

a) Find BC.

b) What is the measure of ∠ACB ?

c)  What is the measure of arc AE

d)  Is triangle ACB similar to triangle ECD

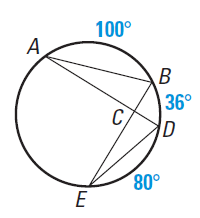
Solution :

Measure of arcs AE + ED + BD + AB = 360

Arc AE + 80 + 36 + 100 = 360

Arc AE = 360 - 216

Arc AE = 144

∠ABE = (1/2) measure of arc AE

∠ABE = (1/2) 144

∠ABE = 72

∠ABE  = ∠ADE 

∠BAD = ∠BED

∠ACB = ∠ECD

Then triangles ACB and ECD are congruent. So, 

AD = BE

AC + CD = BC + EC

12 + 3 = BC + 9

BC = 15 - 9

BC = 6

a) Find BC = 6

b) What is the measure of ∠ACB = 90 degree

c)  What is the measure of arc AE = 144

d)  Is triangle ACB similar to triangle ECD = yes