PROBLEMS ON ADJACENT ANGLES OF A PARALLELOGRAM WORKSHEET

Problem 1 :

If two adjacent angles of a parallelogram are (5x - 5)˚ and (10x + 35)˚, then the ratio of these angles is

a) 1: 3     b) 2: 3     c) 1: 4    d) 1: 2

Solution

Problem 2 :

The adjacent angles of a parallelogram are (2x - 4)˚ and (3x - 1)˚. Find the measures of all angles of the parallelogram.

Solution

Problem 3 :

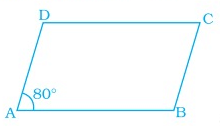
In parallelogram ABCD, find ∠B, ∠C and ∠D.

Solution

Problem 4 :

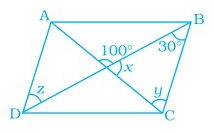
ABCD is a parallelogram. Find the value of x, y and z.

Solution

Problem 5 :

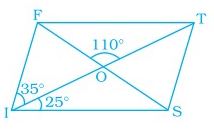
In parallelogram FIST, find ∠SFT, ∠OST and ∠STO.

Solution

Problem 6 :

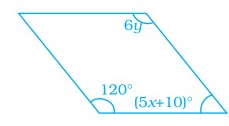
Find the values of x and y in the following parallelogram

Solution

Problem 1 :

From the parallelogram given below, find the value of x, y and z.

Problem 2 :

If one angle of a parallelogram is 24 degree less than twice the smallest angle then, find the largest angle of the parallelogram.

Problem 3 :

In a parallelogram PQRS, if

∠P = (3x − 5)° and ∠Q = (2x + 15)°

then find the value of x.

Problem 4 :

If PQRS is a parallelogram, then ∠P − ∠R is

(a) 90° (b) 45° (c) 60° (d) 0°

Problem 5 :

Two adjacent angles of a parallelogram are 3x - 4 and 3x + 10. Find the angles of parallelogram.

Problem 6 :

One angle of a parallelogram is 60 degree. Find its opposite angle and the adjacent angle.

Related Pages

• Properties of parallelogram worksheet
• Length of diagonal of a parallelogram
• Area of parallelogram
• Missing angles in parallelogram worksheet