PRACTICE QUESTIONS ON STATISTICS AND PROBABILITY FOR GRADE 8 EOG

Problem 1 :

At a particular company, every employee receives a 4% cost of living increase to their salary.

What impact does this cost living increase have on the mean and range of employee salaries at the company.

A)  The mean increases but the range does not change.

B)  The mean does not change but the range increases.

C)  The mean and range both increase.

D)  The mean and range do not change.

Solution :

Every employee is receiving 4% increase means in all values of their previous salary there will be a hike.

By finding mean, while there is increase in sum of salary the same change can be reflected in the mean also. 

Range is the difference between the largest and smallest value of the data. Comparing with the old salary, the new salary is increased 4%. Then new range will have also increase.

So, the mean and range both increase.

Problem 2 :

The frequency table below shows the age distribution of people at a park. 

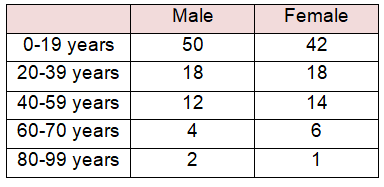
What is the probability a randomly selected person at a park is a female, given that the person is under 40 years old ?

A)   60/167       B) 15/32      C)  1/2       D)  60/81

Solution :

Number of persons under 40 :

= 50 + 42 + 18 + 18

= 128

Let A be the the required event.

Number of females at the park under the age of 40 = 42 + 18

= 60

P(A) = 60/128

= 15/32

So, option B is correct.

Problem 3 :

Twenty one students at a school have an allergy to peanuts, shellfish or both.

• Fourteen students at a school are allergic to peanuts.
• Twelve students at the school are allergic to shellfish.

How many students are allergic to both peanuts and shellfish ?

A)   12       B) 7      C)  5       D)  2

Solution :

Total number of students = 21

Number of student who are allergic to peanuts = 14

Number of students who are allergic to shellfish = 12

Total = 14 + 12

= 26

= 26  -21

= 5

So, 5 students are allergic to both.

Problem 4 :

There are 250 students in a senior class.

• Of the 250 students, 102 are boys.
• There are 20 senior girls and 18 senior boys on the track team

What is the probability a randomly chosen student from the senior class is a girl who does not run track ?

A)   0.920       B) 0.512      C)  0.497       D)  0.135

Solution :

Total number of students = 250

Number of boys = 102

Number of girls = 250 - 102

= 148

In 148 girls, 20 are running in the track. So, 128 are not running in the track. Let A be the event.

P(A) =128/250

= 0.512

So, option B is correct.

Problem 5 :

An elevator can hold a maximum of 1500 pounds. Eight people need to use the elevator. Bill had some measures from the data set how much each person weighed. Which measure would be most useful to determine if the people can safely use the elevator ?

A)   Mean       B) Median      C)  Mode

D)  Interquartile range

Solution :

Answer is option A, mean.

Problem 6 :

The table below shows the area of several states 

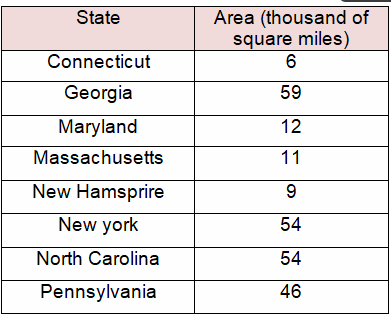
Delaware has an area of 2000 square miles. Which is true if Delaware is included in the data set ?

A) The mean increases        B)  The range decreases

C)  The interquartile range decreases.

D)  The standard deviation increases.

Solution :

In the table, we should include the last row as Dellaware = 2

Option A (Mean) : 2 in thousands = 2000 comparing the previous values in the table, this is not that much big. So, it will not affect mean that much.

Option B (Range) :

Range is the difference between the largest and smallest value, this change will not affect range.

Option C (Interquartile range) :

It will not affect.

Standard deviation will be affected. So, option D is correct.

Problem 7 :

The number of points scored by the basketball player in the first eight games of a season are shown below.

15, 35, 18, 30, 25, 21, 32, 16

What would happen to the data distribution if she scored 24, 22, 27 and 28 points in her four games ?

A) The data distribution would become less peaked and more widely spread.

B)  The data distribution would become less peaked and less widely spread.

C)  The data distribution would become more peaked and less widely spread.

D)  The data distribution would become more peaked and more widely spread.

Solution :

Given data :

15, 35, 18, 30, 25, 21, 32, 16

Values to be included :

24, 22, 27, 28

Including the values and arranging from least to greatest, we get

15, 16, 18, 21, 22, 24, 25, 27, 28, 30, 32, 35

By converting it as graphical representation, The data distribution would become more peaked and less widely spread, option C is correct.

Problem 8 :

A book club has 200 members. Each member was asked whether he or she prefers fiction or nonfiction books. The results are shown in the relative frequency table below.

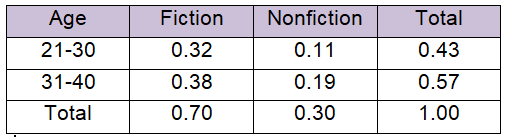
Which statement is true ?

A)  6 more 31-40 years old than 21-30 years olds prefer fiction.

B) 38 members are 31-40 and prefer fiction.

C)  43 members are 21-30 years old.

D)  140 members prefer fiction.

Solution :

This data is provided for 200 students. Multiplying each value by 200, we get

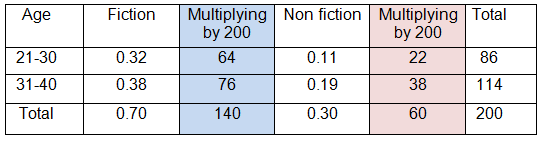
So, option D is correct.

Problem 9 :

A college surveyed 3500 of its students to determine if the students preferred music, movies or sports. The results of the survey are shown in the relative frequency table below.

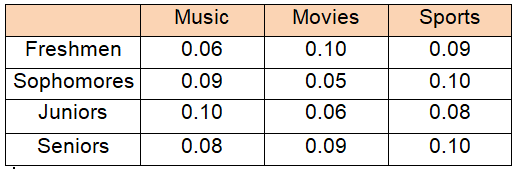
How many seniors and juniors were included in the survey ?

A)  70      B)  105       C)  140      D)  175

Solution :

To find number of Juniors, we have to multiply each quantity by 3500.

= 3500 (0.10 + 0.06 + 0.08)

= 3500(0.24)

= 840

To find number of Seniors, we have to multiply each quantity by 3500.

= 3500 (0.08 + 0.09 + 0.10)

= 3500(0.27)

= 945

Difference = 945 - 840

= 105

So, the answer is option B.

Problem 10 :

In the scatterplot shown below, which statement best describes the correlation between days of the week and the number of bicycles sold ?

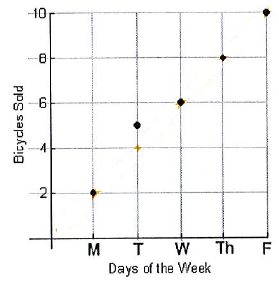
A)  High positive correlation       B)  Low negative correlation

C)  High negative correlation     D)  Low positive correlation

Solution :

Observing the scatterplot, it is clear the relation is high positive correlation.

Problem 11 :

The scatter plot below shows the number of arithmetic errors 10 students made on a quiz and the amount of time the students took to complete the quiz.

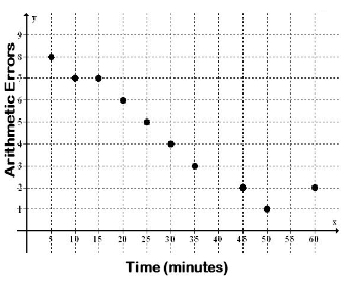
Which describes the relationship between the number of arithmetic errors the students made and the amount of the time the students took to complete the quiz ?

A)  There is a strong positive relationship between the variables

B)  There is a strong negative relationship between the variables.

C)  There is a weak positive relationship between the variables

D) There is a weak negative relationship between the variables.

Solution :

While drawing the line of best fit, we will get the falling line. So, there is strong negative correlation, option B.