MODELLING WITH LINEAR EQUATIONS WORD PROBLEMS

Problem 1 :

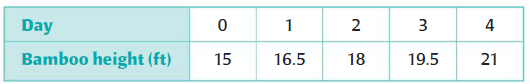
The table shows the height of a bamboo shoot during a period of fast growth. Use the table to write an equation modeling the growth. 

Solution :

When x = 0 and y = 15. So, y-intercept = 15.

Rate of change :

m = (y₂ - y₁)/(x₂ - x₁)

m = (16.5 - 15)/(1 - 0)

m = 1.5

Problem 2 :

Your cell phone plan costs $40 per month plus $0.10 per text message. You receive a bill for $53.80.

a) Writing a Model Write an equation for the situation. Solve it to find exactly how many text messages you sent.

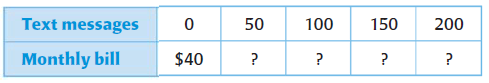
b) Making a Table Copy and complete the table below. Use the table to estimate how many text messages you sent.

Solution :

a) Let x be the number of text messages and y be the bill amount.

y = 40 + 0.10x ------(1)

Bill amount (y) = 53.80

53.80 = 40 + 0.10x

Subtract 40 on both sides.

53.80 - 40 = 0.10x

0.10x = 13.8

x = 13.8/0.10

x = 138

So, the number of text messages sent is 138.

b) Apply x = 50, x = 100, x = 15 and x = 200 in (1), we get

Problem 3 :

A paramotor is a parachute propelled by a fan-like motor. The table shows the height h of a Para motorist t minutes after beginning a descent. Find the height of the Para motorist after 7 minutes.

Solution :

The height decreases by 250 feet per minute.

Initial height = 2000

Let h be the height.

Rate of change(m) = -250

h = -250x + 2000

Height after 7 minutes.

When x = 7

h = -250(7) + 2000

h = -1750 + 2000

h = 250

So, height after 7 minutes is 250 feet.

Problem 4 :

A car used 14 gallons of gasoline and traveled a total distance of 550 miles. The car’s fuel efficiency is 40 miles per gallon on the highway and 35 miles per gallon in the city. How many gallons of gasoline were used on the highway?

Solution :

Quantity of gasoline used = 14

Distance covered = 550 miles

Fuel efficiency in highway = 40 miles

Let g be the number of gallons used in highway. Then, (14-g) is the quantity of fuel used in city.

Fuel efficiency in city = 35 miles

550 = 40g + 35(14-g)

Solving the equation, we get

550 = 40g + 490 - 35g

550 = 5g + 490

550 - 490 = 5g

g = 60/5

g = 12

So, the car used 12 gallons in highway.

Problem 5 :

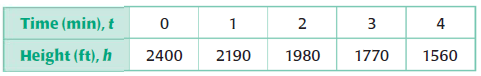
The table shows the height h of a para motorist after t minutes. Find the height of the para motorist after 8 minutes

Solution :

For every one minute, the height decreases.

Rate of change (m) = (2190 - 2400)/(1 - 0)

m = 210

y = 2400 - 210x

Height after 8 minutes

y = 2400 - 210(8)

y = 2400 - 1680

y = 720 ft