MATCH THE SYSTEM OF INEQUALITIES WITH ITS GRAPH
Problem 1 :
Match the graph with the correct system of inequalities.
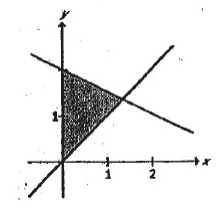
|
A) x + 2y ≤ 4 x ≤ y x ≥ 0 |
B) x + 2y ≤ 4 y ≤ x y ≥ 0 |
|
C) x + 2y ≥ 4 y ≤ x y ≥ 0 |
D) x + 2y ≥ 4 x ≤ y y ≥ 0 |
E) None of these
Solution:
Let take the point (0.5, 1).
To check the given inequalities,
|
A) x + 2y ≤ 4 0.5 + 2(1) ≤ 4 2.5 ≤ 4 (True) x ≤ y 0.5 ≤ 1 (True) x ≥ 0 0.5 ≥ 0 (True) C) x + 2y ≥ 4 0.5 + 2(1) ≥ 4 2.5 ≥ 4 (False) y ≤ x 1 ≤ 0.5 (False) y ≥ 0 1 ≥ 0 (True) |
B) x + 2y ≤ 4 0.5 + 2(1) ≤ 4 2.5 ≤ 4 (True) y ≤ x 1 ≤ 0.5 (False) x ≥ 0 0.5 ≥ 0 (True) D) x + 2y ≥ 4 0.5 + 2(1) ≥ 4 2.5 ≥ 4 (False) x ≤ y 0.5 ≤ 1 (True) y ≥ 0 1 ≥ 0 (True) |
So, option (A) is correct.
Problem 2 :
Find the maximum of the objective function: Z = 9x + 6y given the following constraints:
x + y ≤ 3
x - y ≤ 1
2x + 5y ≤ 10
x ≥ 0
y ≥ 0
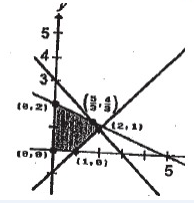
A) 9 B) 12 C) 24 D) 36 E) None of these
Solution:
To find the maximum value of the function Z = 9x + 6y
we have to apply the corner points, one by one in the function Z = 9x + 6y.
(0, 0) (0, 2) (1, 0) (2, 1)(5/3, 4/3)
|
Applying the point (0, 0) Z = 9x + 6y Z = 9(0) + 6(0) ==> 0 |
Applying the point (0, 2) Z = 9x + 6y Z = 9(0) + 6(2) ==> 12 |
|
Applying the point (1, 0) Z = 9x + 6y Z = 9(1) + 6(0) ==> 9 |
Applying the point (2, 1) Z = 9x + 6y Z = 9(2) + 6(1) ==> 24 |
Applying the point (5/3, 4/3)
Z = 9x + 6y
Z = 9(5/3) + 6(4/3) ==> 15+8 ==> 23
So, the maximum value is 24, option C.
Problem 3 :
Which inequality represents the graph to the right?
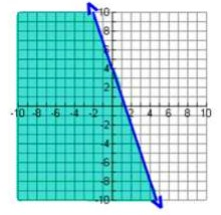
a) y ≥ 3x + 4 b) y ≤ -3x + 4 c) y > -3x + 4
d) y < 3x + 4
Solution:
It is easy to choose two point from the given picture.
(0, 4) and (1, 1)
Slope m = (1 - 4)/(1-0)
m = -3
Since it is falling line, it has negative slope. It is solid line, then we have to choose ≥ or ≤.
Let take the point (-2, 2).
To check given inequalities
a)
y ≥ 3x + 4
2 ≥ 3(-2) + 4
2 ≥ -6 + 4
2 ≥ -2 (False)
b)
y ≤ -3x + 4
2 ≤ -3(-2) + 4
2 ≤ 6 + 4
2 ≤ 10 (True)
So, option (b) is correct.
Problem 4 :
Which inequality represents the graph below?
a) y ≤ -3 b) y > -3 c) x > -3 d) x ≤ -3

Solution:
Let take the point (2, -4). Since it is vertical line, its equation will be x = a. accordingly the given options, checking with options a and b is useless.
To check given inequalities
c)
x > -3
2 > -3 (True)
d)
x ≤ -3
2 ≤ -3 (False)
So, option (C) is correct.
Problem 5 :
Match each system of equations to its graph below.
a) y < 8 x > -3
b) y < 5 y ≥ -7
c) y ≥ -3 x > 5
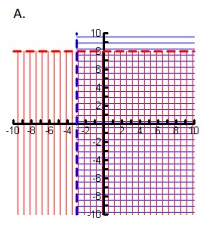
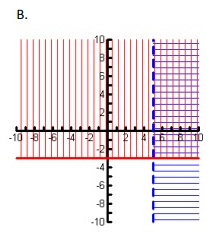
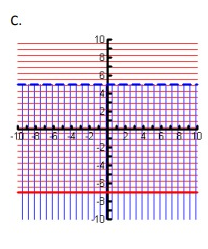
Solution:
a)

y < 8
x > -3
b)

y ≥ -3
x > 5
c)

y < 5
y ≥ -7
Problem 6 :
Which point is a solution to the system graphed to the right?
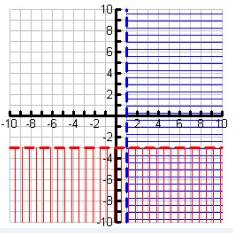
a) (4, 4) b) (4, -4) c) (-4, -4) d) (-4, 4)
Solution:
Point (4, -4) in the shaded area.
The solution is (4, -4).
So, option (b) is correct.
Problem 7 :
Which point(s) are solution to the system graphed to the right?
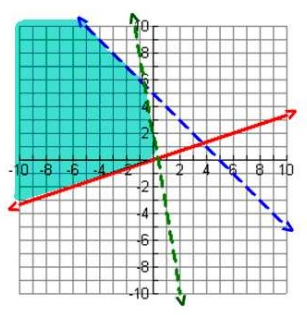
A) (-2, -3) B) (-1, 1) C) (2, 1) D) (-4, 0)
Solution:
Point (-2, -3) and (2, 1) are not in the shaded area. Points (-1, 1) and (-4, 0) are in the shaded area.
So, option (B) and (D) is correct.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling