MATCH EXPONENTIAL FUNCTIONS AND GRAPHS WORKSHEET

Match each function with its graph. 

Problem 1 :

f(x) = 2^x

Problem 2 :

f(x) = -2^x

Problem 3 :

f(x) = 4(2^x)

Problem 4 :

f(x) = (1/2)(2^x)

Problem 5 :

f(x) = (-1/2)(2^x)

Problem 6 :

f(x) = -4 (2^x)

Problem 1 :

f(x) = 2^x

Solution :

Let us find the y-intercept. So, we put x = 0

y = 2⁰

y = 1

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2, then it must be exponential growth function.

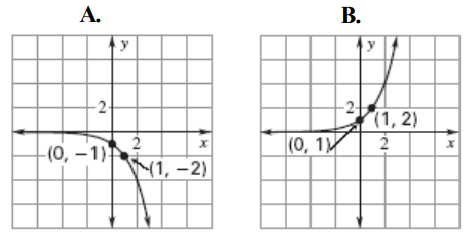
So, the y-intercept is (0, 1). In the given graphs, graph B is having the y-intercepts as (0, 1). Let us check one more point shown in the graph B.

When x = 1, y = 2¹ ==> 2

(1, 2)

So, graph B is correct.

Problem 2 :

f(x) = -2^x

Solution :

Since we have negative for a, it must be the reflection x-axis. Let us find the y-intercept. So, we put x = 0

y = -2⁰

y = -1

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2

y-intercept is (0, -1). In the given graphs, graph A is having the y-intercepts as (0, -1). Let us check one more point shown in the graph A.

When x = 1, y = -2¹ ==> -2

(1, -2). So, graph A is correct.

Problem 3 :

f(x) = 4(2^x)

Solution :

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2. Since the value of b is greater than 2, it must be exponential growth function. Let us find the y-intercept. So, we put x = 0

y = 4(2⁰)

y = 4(1)

y = 4

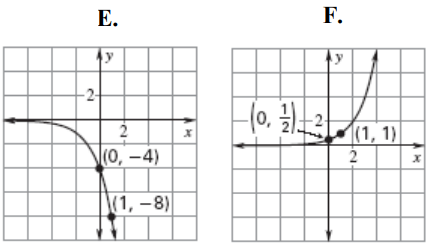
y-intercept is (0, 4). In the given graphs, graph D is having the y-intercepts as (0, 4). Let us check one more point shown in the graph D.

When x = 1, y = 4(2¹) ==> 8

(1, 8). So, graph D is correct.

Problem 4 :

f(x) = (1/2)(2^x)

Solution :

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2. Since the value of b is greater than 2, it must be exponential growth function. Let us find the y-intercept. So, we put x = 0

y = (1/2)(2^x)

y = (1/2)(2⁰)

y = 1/2

y-intercept is (0, 1/2). In the given graphs, graph F is having the y-intercepts as (0, 1/2). Let us check one more point shown in the graph F.

When x = 1, y = (1/2)(2¹) ==> 1

(1, 1). So, graph F is correct.

Problem 5 :

f(x) = (-1/2)(2^x)

Solution :

Since we have negative for a, it must be the reflection on x-axis. Let us find the y-intercept. So, we put x = 0

y = (-1/2)(2^x)

y = (-1/2)(2⁰)

y = -1/2

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2. Since the value of b is greater than 2, it must be exponential growth function. But there is a reflection about x-axis.

y-intercept is (0, -1/2). In the given graphs, graph C is having the y-intercepts as (0, -1/2). Let us check one more point shown in the graph C.

When x = 1, y = (-1/2)(2¹) ==> 1

(1, -1). So, graph C is correct.

Problem 6 :

f(x) = -4 (2^x)

Solution :

Since we have negative for a, it must be the reflection on x-axis. Let us find the y-intercept. So, we put x = 0

y = (-4)(2^x)

y = -4(2⁰)

y = -4

Comparing the given equation with y = a b^{(x - h)} + k, we get b = 2. Since the value of b is greater than 2, it must be exponential growth function. But there is a reflection about x-axis.

y-intercept is (0, -4). In the given graphs, graph E is having the y-intercepts as (0, -4). Let us check one more point shown in the graph E.

When x = 1, y = (-4)(2¹) ==> -8

(1, -8). So, graph E is correct.