LENGTH OF THE TANGENT FROM AN EXTERNAL POINT TO A CIRCLE

Tangents from an external point are equal in length.

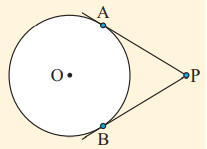
AP = PB

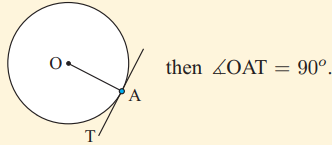
The tangent to a circle is perpendicular to the radius at the point of contact.

Problem 1 :

Find the value of x give reasons.

Solution :

A is a external point, the length of tangent lines drawn from the external point of the circle will be equal.

AD = AB

19 = x + 4

x = 19 - 4

x = 15

Problem 2 :

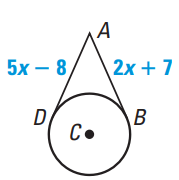
Solution :

A is a external point, the length of tangent lines drawn from the external point of the circle will be equal.

AD = AB

5x - 8 = 2x + 7

5x - 2x = 7 + 8

3x = 15

x = 15/3

x = 5

Problem 3 :

In the diagram given below, EF and EG are tangents of a circle with center C. Find the value of x.

Solution :

E is a external point, the length of tangent lines drawn from the external point of the circle will be equal.

EF = EG

2x + 3 = 4x - 5

2x - 4x = -5 - 3

-2x = -8

x = 4

Problem 4 :

Find the length of the tangent drawn from a point 8 cm away from the center of a circle of radius 6cm is

(a) √7 cm     (b) 2√7 cm      (c) 10 cm     (d) 5 cm

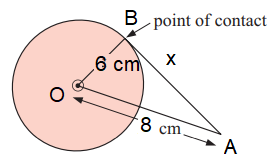
Solution :

∠ABO = 90 degree (angle between radius and tangent)

OA² = OB² + AB²

8² = 6² + x²

64 - 36 = x²

x² = 28

x = 2√7 cm

So, the length of tangent is 2√7 cm.

Problem 5 :

In the picture given below SR is the tangent of the circle with center P. Find the value of r.

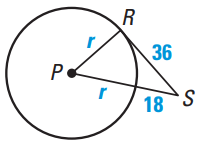
Solution :

From the given picture above,

PR = r, SR = 36, PS = r + 18

∠SRP = 90 degree

PS² = PR² + RS²

(r + 18)² = r² + 36²

r² + 324 + 36r = r² + 1296

36r = 1296 - 324

36r = 972

r = 972/36

r = 27

Problem 6 :

From a point P, the length of the tangent to a circle is 15 cm & distance of P from the centre of the circle is 17 cm. What is the radius of the circle ?

Solution :

Let x be the radius of the circle.

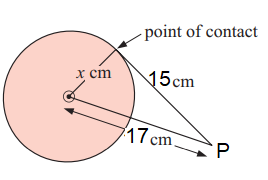
17² = x² + 15²

289 = x² + 225

x² = 289 - 225

x² = 64

x = 8

So, the radius of the circle is 8 cm.

Problem 7 :

In the figure given below, find the radius.

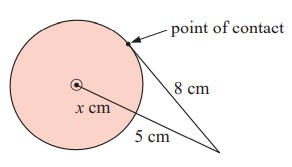
Solution :

Let x be the radius of the circle.

Angle between radius and tangent line will be right angle.

(x + 5)² = x² + 8²

x² + 10x + 25 = x² + 8²

10x + 25 = 64

10x = 64 - 25

10x = 39

x = 39/10

x = 3.9

Problem 8 :

In fig. ABC is a right ∆ right angled at B such that BC = 6 cm & AB = 8 cm .Find radius of the incircle.

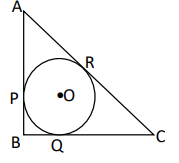
Solution :

Using Pythagorean theorem :

AC² = AB² + BC²

AC² = 6² + 8²

AC² = 36 + 64

AC² = 100

AC = 10

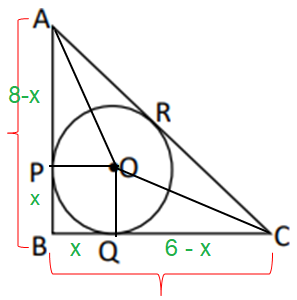
AP = AR and CQ = CR 

Because these are tangents drawn from the external point of the circle.

AC = 10

AR + RC = 10

8 - x + 6 - x = 10

14 - 2x = 10

2x = 14 - 10

2x = 4

x = 2 = OP = OQ

So, radius of the circle is 2 cm.

Problem 9 :

In the figure given below, find the length of the tangent XY.

Solution :

CX and XY are perpendicular.

Using Pythagorean theorem :

CY² = CX² + XY²

(5+8)² = 5² + XY²

13² = 5² + XY²

169 - 25 = XY²

XY² = 144

XY = 12

Problem 10 :

In given figure a circle is inscribed in a∆ PQR with PQ = 10 cm, QR = 8 cm and PR = 12cm. Find the length of QM,RN & PL

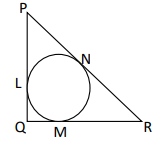
Solution :

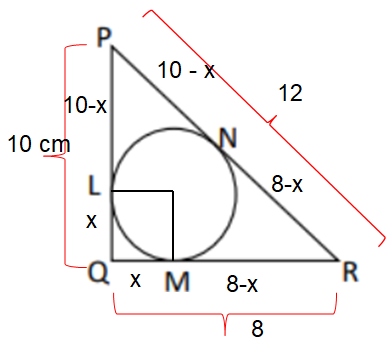
PR = 12

PN + NR = 12

10 - x + 8 - x = 12

18 - 2x = 12

2x = 6

x = 3

QM = 3 cm

RN = 8 - 3 ==> 5 cm

PL = 10 - 3 ==> 7 cm