LATERAL SURFACE AREA AND TOTAL SURFACE AREA OF TRIANGULAR PRISM

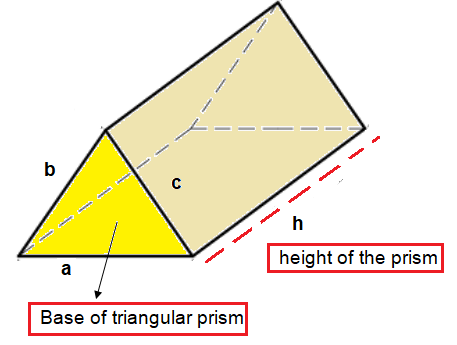
Lateral surface area = ph

p = Perimeter of the triangle and h = height of the prism

Total surface area = ph + 2B

B = Base area of triangular prism

Problem 1 :

Find the lateral surface area and total surface area of the triangular prism.

Solution :

Lateral surface area = p × h

P - Perimeter of base

= 12 + 13 + 5

= 30

Lateral surface area = 30 × 10

= 300 yd² --- > (1)

Total surface area = ph + 2B

Area of triangle = 1/2 × b × h

= 2 × 1/2 × 12 × 5

= 60 yd² --- > (2)

Add (1) & (2)

= 300 + 60

Total surface area   = 360 yd²

Problem 2 :

Find the lateral surface area and total surface area of the triangular prism.

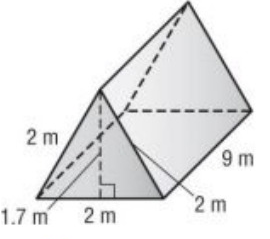
Solution :

Area of triangle = 2(1/2) × b × h

= 2(1/2) × 2 × 1.7

= 3.4 m² --- > (1)

Area of rectangle = 3 ×b × h

= 3 × 9 × 2

= 54 m² --- > (2)

Add (1) & (2)

Surface area = 3.4 + 54

= 57.4 m²

So, surface area of the triangular prism = 57.4 m²

Problem 3 :

Find the lateral surface area and total surface area of the triangular prism.

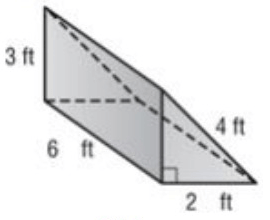
Solution :

Lateral surface area = p × h

P - Perimeter of base       

= 3 + 2 + 4

= 9

Lateral surface area = 9 × 6

= 54 ft² --- > (1)

Total surface area = ph + 2B

Area of triangle = 1/2 × b × h

= 2 × 1/2 × 2 × 3

= 6 ft² --- > (2)

Add (1) & (2)

= 54 + 6

Total surface area   = 60 ft²

Problem 4 :

Determine the surface area of the tent. The front of the tent has the shape on an isosceles triangle.

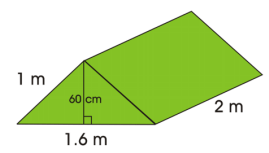
Solution :

Lateral surface area = p × h

P - Perimeter of base       

= 1.6 + 1 + 1

= 3.6

height of the prism = 2 m

Lateral surface area = 3.6 × 2

= 7.2 m²

Problem 5 :

This A-frame chalet needs to have the roof shingled. Determine the surface area of the roof.

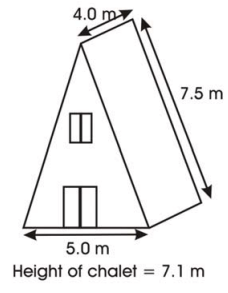
Hint: Think about whether the height of the chalet is the same as the height of the prism. Which measurements are unnecessary for this question?

Solution :

Surface area of roof = p × h

p = perimeter

= 5 + 7.5 + 7.5

= 20 m

Length from one end of the triangular face to another end is 4 m.

Surface area of roof = 20 x 4

= 80 square meter

Problem 6 :

Hector is designing a glass greenhouse for a city park. He has a 40-foot by 20- foot rectangular plot available. He wants the roof to be a triangular prism in which the center of the roof is 4 feet higher than the edges. The glass costs $25 per square foot, and Hector cannot spend more than $60,000 on glass.

a) What is the maximum height that Hector should make the edge of the roof?

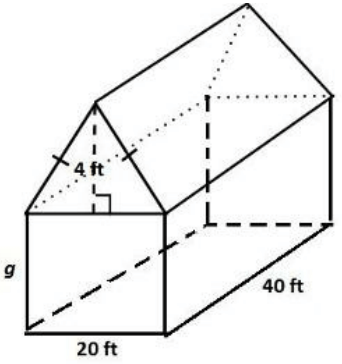
Solution :

In the triangular shape, the slant height :

l² = 4² + 10²

l² = 16 + 100

l = √116

l = 10.77

Find the sum of the surface areas of each individual section. 

The rectangular section of the front and back

= 2 × 20 × g or 40g ft²

The sides cover 

= 2 × 40 × g

= 80g ft²

The triangular tops of the front and back of the greenhouse cover

= 2(0.5)(4)(20) or 80 ft²

Thus, the roof covers 

= 2(40)(10.77) or 861 ft²

The total surface area is

= 861 + 80 + 120g ft²

Hector can use up to 60,000 ÷ 25 or 2400 ft²

941 + 120g = 2400

120g = 1459

g = 12.1

Therefore, g is approximately 12.1