ISOSCELES TRAPEZOID WITH MIDSEGMENT

Problem 1 :

Trapezoid with Midsegment

x = _____, y = _____

Solution :

By observing the figure,

Sum of co-interior angles = 180

(3y - 2)^º + 122^º = 180^º

3y^º - 2^º + 122^º = 180^º

3y^º + 120^º = 180^º

3y^º = 180^º - 120^º

3y^º = 60^º

y^º = 60^º/3^º

y^º = 20^º

So, the values of x and y is 4 and 20.

Problem 2 :

Isosceles Trapezoid with Midsegment

x = _____, y = _____

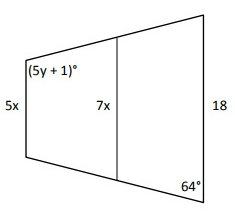
Solution :

By observing the figure,

Opposite angles are supplementary.

(5y + 1)^º + 64^º = 180^º

5y^º + 1^º + 64^º = 180^º

5y^º + 1^º = 180^º - 64^º

5y^º = 180^º - 64^º - 1^º

5y^º = 180^º - 65^º

5y^º = 115^º

y^º = 115^º/5^º

y^º = 23^º

So, the values of x and y is 2 and 23.

Problem 3 :

Trapezoid with Midsegment

x = _____, y = _____

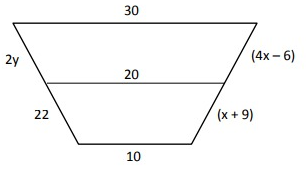
Solution :

By observing the figure,

Midsegment = 20

2y = 4x - 6 --- (1)

22 = x + 9 --- (2)

x = 22 - 9

x = 13

x = 13 substitute the equation (1).

2y = 4(13) - 6

2y = 52 - 6

2y = 46

y = 46/2

y = 23

So, the values of x and y is 13 and 23.

Problem 4 :

Trapezoid with Midsegment

x = _____, Perimeter = _____

Solution :

By observing the figure,

Midsegment = 2x + 4

 = 2(5) + 4

= 10 + 4

= 14

So, the value of x is 5.

Perimeter = 14 units.

Problem 5 :

Isosceles Trapezoid with Midsegment

x = _____, y = _____

Solution :

By observing the figure,

mid - segment theorem,

Opposite angles are supplementary.

72^º + (5y - 2)^º = 180^º

72^º + 5y^º - 2^º = 180^º

70^º + 5y^º = 180^º

5y^º = 180^º - 70^º

5y^º = 110^º

y^º = 110^º/5^º

y^º = 22^º

So, the values of x and y is 13.75 and 22.

Problem 6 :

Isosceles Trapezoid with Midsegment

x = _____, y = _____

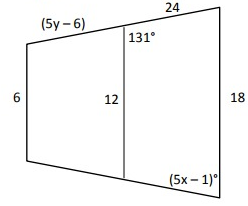
Solution :

5y - 6 = 24

5y = 24 + 6

5y = 30

y = 30/6

y = 5

Non - parallel sides will be equal.

5x - 1 = 24

5x = 24 + 1

5x = 25

x = 25/5

x = 5

So, the values of x and y is 5 and 5.