Learn Math step-by-step
INTEGRATING ABSOLUTE VALUE FUNCTIONS
Problem 1 :
Solution :
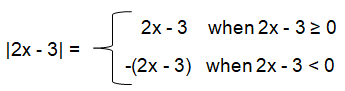
Vertex of the absolute value function is (3/2, 0)
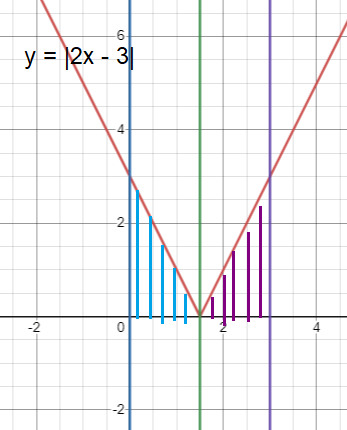
Area cannot be negative. So, the required area is 9/2 square units.
Problem 2 :
Solution :
x2 - 4x + 3
Decomposing into linear factors, we get
(x - 3)(x - 1)
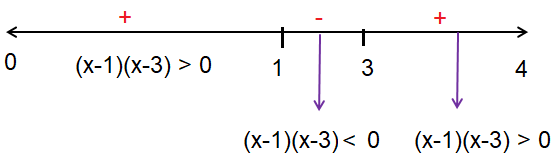
By drawing the sign diagram, we can easily figure out which part will positive and negative.
So, the required area is 4 square units.
Problem 3 :
Solution :
x3 - 5x2 + 6x
Decomposing into linear factors, we get
= x(x2 - 5x + 6)
= x (x - 2)(x - 3)
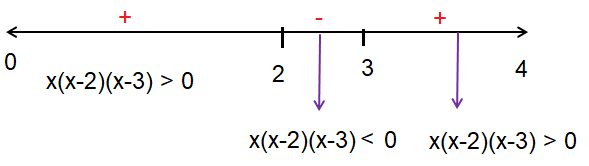
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling