IF THE GRAPH REPRESENTS ODD EVEN OR NEITHER WITHOUT EQUATION

A Function can be classified as Even, Odd or Neither. This classification can be determined graphically or algebraically.

How to check if the graph is odd ?

The graph will be symmetric with respect to the origin.

In other words :

If you spin the picture upside down about the Origin, the graph looks the same!

How to check if the graph is even ?

The graph will be symmetric with respect to the y-axis.

Decide if these are even or odd or neither from the graph.

Problem 1 :

Solution :

The graph is symmetric about origin. So, it is odd function.

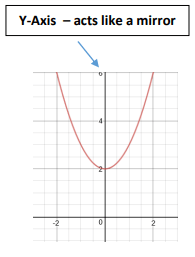
Problem 2 :

Solution :

Here y-axis is acting as a mirror. Clearly it is symmetric about y-axis. Then, it is even function.

Problem 3 :

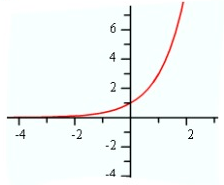
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

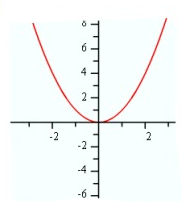
Problem 4 :

Solution :

Here y-axis is acting as a mirror. Clearly it is symmetric about y-axis. Then, it is even function.

Problem 5 :

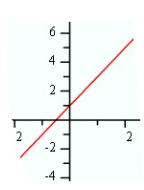
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

Problem 6 :

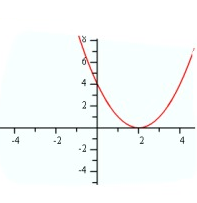
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

Problem 7 :

Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

Problem 8 :

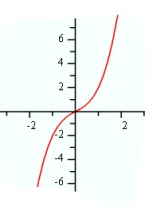
Solution :

The graph is symmetric about origin. So, it is odd function.