HOW TO WRITE A CUBIC FUNCTION FROM A GRAPH

To find cubic equation from the given zeroes, x-intercepts, solutions or values of x, we use the formula given below.

y = k(x - a) (x - b) (x - c)

Here a, b and c are x-intercepts.

Difference between touches and crosses :

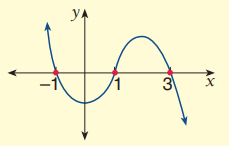
Here the curve crosses the x-axis at three different points.

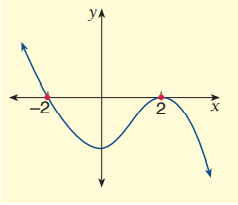
The curve crosses x-axis at -2 and touches the x-axis at 2. So, we have to take the factor (x - 2) twice. So, the equation would be y = (x + 2)(x - 2)²

Find the equation of the cubic with graph :

Problem 1 :

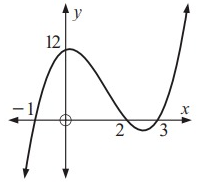
Solution :

By observing the figure.

Given the points -1, 2 and 3 

x = -1, x = 2 and x = 3

P(x) = a (x + 1) (x - 2) (x - 3) --- (1)

Since, the graph passes through the points are (0, 12).

The points (0, 12) substitute the equation (1).

12 = a (0 + 1) (0 - 2) (0 - 3)

12 = a (1) (-2) (-3)

12 = 6a

Dividing 6 on each sides.

2 = a

a = 2 substitute the equation (1).

P(x) = 2 (x + 1) (x - 2) (x - 3)

Problem 2 :

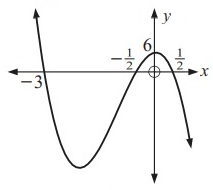
Solution :

By observing the figure.

Given the points -3, -1/2 and 1/2 

x = -3, x = -1/2 and x = 1/2

P(x) = a (x + 3) (x + 1/2) (x - 1/2)

= a (x + 3) 1/2[(2x + 1) (2x - 1)]--- (1)

Since, the graph passes through the points are (0, 6).

The points (0, 6) substitute the equation (1).

6 = a (0 + 3) 1/2[(2(0) + 1) (2(0) - 1)

6 = a (3) (1/2) (1) (-1)

6 = -3a/2

(6) (-2/3) = a

-12/3 =  a

-4 = a

a = -4 substitute the equation (1).

P(x) = -4 (x + 3) 1/2[(2x + 1) (2x - 1)]

= -2 (x + 3) (2x + 1) (2x - 1)

Problem 3 :

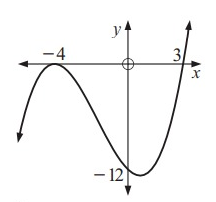
Solution :

By observing the figure, the curve passes through 3 and touches at -4

P(x) = a (x + 4)² (x - 3) --- (1)

Since, the graph touches the points are (0, -12).

The points (0, -12) substitute the equation (1).

-12 = a (0 + 4)² (0 - 3)

-12 = a (16) (-3)

-12 = -48a

Dividing -48 on each sides.

1/4 = a

a = 1/4 substitute the equation (1).

P(x) = 1/4 (x + 4)² (x - 3)

Problem 4 :

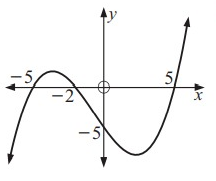
Solution :

By observing the figure.

Given the points -5, -2 and 5 

x = -5, x = -2 and x = 5

P(x) = a (x + 5) (x + 2) (x - 5) --- (1)

Since, the graph passes through the points are (0, -5).

The points (0, -5) substitute the equation (1).

-5 = a (0 + 5) (0 + 2) (0 - 5)

-5 = a (5) (2) (-5)

-5 = -50a

Dividing -50 on each sides.

1/10 = a

a = 1/10 substitute the equation (1).

P(x) = 1/10 (x + 5) (x + 2) (x - 5)

Problem 5 :

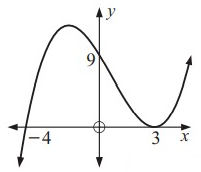
Solution :

By observing the figure, the curve crosses the x-axis at -4 and touches 3. So, we repeat the factor (x - 3) twice.

P(x) = a (x + 4) (x - 3)² --- (1)

Since, the graph touches the points are (0, 9).

The points (0, 9) substitute the equation (1).

9 = a (0 + 4) (0 - 3)²

9 = a (4) (-3)²

9 = 36a

Dividing 36 on each sides.

9/36 = a

1/4 = a

a = 1/4 substitute the equation (1).

P(x) = 1/4 (x + 4) (x - 3)²

Problem 6 :

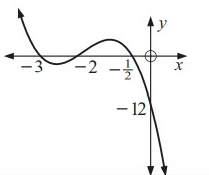
Solution :

By observing the figure.

x = -3, x = -2 and x = -1/2

P(x) = a (x + 3) (x + 2) (x + 1/2)

= a (x + 3) (x + 2) 1/2(2x + 1)--- (1)

Since, the graph passes through the points are (0, -12).

The points (0, -12) substitute the equation (1).

-12 = a (0 + 3) (0 + 2) 1/2(2(0) + 1)

-12 = a(3) (2) 1/2

-12 = 6a/2

-12 = 3a

-12/3 =  a

-4 = a

a = -4 substitute the equation (1)

P(x) = -4 (x + 3) (x + 2) 1/2(2x + 1) 

= -2 (x + 3) (x + 2 ) (2x + 1)