HOW TO GRAPH STEP FUNCTION

Problem 1 :

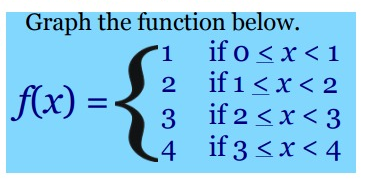
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point 1, 2, 3 and 4 respectively. 

For y = 1, the domain is [0, 1). At the left end use filled circle and at right end use unfilled circle.

For y = 2, the domain is [1, 2). At the left end use filled circle and at right end use unfilled circle.

For y = 3, the domain is [2, 3). At the left end use filled circle and at right end use unfilled circle.

For y = 4, the domain is [3, 4). At the left end use filled circle and at right end use unfilled circle.

Problem 2 :

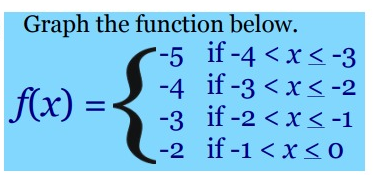
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point -5, -4, -3 and -2 respectively. 

For y = -5, the domain is (-4, -3]. At the left end use unfilled circle and at right end use filled circle.

For y = -4, the domain is (-3, -2]. At the left end use unfilled circle and at right end use filled circle.

For y = -3, the domain is (-2, -1]. At the left end use unfilled circle and at right end use filled circle.

For y = -2, the domain is (-1, 0]. At the left end use unfilled circle and at right end use filled circle.

Problem 3 :

Graph the step function.

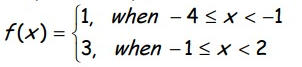
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point 1 and 3 respectively. 

For y = 1, the domain is [-4, -1). At the left end use filled circle and at right end use unfilled circle.

For y = 3, the domain is [-1, 2). At the left end use filled circle and at right end use unfilled circle.

Problem 4 :

A step function is defined using the piecewise formula 

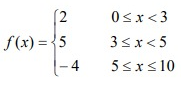
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point 2, 5 and -4 respectively. 

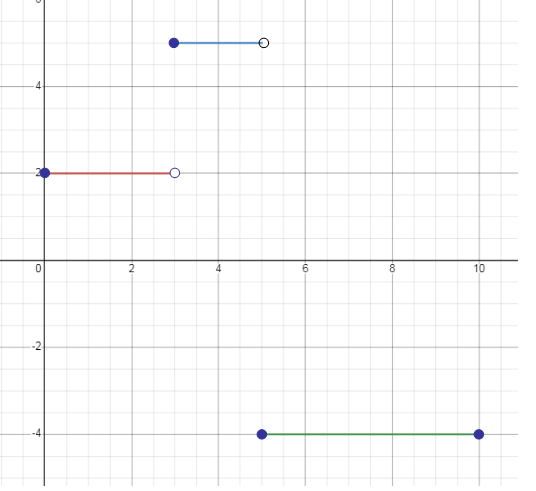
For y = 2, the domain is [0, 3). At the left end use filled circle and at right end use unfilled circle.

For y = 5, the domain is [3, 5). At the left end use filled circle and at right end use unfilled circle.

For y = -4, the domain is [5, 10]. At both ends, we should use the filled circle.

Problem 5 :

Graph the step function. Describe the domain and range.

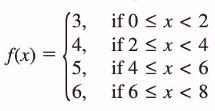
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point 3, 4, 5 and 6 respectively. 

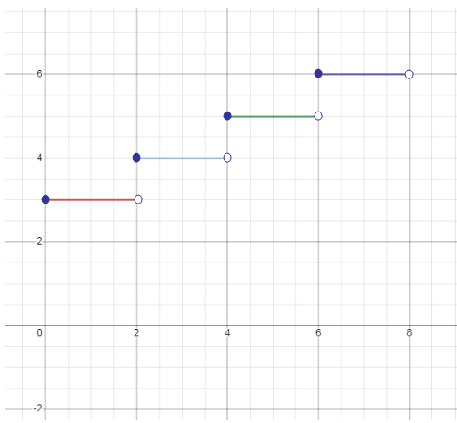
Domain:

For y = 3, the domain is [0, 2). At the left end use filled circle and at right end use unfilled circle.

For y = 4, the domain is [2, 4). At the left end use filled circle and at right end use unfilled circle.

For y = 5, the domain is [4, 6). At the left end use filled circle and at right end use unfilled circle.

For y = 6, the domain is [6, 8). At the left end use filled circle and at right end use unfilled circle.

Range:

Range = {3 ≤ y ≤ 7}

Problem 6 :

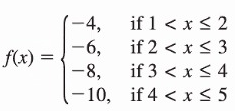
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point -4, -6, -8 and -10 respectively. 

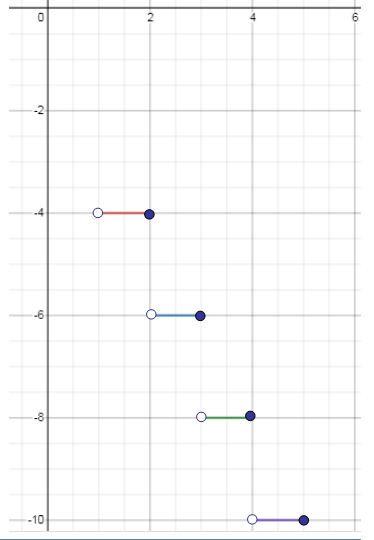
Domain:

For y = -4, the domain is (1, 2]. At the left end use unfilled circle and at right end use filled circle.

For y = -6, the domain is (2, 3]. At the left end use unfilled circle and at right end use filled circle.

For y = -8, the domain is (3, 4]. At the left end use unfilled circle and at right end use filled circle.

For y = -10, the domain is (4, 5]. At the left end use unfilled circle and at right end use filled circle.

Range:

Range = {-4 ≤ y ≤ -10}

Problem 7 :

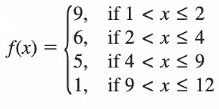
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point 9, 6, 5 and 1 respectively. 

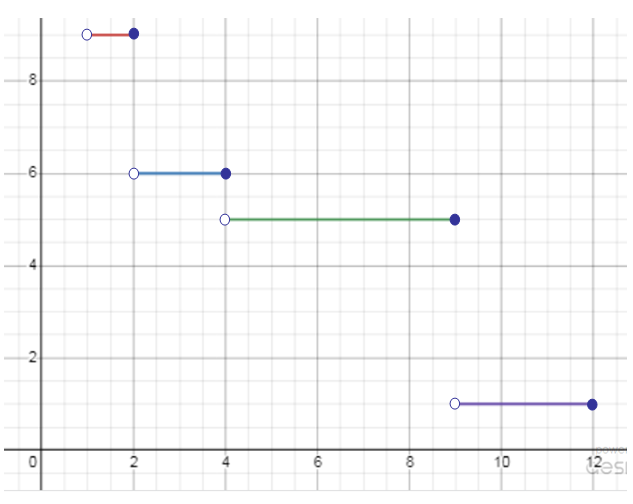
Domain:

For y = 9, the domain is (1, 2]. At the left end use unfilled circle and at right end use filled circle.

For y = 6, the domain is (2, 4]. At the left end use unfilled circle and at right end use filled circle.

For y = 5, the domain is (4, 9]. At the left end use unfilled circle and at right end use filled circle.

For y = 1, the domain is (9, 12]. At the left end use unfilled circle and at right end use filled circle.

Range:

Range = {9 ≤ y ≤ 1}

Problem 8 :

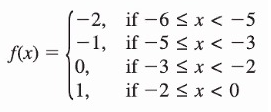
Solution:

f(x) = y

So, the line that we draw should be horizontal line. It will pass through the point -2, -1, 0 and 1 respectively. 

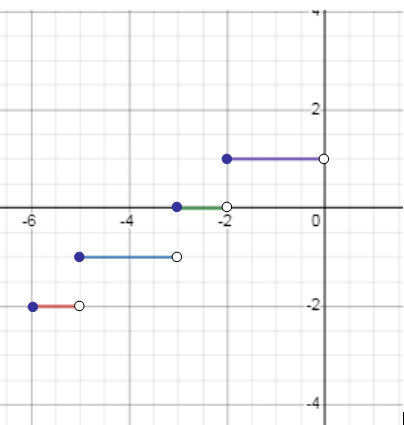
Domain:

For y = -2, the domain is [-6, -5). At the left end use filled circle and at right end use unfilled circle.

For y = -1, the domain is [-5, -3). At the left end use filled circle and at right end use unfilled circle.

For y = 0, the domain is [-3, -2). At the left end use filled circle and at right end use unfilled circle.

For y = 1, the domain is [-2, 0). At the left end use filled circle and at right end use unfilled circle.

Range:

Range = {-2 ≤ y ≤ 1}