HOW TO FIND THE EQUATION OF A TANGENT LINE WITH TRIG FUNCTIONS

To find equations of the tangle line for the given function, we have to follow the steps given below.

Step 1 :

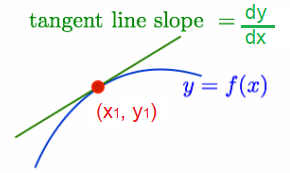
Find the slope of the tangent line drawn to the curve at the indicated point. For that, we have to find the derivative of the equation of the curve.

Derivative of equation of the curve = Slope of the tangent

To find slope at the specific point, apply the given point in the slope that we have derived.

Step 2 :

The point will be given, use that as (x₁, y₁)

Step 3 :

Using the formula 

y - y₁ = m(x - x₁)

we can find equation of the tangent line.

Find the equation of the tangent line to the following curves at the point indicated.

Problem 1 :

y = x cos x at (0, 0)

Solution :

y = x cos x

Here we have two differentiable functions, so we use product rule to find the derivative.

d(uv) = uv' + vu'

u = x and v = cos x

u' = 1 and v' = -sinx

Applying the values in the formula, we get

dy/dx = x (-sin x) + cos x (1)

Slope (dy/dx) = -x sin x + cos x

Slope at (0, 0) :

dy/dx = -(0)  sin (0) + cos (0)

dy/dx =  1

That is, slope of the tangent line at (0, 0) is 1.

Equation of tangent :

y - y₁ = m(x - x₁)

y - 0 = 1(x - 0)

y = x

So, equation of the required tangent line is y = x.

Problem 2:

y = sin x tan x at (π/6, 1/2√3)

Solution:

y = sin x tan x

Here we have two differentiable functions, so we use product rule to find the derivative.

d(uv) = uv' + vu'

u = sin x and v = tan x

u' = cos x and v' = sec² x

Applying the values in the formula, we get

Slope (dy/dx) = (sin x) (sec² x) + (tan x) (cos x)

Slope at (π/6, 1/2√3):

dy/dx = sin(π/6) sec²(π/6) + tan(π/6) cos(π/6)

= (1/2) (2/√3)² + (1/√3) (√3/2)

= 2/3 + 1/2

dy/dx = 7/6

That is, slope of the tangent line at (π/6, 1/2√3) is 7/6.

Equation of tangent:

y - y₁ = m(x - x₁)

y - 1/2√3 = 7/6 (x - π/6)

y - 1/2√3 = 7x/6 - 7π/36

y = 7x/6 + 1/2√3 - 7π/36

So, equation of the required tangent line is y = 7x/6 + 1/2√3 - 7π/36.

Problem 3:

y = sec x at (π/4, √2)

Solution:

y = sec x

Slope (dy/dx) = sec(x) tan(x)

Slope at (π/4, √2):

dy/dx = sec(π/4) tan(π/4)

= (√2) (1)

dy/dx = √2

That is, slope of the tangent line at (π/4, √2) is √2.

Equation of tangent:

y - y₁ = m(x - x₁)

y - √2 = √2 (x - π/4)

y - √2 = √2x - √2π/4

y = √2x + √2 - √2π/4

So, equation of the required tangent line is y = √2x + √2 - √2π/4.

Problem 4 :

y = sin x at (0, 0)

Solution :

y = sin x

Slope (dy/dx) = cos x

Slope at (0, 0):

dy/dx = cos (0)

dy/dx = 1

That is, slope of the tangent line at (0, 0) is 1.

Equation of tangent:

y - y₁ = m(x - x₁)

y - 0 = 1 (x - 0)

y = x

So, equation of the required tangent line is y = x.

Problem 5 :    

y = x + tan x at (0, 0)

Solution :

y = x + tan x

Slope (dy/dx) = 1 + sec²x

Slope at (0, 0):

dy/dx = 1 + sec² (0)

= 1 + (1)²

dy/dx = 2

That is, slope of the tangent line at (0, 0) is 2.

Equation of tangent:

y - y1 = m(x - x1)

y - 0 = 2(x - 0)

y = 2x

So, equation of the required tangent line is y = 2x.

Problem 6 :

y = csc x at (π/3, 2/√3)

Solution :

y = csc x

Slope (dy/dx) = - cot(x) csc(x)

Slope at (π/3, 2/√3):

dy/dx = - cot (π/3) csc(π/3)

= - (1/√3) (2/√3)

dy/dx = -2/3

That is, slope of the tangent line at (π/3, 2/√3) is -2/3.

Equation of tangent:

y - y₁ = m(x - x₁)

y - 2/√3 = -2/3 (x - π/3)

y - 2/√3 = -2x/3 + 2π/9

y = -2x/3 + 2/√3 + 2π/9

So, equation of the required tangent line is y = -2x/3 + 2/√3 + 2π/9.