HOW TO FIND THE DOMAIN AND RANGE F A RELATION GRAPH

Problem 1 :

Identify the values of the domain for the following relation :

A) {-2, 0, 1, 3}        B) {-3, -2, 0, 1, 2}

C) {-2, -1, 0, 1, 2, 3}     D) {-3, 0, 1, 2} 

Solution :

From the given graph,

A (2, 1), B (-3, -2), C (0, 3) and D (1, 0)

The domain of a relation is the set of x values of the ordered pairs.

Domain = {2, -3, 0, 1}

Domain = {-3, 0, 1, 2}

So, option (D) is correct.

Problem 2 :

Identify the values for the domain of the function :

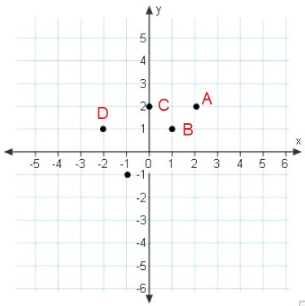
A)  {-2, -1, 0, 1, 2}

B) {(1, -2), (-1, -1), (2, 0), (1, 1), (2, 2)}

C) {-1, 1, 2}

D) {(-2, 1), (-1, -1), (0, 2), (1, 1), (2, 2)}

Solution :

From the given graph,

A (2, 2), B (1, 1), C (0, 2), D (-2, 1) and (-1, -1)

The domain of a relation is the set of x values of the ordered pairs.

Domain = {2, 1, 0, -2, -1}

Domain = {-2, -1, 0, 1, 2}

So, option (A) is correct.

Problem 3 :

List the range for the following function.

{(2, 3), (4, 5), (3, 4), (7, 8)}

A) {3, 4, 5, 8}           B) {2, 3, 4, 7}

C) {2, 3, 4, 5, 7, 8}       D) {2, 3, 3, 4, 4, 5, 7, 8}

Solution :

The range of a relation is the set of y values of the ordered pairs.

Range = {3, 5, 4, 8}

Range = {3, 4, 5, 8}

So, option (A) is correct.

Problem 4 :

Identify the values of the range for the following relation :

{(2, 3), (5, 7), (7, 9), (4, 8)}

A) {2, 5, 7, 4}             B) {2, 3, 4, 8}

C) {2, 8}                     D) {3, 7, 9, 8}

Solution :

The range of a relation is the set of y values of the ordered pairs.

Range = {3, 7, 9, 8}

So, option (D) is correct.

Problem 5 :

Identify the range for the following relation :

A) {-2, -1, 3, 5}                    B) {-1, 0, 4, 7}

C) {-2, -1, 0, 3, 4, 5, 7}           D) {0, 1, 4, 7}

Solution :

Range = {4, 0, -1, 7}

So, option (B) is correct.

Problem 6:

To graph the equation y = 3x - 7,

Renita created the following table of values.

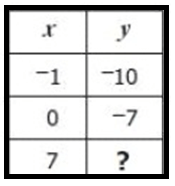
Which y -value corresponds to 7?

A)   21    B)  28      C) 14      D)  -4

Solution :

y = 3x - 7

Put x = 7,

y = 3(7) - 7

y = 21 - 7

y = 14

So, option (C) is correct.

Problem 7 :

A relation is graphed as shown.

Find all the values that are in the domain of this relation.

Solution :

From the given graph,

(1, 6), (3, 3), (4, 2), (6, 5)

Domain = {1, 3, 4, 6}