HOW TO FIND DIAGONAL OF CYLINDER

Problem 1 :

A cylindrical can of seltzer has a height of 5 inches and a radius of 1 inch, as shown.

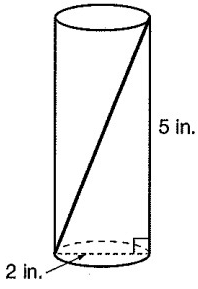
Solution :

h = 5 in, r = 2 in.

Diagonal of cylinder c² = a² + b²

c² = 5² + 2²

= 25 + 4

= 29

c = √29

So, diagonal of the cylinder √29in.

Problem 2 :

Find the length of the diagonal of shown below.

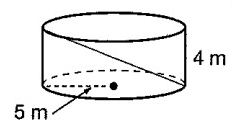
Solution :

h = 4 m and r = 5 m

Diagonal of cylinder c² = a² + b²

c² = 4² + (10)²

= 16 + 100

= 116

d = √116

d = 10.8

So, diagonal of the cylinder is 10.8.

Problem 3 :

Find the length of the diagonal of shown below.

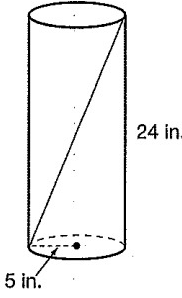
Solution :

By observing the figure,

Height h= 24 in.

Radius r = 5 in.

Diagonal of cylinder c² = a² + b²

d² = (10)² + (24)²

= 100 + 576

= 676

d = √676

d = 26

So, diagonal of the cylinder is 26.

Problem 4 :

An oil tank is in the shape of a cylinder. A dipstick can be used to measure the amount of oil in the tank.

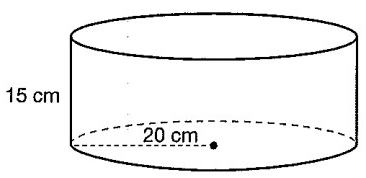
The dipstick has a length that is an integer value. What is the smallest possible length of a dipstick that cannot be submerged completely in the oil tank?

Solution :

Height h= 15 cm

Radius r = 20 cm

Diagonal of cylinder c² = a² + b²

c² = (40)² + (15)²

= 1600 + 225

= 1825

d = √1825

d = 42.72

diagonal of the cylinder is 42.72

So, length of dipstick 43 cm.