HOW TO FIND AVERAGE VALUE OF FUNCTION ON GIVEN INTERVAL

Let f(x) be a function on the interval [a,b]. If we divided our interval into n equally sized intervals and took the sample at left endpoints f(x_i), then an approximation for the average value would be given by the formula:

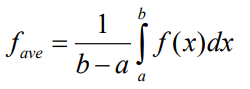
Find the average value of the function over the given interval

Problem 1 :

f(x) = -x² + 2x + 1 for [1, 4]

Solution :

a = 1, b = 4, then b - a = 4 - 1 ==> 3

So, the average value of the given function in the given interval is -1.

Problem 2 :

Find the average value of the function on the interval. At what point(s) in the interval does the function assume its average value?

Solution :

a = 0, b = 3, b - a = 3 - 0 ==> 3

Applying the values in the formula, we get

Average value of the given function in the given interval is -3/2. 

Let the required value be c.

-c²/2 = -3/2

c² = 3

c = √3

c = ±√3

-√3 is not in the given interval. So, the required value is √3

Problem 3 :

f(x) = 3e^x on [-1, 0]

Solution :

a = 1, b = 0

b - a ==> 0 - 1 ==> -1

Problem 4 :

Solution :

Here a = -3, b = -2, then

b - a = -2 - (-3) ==> -2 + 3 ==> 1

Problem 5 :

Solution :

So, the average value of the function is 1.27

Problem 6 :

f(x) = 2 sin x , [-π/3, π/4]

Solution :

So, the average value of the function is -0.22.