HORIZONTAL AND VERTICAL DILATION WITH EQUATION

Dilations are enlargements or reductions.

The transformation equation for a dilation with center (0, 0) and factor k are x' = kx and y' = ky

• If k > 1, the image figure is an enlargement of the object
• If 0 < k < 1, the image figure is an reduction of the object.

Vertical dilation with fixed y-axis :

Suppose P(x, y) moves P'(x', y') such that P' lies on the line through N(0, y) and P, and

we call this a horizontal dilation with the factor k.

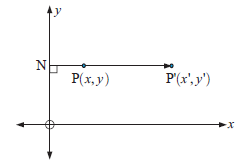
For a horizontal dilation with factor k, the transformation equation are 

x' = kx and y' = y

Vertical dilation with fixed x-axis :

Suppose P(x, y) moves P'(x', y') such that P' lies on the line through N(x, 0) and P, and

we call this a vertical dilation with the factor k.

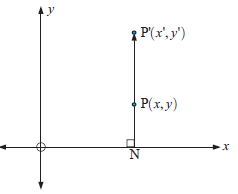
For a vertical dilation with factor k, the transformation equation are 

x' = x and y' = ky

Problem 1 :

Find the image equation of 

y = 2x + 3

under a dilation with center O and factor 2.

Solution :

dilation factor = 2, so k = 2

Dilation with center O.

Given function is y = 2x + 3

x' = 2x and y' = 2y

x = x'/2 and y = y'/2

By applying the above values in the equation, we get

y'/2 = 2(x'/2) +3

y'/2 = x' + 3

Changing x' and y' as x and y respectively.

y/2 = x + 3

y = 2(x + 3)

y = 2x + 6

Problem 2 :

Find the image equation of 

y = -x²

under a dilation with center O and factor 1/2.

Solution :

dilation factor = 1/2, so k = 1/2

Dilation with center O.

x' = x/2 and y' = y/2

x = 2x' and y = 2y'

2y' = -(2x')²

2y' = -4x'²

y' = -2x'²

Changing x' and y' as x and y respectively.

y = -2x²

Problem 3 :

Find the image equation of 

y = 2x²

under a horizontal dilation with the factor 4.

Solution :

dilation factor = 4, so k = 4

Horizontal dilation, x values only will affect

x' = 4x and y' = y

x = x'/4

y' = 2(x'/4)²

y' = 2(x'²/16)

y' = (x'²/8)

Changing x' and y' as x and y respectively.

y = (x²/8)

Problem 4 :

Find the image equation of 

xy = 2

under a horizontal dilation with the factor 2.

Solution :

dilation factor = 2, so k = 2

Horizontal dilation, x values only will affect

x' = 2x and y' = y

x = x'/2

(x'/2) y' = 2

x'y' = 4

Changing x' and y' as x and y respectively

xy = 4

Problem 5 :

Find the image equation of 

y = 2^x

under a vertical dilation with the factor 2.

Solution :

dilation factor = 2, so k = 2

vertical dilation, y values only will affect

x' = x and y' = 2y

y = y'/2

(y'/2) = 2^x

y' = 2^x' (2)

y' = 2^x'+1

Changing x' and y' as x and y respectively

y = 2^x+1

Problem 6 :

Find the image equation of 

y = 3x + 2

under a dilation with center O and factor 3.

Solution :

dilation factor = 3, so k = 3

Dilation with center O.

Given function is y = 3x + 2

x' = 3x and y' = 3y

x = x'/3 and y = y'/3

By applying the above values in the equation, we get

y'/3 = 3(x'/3) + 2

y'/3 = x' + 2

y' = 3(x' + 2)

y' = 3x' + 6

Changing x' and y' as x and y respectively.

y = 3x + 6

Problem 7 :

Find the image equation of 

2x - 5y = 10

under the vertical dilation with the factor 2.

Solution :

dilation factor = 2, so k = 2

Dilation with center O.

Given function is 2x - 5y = 10, vertical dilation y only will affect.

x' = x and y' = 2y

x = x' and y = y'/2

By applying the above values in the equation, we get

2x' - 5(y'/2) = 10

4x' - 5y' = 20

Changing x' and y' as x and y respectively,

4x - 5y = 20

Problem 8 :

Find the image equation of 

y = -2x + 1

under the horizontal translation with the factor 1/2.

Solution :

dilation factor = 1/2, so k = 1/2

Given function is y = -2x + 1, horizontal dilation x only will affect.

x' = x/2 and y' = y

x = 2x' and y = y'

By applying the above values in the equation, we get

y' = -2(2x') + 1

y' = -4x' + 1

Changing x' and y' as x and y respectively, we get

y = -4x + 1