GRAPHING QUADRATIC FUNCTION IN VERTEX FORM

Graph the following function.

Problem 1 :

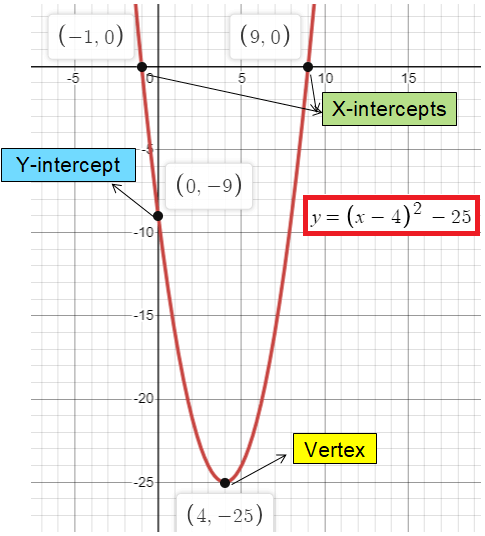
y = (x - 4)² - 25

Solution :

Finding vertex :

y = (x - 4)² - 25

By comparing the given equation with vertex form

y = a(x - h)² + k

we get (h, k) ==> (4, -25)

The vertex is at (4, -25).

Here a = 1 > 0, then the parabola opens up. It will have minimum.

Finding x-intercepts :

0 = (x - 4)² - 25

(x - 4)² = 25

x - 4 = √25

x - 4 = ±5

x-intercepts are (-1, 0) and (9, 0).

Finding y-intercept :

Put x = 0

y = (0 - 4)² - 25

y = 16 - 25

y = -9

y-intercept is (0, -9)

Problem 2 :

y = (-1/2)(x + 3)² + 8

Solution :

Finding vertex :

y = (-1/2)(x + 3)² + 8

By comparing the given equation with vertex form

y = a(x - h)² + k

we get (h, k) ==> (-3, 8)

The vertex is at (-3, 8).

Here a = -1/2 < 0, then the parabola opens down. It will have maximum.

Finding x-intercepts :

0 = (-1/2)(x + 3)² + 8

(-1/2)(x + 3)² + 8 = 0

(-1/2)(x + 3)² = -8

Multiplying by 2 on both sides, we get

(x + 3)² = 16

x + 3 = √16

x + 3 = ±4

x-intercepts are (1, 0) and (-7, 0).

Finding y-intercept :

Put x = 0

y = (-1/2)(0 + 3)² + 8

y = -9/2 + 8

y = (-9+16)/2

y = 7/2

y-intercept is (0, 7/2)

Problem 3 :

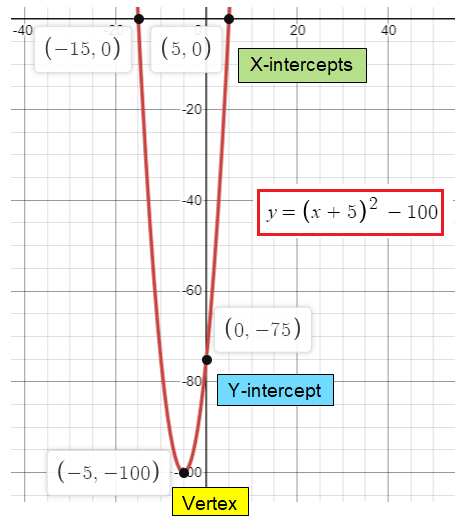
y = (x + 5)² - 100

Solution :

Finding vertex :

y = (x + 5)² - 100

By comparing the given equation with vertex form

y = a(x - h)² + k

we get (h, k) ==> (-5, -100)

The vertex is at (-5, -100).

Here a = 1 > 0, then the parabola opens up. It will have minimum.

Finding x-intercepts :

0 = (x + 5)² - 100

(x + 5)² - 100 = 0.

(x + 5)² = 100

x + 5 = √100

x + 5 = ±10

x-intercepts are (5, 0) and (-15, 0).

Finding y-intercept :

Put x = 0

y =  (0 + 5)² - 100

y = 25 - 100

y = -75

y-intercept is (0, -75)